CBSE > Class 09 > Mathematics | CBSE Board | Homework Help | myCBSEguide

No products in the cart.

Ask questions which are clear, concise and easy to understand.

Ask Question

Simplify by rationalising the denominator. 2underrot6-5underrot / divided 3 underrot 5-2underrot6

Posted by Ashwani Kumar 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Sia ? 5 years, 8 months ago

We have,
$\frac{2 \sqrt{6}-\sqrt{5}}{3 \sqrt{5}-2 \sqrt{6}}$ = $\frac{2 \sqrt{6}-\sqrt{5}}{3 \sqrt{5}-2 \sqrt{6}} \times \frac{3 \sqrt{5}+2 \sqrt{6}}{3 \sqrt{5}+2 \sqrt{6}}$
= $\frac{(2 \sqrt{6}-\sqrt{5})(3 \sqrt{5}+2 \sqrt{6})}{(3 \sqrt{5}-2 \sqrt{6})(3 \sqrt{5}+2 \sqrt{6})}$
= $\frac{2 \sqrt{6}(3 \sqrt{5}+2 \sqrt{6})-\sqrt{5}(3 \sqrt{5}+2 \sqrt{6})}{(3 \sqrt{5})^{2}-(2 \sqrt{6})^{2}}$
= $\frac{6 \sqrt{30}+24-15-2 \sqrt{30}}{45-24}$
= $\frac{4 \sqrt{30}+9}{21}$
$\therefore$ $\frac{2 \sqrt{6}-\sqrt{5}}{3 \sqrt{5}-2 \sqrt{6}}$ = $\frac{4 \sqrt{30}+9}{21}$

ANSWER

2x-3x =180

Posted by Vishal Kumar 5 years, 8 months ago

CBSE > Class 09 > Mathematics

2 answers

Chaitanya Hadpe 5 years, 8 months ago

-x=180 x=-180

Sunny Kumar 5 years, 8 months ago

-x=180 -1x=180 -X=180/1 X=180 Ans

ANSWER

2.2

Posted by Arun Yadav 5 years, 8 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

NCERT EXEMPLER exercise 1.3 question number 14 (v,vi,vii)

Posted by @Dinesh_ 5N04 5 years, 8 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Y + B+ O + R = ? ( Y,B,O,R colours)

Posted by Prathamesh Kevale 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Karishma Saini 5 years, 8 months ago

Yellow blue orange red

ANSWER

a2-b2=(a+b)(a-b)

Posted by Riya Kumari 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Sunny Kumar 5 years, 8 months ago

Ya a2-b2=(a+b) (a-b)

ANSWER

a2+b2+2ab

Posted by Riya Kumari 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Sunny Kumar 5 years, 8 months ago

(a+b)2

ANSWER

2 x = 304142 (12 30-100 40)45

Posted by Rakesh Kumar 5 years, 8 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

a+b+c=5 and ab+bc+ac=10, then orove that a^3+b^3+c^3-3abc=-25..

Posted by Neha Krishnan 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Sia ? 5 years, 8 months ago

As we know,

$a ^ { 3 } + b ^ { 3 } + c ^ { 3 } - 3 a b c =$ $( a + b + c ) \left( a ^ { 2 } + b ^ { 2 } + c ^ { 2 } - a b - b c - c a \right)$

$= ( a + b + c ) \left[ a ^ { 2 } + b ^ { 2 } + c ^ { 2 } - ( a b + b c + c a ) \right]$

$= 5 \left\{ a ^ { 2 } + b ^ { 2 } + c ^ { 2 } - ( a b + b c + c a ) \right\}$

$= 5 \left( a ^ { 2 } + b ^ { 2 } + c ^ { 2 } - 10 \right)$

Now, $a + b + c = 5$

Squaring both sides, we get

$( a + b + c ) ^ { 2 } = 5 ^ { 2 }$

$\Rightarrow a ^ { 2 } + b ^ { 2 } + c ^ { 2 } + 2 ( a b + b c + c a ) = 25$

$\therefore a ^ { 2 } + b ^ { 2 } + c ^ { 2 } + 2 ( 10 ) = 25$

$\Rightarrow a ^ { 2 } + b ^ { 2 } + c ^ { 2 } = 25 - 20 = 5$

Now, $a ^ {3} + b ^ {3} + c ^ { 3 } - 3 a b c = 5 \left( a ^ { 2 } + b ^ { 2 } + c ^ { 2 } - 10 \right)$

$= 5 ( 5 - 10 ) = 5 ( - 5 ) = - 25$

Hence, proved.

ANSWER

The sum of rational number is always rational? Please comment the answer...

Posted by Tanushka Rawat 5 years, 8 months ago

CBSE > Class 09 > Mathematics

4 answers

Sunny Kumar 5 years, 8 months ago

Yes 2+2=4 it will always be a rational no

Nikita Virmani 5 years, 8 months ago

Yes The sum of rational is always rational

Ayan Ali 5 years, 8 months ago

Yes always be rational no.

M M 5 years, 8 months ago

yes

ANSWER

If a+b+c=9 and a²+b² +c²=35,find the value of a³+b³+c³-3abc

Posted by Pradum Dubey 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Ishika Darpe 5 years, 8 months ago

a^3+b^3+c^3-3abc = (a+b+c)(a^2+b^2+c^2-ab-bc-ca)

ANSWER

Ex 13.7

Posted by Dayaram Yadav 5 years, 8 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Different identities

Posted by Green Tea 5 years, 8 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

A= Set of all even positive integers less than 9

Posted by Keimah Thingtlang Naupangfela 5 years, 8 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Examine wheather √2 is rational or irrational number

Posted by Harsh Patel 5 years, 8 months ago

CBSE > Class 09 > Mathematics

2 answers

Sunny Kumar 5 years, 8 months ago

Root 2 is an irrational

Srishtika Murugan 5 years, 8 months ago

Root 2 is an irrational no.

ANSWER

4root 3/2-root 2-30/4root 3-3root 2-3root 2/3+2root 3

Posted by Rima Tiwary 5 years, 8 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Find a rational number between -2 by 3and 1by 4 ,

Posted by Sunil Harjai 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Sia ? 5 years, 8 months ago

The rational number $\left(\frac{-2}{3}+\frac{1}{4}\right)$ $\div$ 2 lies in the middle of the two given rational numbers, that is, $\frac{-2}{3}$ and $\frac{1}{4}$ .
Now, $\left(\frac{-2}{3}+\frac{1}{4}\right)$ = $\frac{(-2) \times 4 + 3 \times 1}{12}$ = $\frac{-8+3}{12}$ = $\frac{-5}{12}$
$\therefore$ $\left(\frac{-2}{3}+\frac{1}{4}\right)$ $\div$ 2 = $\frac{-5}{12}$ $\div$ 2 = $\frac{-5}{12}$ $\times$ $\frac{1}{2}$ = $\frac{-5}{24}$
Thus, the required rational number is $\frac{-5}{24}$ . That is, $\frac{-2}{3}$ < $\frac{-5}{24}$ < $\frac{1}{4}$ .

ANSWER

5×x=16. So find the value of 5×x÷2+1

Posted by Sagar Gupta 5 years, 8 months ago

CBSE > Class 09 > Mathematics

2 answers

Railway Hub 5 years, 8 months ago

9

Sagar Gupta 5 years, 8 months ago

20

ANSWER

√x+1/x find value , if x = 7+√40

Posted by Lata Singh 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Dipanshu Verma 5 years, 8 months ago

hiii

ANSWER

4(1/2)

Posted by Bhoomi Mishra 5 years, 8 months ago

CBSE > Class 09 > Mathematics

2 answers

Aditi Mundhe 5 years, 8 months ago

4 x 1/2 4/2 =2

Upllakshaya Jain 5 years, 8 months ago

2

ANSWER

cube root of 2 × fourth root of 3

Posted by Sri Hari 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Ilayapriya2114@Mail.Com Yuvibala 5 years, 8 months ago

568

ANSWER

Is the sss and rjs criteria of congruency

Posted by Navneet Ghuman 5 years, 8 months ago

CBSE > Class 09 > Mathematics

2 answers

Ayan Ali 5 years, 8 months ago

Yes

Aditi Mundhe 5 years, 8 months ago

SSS and RJS are none of the criterias of congruency.

ANSWER

Which identity will be use to factories y2-xby 100 2

Posted by Vrushabh Tubachi 5 years, 8 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

6x +19x +15

Posted by Banny Sundrapalli 5 years, 8 months ago

CBSE > Class 09 > Mathematics

3 answers

Sunny Kumar 5 years, 8 months ago

6x+15x-6x+15 by(middle term split) 3x(2x-5x)-3(2x-5x) (3x-3) (2x-5x) I ? think the question is wrong because the right Question is 6x2square +9x +15

Narendra Kumar 5 years, 8 months ago

6x+19x+15 6x+9x+10x+15 3x(2x+3)+5(2x+3) (2x+3)(3x+5)

Ruchika Aggarwal 5 years, 8 months ago

6x^2+19x+15 6x^2+9x+10x+15 3x(2x+3)+5(2x+3) (3x+5)(2x+3)

ANSWER

Define axioms and postulates

Posted by Mahi Singh 5 years, 8 months ago

CBSE > Class 09 > Mathematics

2 answers

Chirag Sharma 5 years, 8 months ago

He used the term 'POSTULATE' for the assumption that geomatry. AXIOMS are also true statement in which they are appiabale for only mathematics are called axioms.

Manjunatha Setty K A 5 years, 8 months ago

Axioms are true statements in which they are applicable for only mathematics are called axioms Postulates are also true statements in which they are applicable for only geometry

ANSWER

Expand form of (3a+4b)3

Posted by Ashmit Gardia 5 years, 8 months ago

CBSE > Class 09 > Mathematics

3 answers

Sarthak Shavarn 5 years, 8 months ago

Abe nikal yaha se

Mahi Singh 5 years, 8 months ago

(3a)(3a)(3a) +(4b)(4b)(4b) +3(3a)(4b)(3a+4b) 27a³+64b³+108a²b+144ab²

Yogita Ingle 5 years, 8 months ago

Using the identity (a + b)³ = a³ + b³+ 3a²b + 3ab² , we have , we have
= (3a)³ + (4b)³ + 3 (3a)²(4b) + 3(3a) (4b)²
= 27a³ + 64b³+ 108a²b + 144ab²

which is the required expanded form.

ANSWER

A cylindrical tub container a radius 12cm to a depth 20cm . A spherical iron ball is drop into the tub and thus the level of water is rased by 6.75cm . What is the radius of the ball?

Posted by Sumit Karan 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Sia ? 5 years, 8 months ago

According to question it is given that
Radius of cylindrical tub = 12 cm
Depth of cylindrical tub = 20 cm
Let us suppose that (r) be the radius of spherical ball
Again it is given that level of water is raised by 6.75 cm
Now, according to the question,
Volume of spherical ball = Volume of water rise in cylindrical tub
$\Rightarrow \quad \frac { 4 } { 3 } \pi r ^ { 3 } = \pi ( 12 ) ^ { 2 } \times 6.75$
$\Rightarrow \frac { 4 } { 3 } r ^ { 2 } = 12 \times 12 \times 6. 75$
$\Rightarrow \quad r ^ { 3 } = \frac { 12 \times 12 \times 6.75 \times 3 } { 4 }$
$\Rightarrow \quad r ^ { 3 } = 729$
$\Rightarrow \quad r = \sqrt [ 3 ] { 729 } = 9 \mathrm { cm }$
Therefore, Radius of the ball = 9 cm

ANSWER

x3+3x3+3x+1÷x+

Posted by Yash Sihag 5 years, 8 months ago

CBSE > Class 09 > Mathematics

1 answers

Vrushabh Tubachi 5 years, 8 months ago

Using identity solve (x-3)(x+3)

ANSWER

An exterior angle of a triangle is 115°& one of the interior opposite angle is 35°. Find the other two angles.

Posted by Hariom Dalal 5 years, 8 months ago

CBSE > Class 09 > Mathematics

2 answers

Sia ? 5 years, 8 months ago

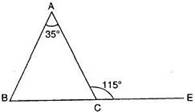
Let in $\bigtriangleup$ ABC, exterior $\angle$ ACE = 115^o and $\angle$ A = 35^o
We know that,
$\angle$ ACE = $\angle$ A + $\angle$ B (Exterior Angle Theorem)
$\Rightarrow$ 115^o = 35^o + $\angle$ B
$\angle$ B = 115^o – 35^o = 80^o
Again, in $\bigtriangleup$ ABC,
$\angle$ A + $\angle$ B + $\angle$ C = 180^o
(The sum of the three angles of a triangle is 180^o)
$\Rightarrow$ 35^o + 80^o + $\angle$ C = 180^o
$\Rightarrow$ 115^o + $\angle$ C = 180^o
$\Rightarrow$ $\angle$ C = 180^o – 115^o = 65^o

Srishtika Murugan 5 years, 8 months ago

In the figure, By linear pair axiom, Angle ACB = 180 - 115 =65 By angle sum property, Angle ABC=180-(AngleBAC +AngleACB)=180-(35+65)=180-100=80

ANSWER

Cos10+cos20+....+cos170=

Posted by Balamourougane Balamourougane 5 years, 8 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Page 377 of 1013

myCBSEguide App

myCBSEguide

Trusted by 1 Crore+ Students

Test Generator

Test Generator

Create papers online. It's FREE.

CUET Mock Tests

CUET Mock Tests

75,000+ questions to practice only on myCBSEguide app

Google Play

Download myCBSEguide App