Ask questions which are clear, concise and easy to understand.
Ask QuestionPosted by Noty Kannika 5 years, 8 months ago
- 1 answers
Posted by Aryan Agnihotri 5 years, 8 months ago
- 2 answers
Simran Kaur 5 years, 8 months ago
Posted by Ankit Patel 5 years, 8 months ago
- 1 answers
Shubh Agarwal 5 years, 8 months ago
Posted by Niraj Mishra 5 years, 8 months ago
- 1 answers
Shubh Agarwal 5 years, 8 months ago
Posted by Niraj Mishra 5 years, 8 months ago
- 0 answers
Posted by Soham Guru 5 years, 8 months ago
- 0 answers
Posted by Athul Santhosh 5 years, 8 months ago
- 4 answers
Posted by Loki Sushmi 5 years, 8 months ago
- 0 answers
Posted by Aryan Tiwari 5 years, 8 months ago
- 1 answers
Yogita Ingle 5 years, 8 months ago
Rational number: In mathematics, a rational number is a number which can be expressed as a fraction or a quotient, i.e., in the form of p/q where p and q are the two integers and ‘p’ is the numerator and ‘q’ is the non-zero denominator and p/q is in the lowest form, i.e. p and q have no common factors.
Irrational numbers: Irrational numbers are those which can’t be expressed in fractional form, i.e., in p/q form. They neither terminate nor do they repeat. They are also known as non- terminating non-repeating numbers.
Posted by Subarna Rai 5 years, 8 months ago
- 1 answers
Gaurav Seth 5 years, 8 months ago
Consider a square ABCD whose diagonals AC and BD intersect each other at a point O.
We have to prove that AC = BD, OA = OC, OB = OD, and ∠AOB = 90º.
Consider ΔABC and ΔDCB,
AB = DC
∠ABC = ∠DCB = 90°
BC = CB (Common side)
∴ ΔABC ≅ ΔDCB (By SAS congruency)
∴ AC = DB (By CPCT)
Hence, the diagonals of the square ABCD are equal in length.
Now, consider ΔAOB and ΔCOD,
∠AOB = ∠COD (Vertically opposite angles)
∠ABO = ∠CDO (Alternate interior angles)
AB = CD (Sides of a square are equal)
∴ ΔAOB ≅ ΔCOD (By AAS congruence rule)
∴ AO = CO and OB = OD (By CPCT)
Hence, the diagonals of the square bisect each other.
Similarly, in ΔAOB and ΔCOB,
AO = CO
AB = CB
BO = BO
∴ ΔAOB ≅ ΔCOB (By SSS congruency)
∴ ∠AOB = ∠COB (By CPCT)
∠AOB + ∠COB = 180º (Linear pair)
or, 2∠AOB = 180º
or, ∠AOB = 90º
Hence, the diagonals of a square bisect each other at right angles.
Posted by Kjode Sharma 5 years, 8 months ago
- 3 answers
Arvind Yadav 5 years, 8 months ago
Posted by Sanjana Singh 5 years, 8 months ago
- 4 answers
Abhishek Kumar 5 years, 8 months ago
Upllakshaya Jain 5 years, 8 months ago
Posted by Sumit Lohar 5 years, 8 months ago
- 1 answers
Prisha Gupta 5 years, 8 months ago
Posted by Muskan Praveen 5 years, 9 months ago
- 1 answers
Posted by Surya Sekhar Jena 5 years, 9 months ago
- 2 answers
Gaurav Seth 5 years, 9 months ago

Given:
In quadrilateral ABCD, AB smallest & CD is longest sides.
To Prove: ∠A>∠C
& ∠B>∠D
Construction: Join AC.
Mark the angles as shown in the figure..
Proof:
In △ABC , AB is the shortest side.
BC > AB
∠2>∠4 …(i)
[Angle opposite to longer side is greater]
In △ADC , CD is the longest side
CD > AD
∠1>∠3 …(ii)
[Angle opposite to longer side is greater]
Adding (i) and (ii), we have
∠2+∠1>∠4+∠3
⇒∠A>∠C
Similarly, by joining BD, we can prove that
∠B>∠D
Posted by Ayush Arora 5 years, 9 months ago
- 1 answers
Posted by Abhimanyu Sharma 5 years, 9 months ago
- 1 answers
Posted by Swapnil Shinde 5 years, 9 months ago
- 1 answers
Sia ? 5 years, 9 months ago
Proof: Let us prove this statement by contradiction method. Let us assume that the line segment PT has two midpoints R and S.

⇒PR=12PT...........(1)
PS=12PT .......... (2) (∵ R and S are mid-points according to assumption)
from (1) and (2) , we get
⇒ PR = PS
But this is possible only if R and S coincide. { which may not be possible if R and S are two different points }
Therefore,Our contradiction is incorrect.
i.e. there is only one mid-point of every line segment.
Hence, proved
Posted by Rafeeque Rafeeque 5 years, 9 months ago
- 1 answers
Sia ? 5 years, 9 months ago
Given: ΔABC with AB = AC
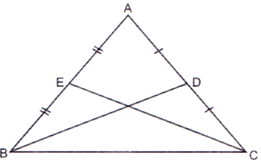
And AD = CD, AE = BE.
To prove: BD = CE
Proof: In ΔABC we have
AB = AC [Given]
⇒12AB=12AC
⇒ AE = AD
[∵ D is the mid-point of AC and E is the mid-point of AB]
Now, in ΔABD and ΔACE, we have
AB = AC [Given]
∠A=∠A (Common angle]
AE = AD [Proved above]
SO, by SAS criterion of congruence, we have
ΔABD≅ΔACE
⇒ BD = CE [CPCT]
Hence, proved.
Posted by Premananda Patra 5 years, 9 months ago
- 1 answers
Posted by Kaviya Sri 5 years, 9 months ago
- 0 answers
Posted by Nikhil Singh 5 years, 9 months ago
- 2 answers
Posted by Prakash Khadye 5 years, 9 months ago
- 3 answers
Posted by Aditya Vavhal 5 years, 9 months ago
- 2 answers
Mohd Raza 5 years, 9 months ago
Ayush Ramteke 5 years, 9 months ago
Posted by Aakansha Sharma 5 years, 9 months ago
- 2 answers
Posted by Amab Kumar 5 years, 9 months ago
- 0 answers
Posted by Aditya Raj 5 years, 9 months ago
- 0 answers

myCBSEguide
Trusted by 1 Crore+ Students

Test Generator
Create papers online. It's FREE.

CUET Mock Tests
75,000+ questions to practice only on myCBSEguide app
 myCBSEguide
myCBSEguide
A A 5 years, 8 months ago
1Thank You