Miscellaneous Exercise
1. Show that the sum of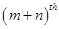 
and 
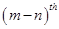terms of an A.P. is equal to twice the 
terms.
Ans. Here,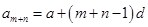 
…..(i)
and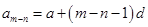 
…..(ii)
To prove: 
Adding eq. (i) and (ii), we get
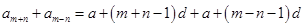
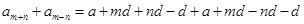
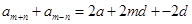
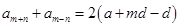
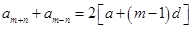
2. If the sum of three numbers in A.P., is 24 and their product is 440. Find the numbers.
Ans. Let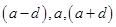 
be three numbers in A.P.
According to question,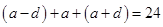 
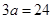
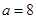
And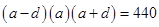 
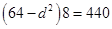
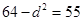
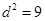
Taking 
, A.P. is (8 – 3), 8, (8 + 3)
5, 8, 11
Taking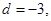 
A.P. is (8 + 3), 8, (8 – 3)
11, 8, 5
3. Let sum of 
terms of an A.P. be 
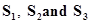respectively, show that
.
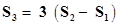Ans. Given: 
…..(i)
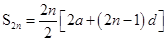…..(ii)
And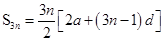 
Now,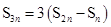 
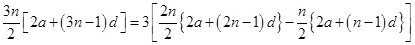
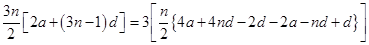
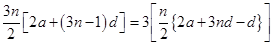
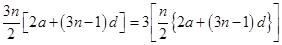
Proved.
4. Find the sum of all numbers between 200 and 400 which are divisible by 7.
Ans. Given: A.P. 203, 210, 217, ………., 399
Here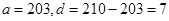 
and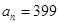 
Now,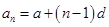 
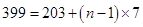
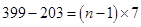
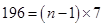
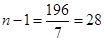
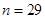
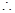
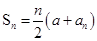
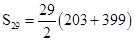
= 8729
5. Find the sum of integers from 1 to 100 that are divisible by 2 or 5.
Ans. Given: A.P. which is divisible by 2
2, 4, 6, ………., 100
Here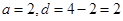 
and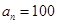 
Now,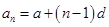
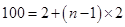
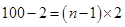
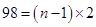
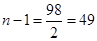
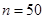
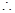
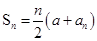
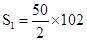= 2550
Again A.P. which is divisible by 5
5, 10, 15, ………., 100
Here 
and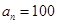
Now,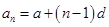
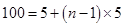
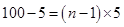
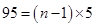
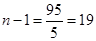
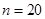
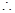
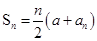
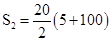
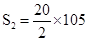= 1050
Again A.P. which is divisible by both 2 and 5
10, 20, 30, ………., 100
Here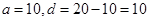 
and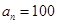
Now,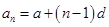
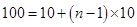
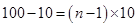
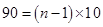
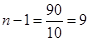
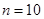
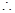
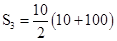
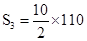= 550
Now, According to question,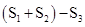 
= (2550 + 1050) – 550 = 3050
6. Find the sum of all two digit numbers which when divided by 4, yield 1 as remainder.
Ans. Given: A.P. 13, 17, 21, ………., 97
Here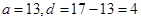 
and 
Now,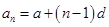
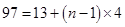
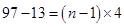
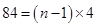
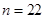
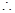
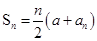
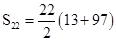
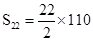= 1210
7. If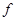 
is a function satisfying 
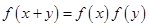for all 
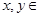N such that 
=3 and 
= 120, find the value of 
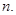
Ans. Here: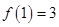 
and 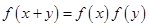for all 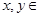N ……….(i)
Putting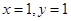 
in eq. (i),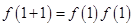 
= 9
Putting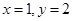 
in eq. (i),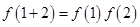 
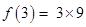= 27
Putting 
in eq. (i), 
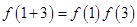
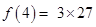= 81
Now, 
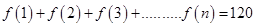
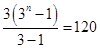
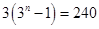
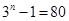
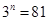
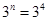
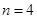
8. The sum of some terms of G.P. is 315 whose first term and the common ratio are 5 and 2 respectively. Find the last term and the number of terms.
Ans. Given: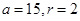 
and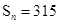 
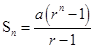
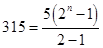
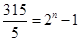
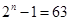
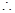
9. The first term of a G.P. is 1. The sum of the third term and fifth term is 90. Find common ratio of G.P.
Ans. Given: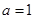 
and 
= 
= 
= 
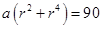
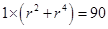
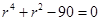
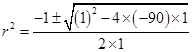
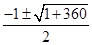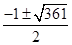
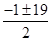
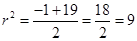or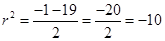 
which is not possible
Therefore, common ratio is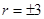 
10. The sum of three numbers in G.P. is 56. If we subtract 1, 7, 21 from these numbers in that order, we obtain an arithmetic progression. Find the numbers.
Ans. Let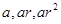 
be three numbers in G.P., therefore,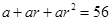 
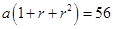..(i)
According to question,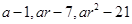 
are in A.P.
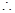
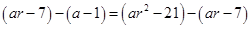
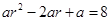
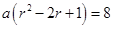…..(ii)
Dividing eq. (i) by eq. (ii),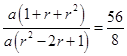 
= 
= 
= 
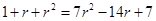
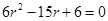
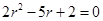
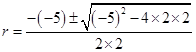
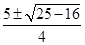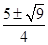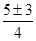
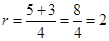or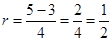 
Putting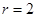 
in eq. (i),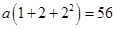 
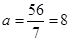
Then the required numbers are 8, 16, 32.
Putting 
in eq. (i),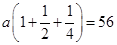 
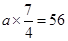
Then the required numbers are 32, 16, 8.
11. A G.P. consists of an even number of terms. If the sum of all the terms is 5 times the sum of terms occupying odd places, then find its common ratio.
Ans. Let the number of terms be 
, number of odd terms be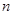 
and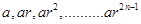 
be in G.P.
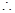
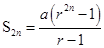and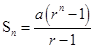
According to question,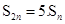 
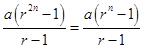
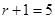
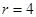
12. The sum of the first four terms of an A.P. is 56. The sum of the last four terms is 112. If its first term is 11, then find the number of terms.
Ans. Given: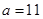 
and 
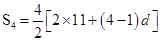
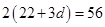
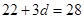
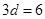
Also,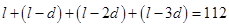 
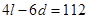
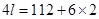
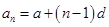
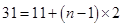
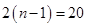
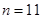
13. If 
then show that 
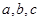and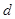 
are in G.P.
Ans. Taking 
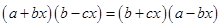
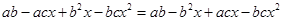
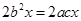
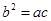
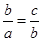……….(i)
Taking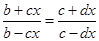 
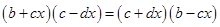
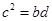
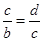……….(ii)
From eq. (i) and (ii),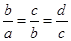 
14. Let S be the sum, P the product and R the sum of reciprocals of 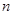terms in a G.P. Prove that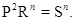 
Ans. Here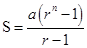 
, P = 
and R =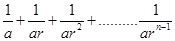 
Now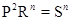 
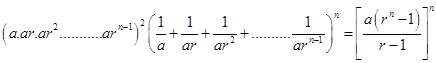
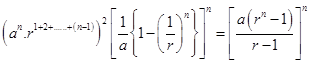
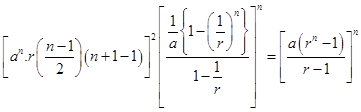
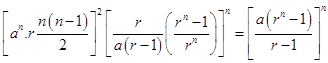
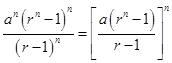
Proved.
15. The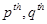 
and 
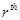terms of an A.P. are 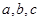respectively. Show that
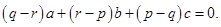
Ans. According to question,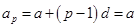 
, 
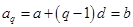and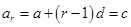 
Now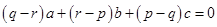 
Putting values of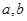 
and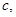 
we get
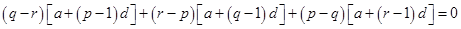
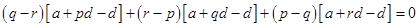
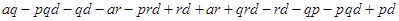
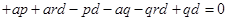
0 = 0 Proved.
16. If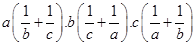 
are in A.P., prove that 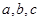are in A.P.
Ans. Given: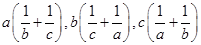 
are in A.P.
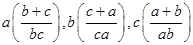are in A.P.
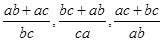are in A.P.
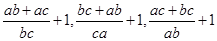are in A.P. [Adding 1 to each numerator]
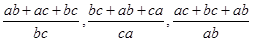are in A.P.
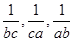are in A.P.[Dividing each fraction by 
]
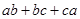are in A.P.[Multiplying each fraction by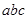 
]
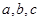are in A.P.
Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now