NCERT Solutions class 12 Maths Exercise 8.1 Class 12 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
Download NCERT solutions for Applications of Integrals as PDF.

NCERT Solutions class 12 Maths Applications of Integrals
1. Find the area of the region bounded by the curve 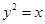 and the lines
and the lines 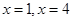 and the
and the 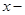 axis.
axis.
Ans. Equation of the curve (rightward parabola) is 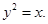

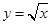 ……….(i)
……….(i)
 Required area (shaded region)
Required area (shaded region)
=  [From eq. (i)]
[From eq. (i)]
= 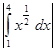 =
= 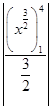 =
= 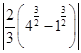
= 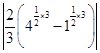 =
= 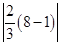 =
= 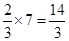 sq. units
sq. units
NCERT Solutions class 12 Maths Exercise 8.1
2. Find the area of the region bounded by 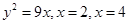 and the
and the 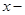 axis in the first quadrant.
axis in the first quadrant.
Ans. Equation of the curve (rightward parabola) is 
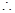
 ……….(i)
……….(i)
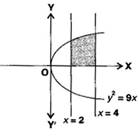
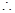 Required area (shaded region) bounded by curve
Required area (shaded region) bounded by curve 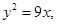 (vertical lines
(vertical lines 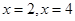 ) and
) and 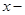 axis in first quadrant.
axis in first quadrant.
= 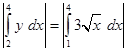 [From eq. (i)]
[From eq. (i)]
= 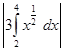 =
= 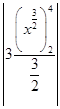
= 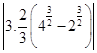 =
= 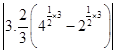
= 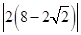 =
= 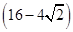 sq. units
sq. units
NCERT Solutions class 12 Maths Exercise 8.1
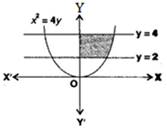
3. Find the area of the region bounded by 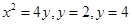 and the
and the  axis in the first quadrant.
axis in the first quadrant.
Ans. Equation of curve (parabola) is 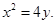
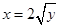 ……….(i)
……….(i)
 Required (shaded) area bounded by curve
Required (shaded) area bounded by curve 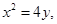 (Horizontal lines
(Horizontal lines  ) and
) and  axis in first quadrant.
axis in first quadrant.
= 
=  =
= 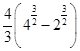 =
= 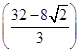 sq. units
sq. units
NCERT Solutions class 12 Maths Exercise 8.1
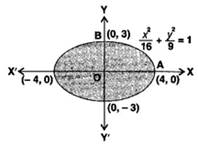
4. Find the area of the region bounded by the ellipse 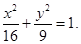
Ans. Equation of ellipse is 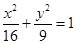 ……….(i)
……….(i)
Here 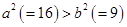
From eq. (i),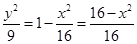



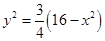 ……….(ii)
……….(ii)
for arc of ellipse in first quadrant.
Ellipse (i) ia symmetrical about 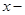 axis,
axis,
(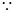 On changing
On changing 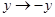 in eq. (i), it remains unchanged)
in eq. (i), it remains unchanged)
Ellipse (i) ia symmetrical about  axis,
axis,
(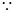 On changing
On changing 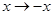 in eq. (i), it remains unchanged)
in eq. (i), it remains unchanged)
Intersections of ellipse (i) with 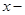 axis
axis 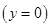
Putting 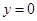 in eq. (i),
in eq. (i), 



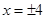
Therefore, Intersections of ellipse (i) with 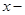 axis are (0, 4) and
axis are (0, 4) and 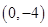 .
.
Intersections of ellipse (i) with  axis
axis 
Putting 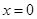 in eq. (i),
in eq. (i), 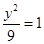

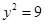

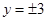
Therefore, Intersections of ellipse (i) with  axis are (0, 3) and
axis are (0, 3) and 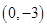 .
.
Now Area of region bounded by ellipse (i) = Total shaded area
= 4 x Area OAB of ellipse in first quadrant
= 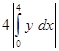 [
[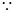 At end B of arc AB of ellipse;
At end B of arc AB of ellipse; 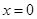 and at end A of arc AB ;
and at end A of arc AB ; 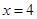 ]
]
= 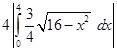 =
= 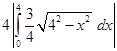
= 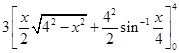
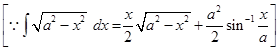
= 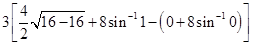 =
= 
= 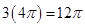 sq. units
sq. units
NCERT Solutions class 12 Maths Exercise 8.1
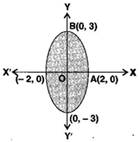
5. Find the area of the region bounded by the ellipse 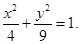
Ans. Equation of ellipse is 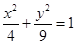
Here 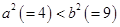
From eq. (i),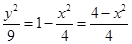

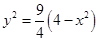

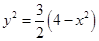 ……….(ii)
……….(ii)
for arc of ellipse in first quadrant.
Ellipse (i) ia symmetrical about 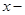 axis,
axis,
( On changing
On changing 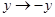 in eq. (i), it remains unchanged)
in eq. (i), it remains unchanged)
Ellipse (i) ia symmetrical about  axis,
axis,
(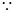 On changing
On changing 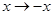 in eq. (i), it remains unchanged)
in eq. (i), it remains unchanged)
Intersections of ellipse (i) with 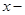 axis
axis 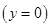
Putting 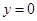 in eq. (i),
in eq. (i), 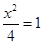

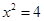

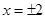
Therefore, Intersections of ellipse (i) with 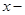 axis are (0, 2) and
axis are (0, 2) and 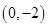 .
.
Intersections of ellipse (i) with  axis
axis 
Putting 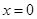 in eq. (i),
in eq. (i), 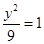

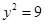

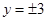
Therefore, Intersections of ellipse (i) with  axis are (0, 3) and
axis are (0, 3) and 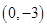 .
.
Now Area of region bounded by ellipse (i) = Total shaded area
= 4 x Area OAB of ellipse in first quadrant
= 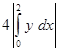 [
[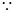 At end B of arc AB of ellipse;
At end B of arc AB of ellipse; 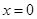 and at end A of arc AB ;
and at end A of arc AB ; 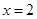 ]
]
= 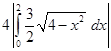 =
= 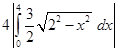
= 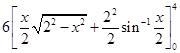
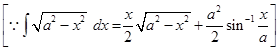
= 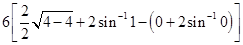
= 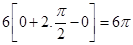 sq. units
sq. units
NCERT Solutions class 12 Maths Exercise 8.1
6. Find the area of the region in the first quadrant enclosed by 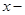 axis, line
axis, line 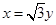 and the circle
and the circle 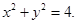
Ans. Step I. To draw the graphs and shade the region whose area we are to find.
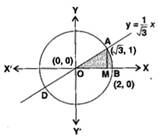
Equation of the circle is 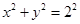 ……….(i)
……….(i)
We know that eq. (i) represents a circle whose centre is (0, 0) and radius is 2.
Equation of the given line is 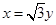

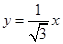 ……….(ii)
……….(ii)
We know that eq. (ii) being of the form  where
where 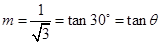

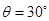 represents a straight line passing through the origin and making angle of
represents a straight line passing through the origin and making angle of 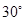 with
with 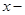 axis.
axis.
Step II. To find values of  and
and 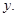 Putting
Putting 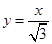 from eq. (ii) in eq. (i),
from eq. (ii) in eq. (i),
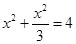

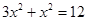

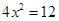

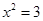

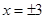
Putting 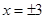 in
in 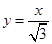 ,
,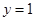 and
and 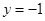
Therefore, the two points of intersections of circle (i) and line (ii) are A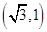 and D
and D .
.
Step III. Now shaded area OAM between segment OA of line (ii) and 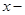 axis
axis
= 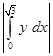
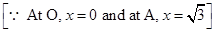
=  =
= 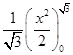 =
= 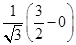 =
= 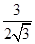 =
= 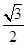 sq. units……….(iii)
sq. units……….(iii)
Step IV. Now shaded area AMB between are AB of circle and 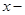 axis.
axis.
= = 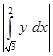
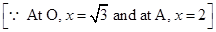
=  From eq. (ii),
From eq. (ii),
= 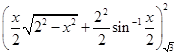 =
= 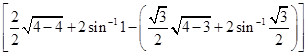
= 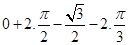 =
= 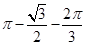 =
=  sq. units……….(iv)
sq. units……….(iv)
Step V. Required shaded area OAB = Area of OAM + Area of AMB
= 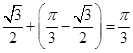 sq. units
sq. units
NCERT Solutions class 12 Maths Exercise 8.1
7. Find the area of the smaller part of the circle 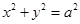 cut off by the line
cut off by the line 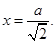
Ans. Equation of the circle is 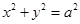 ……….(i)
……….(i)
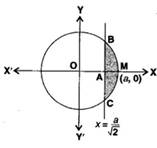
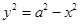

 ……….(ii)
……….(ii)
Here Area of smaller part of the circle 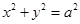 cut off by the line
cut off by the line 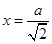 = Area of ABMC = 2 x Area of ABM
= Area of ABMC = 2 x Area of ABM
= 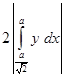 =
=  [From eq. (ii)]
[From eq. (ii)]
= 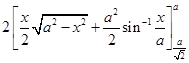
= 
= 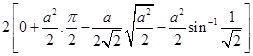
= 
= 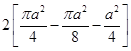
= 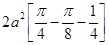
= 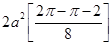
= 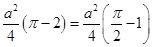 sq. units
sq. units
NCERT Solutions class 12 Maths Exercise 8.1
8. The area between 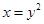 and
and 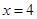 is divided into two equal parts by the line
is divided into two equal parts by the line  find the value of
find the value of 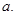
Ans. Equation of the curve (parabola) is 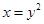 ……(i)
……(i)


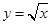
Now area bounded by parabola (i) and vertical line 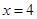 is divided into two equal parts by the vertical line
is divided into two equal parts by the vertical line 
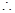 Area OAMB = Area AMBDNC
Area OAMB = Area AMBDNC

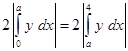

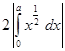 =
= 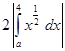

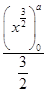 =
= 

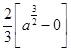 =
= 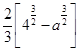

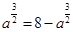

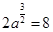

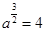

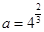
NCERT Solutions class 12 Maths Exercise 8.1
9. Find the area of the region bounded by the parabola  and
and 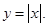
Ans. The required area is the area included between the parabola  and the modulus function
and the modulus function 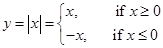

To find: Area between the parabola  and the ray
and the ray 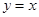 for
for 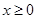
Here, Limits of integration 
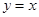

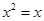

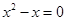

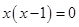

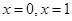
Now, for 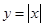 , table of values,
, table of values,
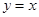 if
if 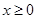
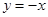 if
if 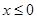
 |
0 | 1 | 2 |
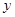 |
0 | 1 | 2 |
 |
0 | 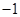 |
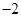 |
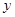 |
0 | 1 | 2 |
Now, Area between parabola  and
and 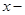 axis between limits
axis between limits 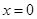 and
and 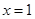
= 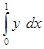 =
= 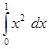 =
= 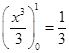 ………..(i)
………..(i)
And Area of ray 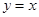 and
and 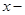 axis,
axis,
= 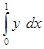 =
= 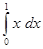 =
=  ………..(ii)
………..(ii)
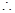 Required shaded area in first quadrant = Area between ray
Required shaded area in first quadrant = Area between ray 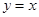 for
for 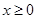 and
and 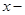 axis –
axis –
Area between parabola  and
and 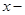 axis in first quadrant
axis in first quadrant
= Area given by eq. (ii) – Area given by eq. (i) = 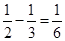 sq. units
sq. units
NCERT Solutions class 12 Maths Exercise 8.1
10. Find the area bounded by the curve 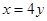 and the line
and the line 
Ans. Step I. Graphs and region of
Integration
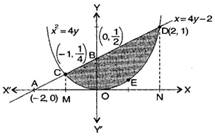
Equation of the given curve is
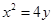 ……..(i)
……..(i)
Equation of the given line is
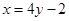 ……….(ii)
……….(ii)

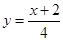
 |
0 | 1 |  |
 |
0 | 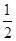 |
0 |
Step II. Putting  from eq. (i) in eq. (ii),
from eq. (i) in eq. (ii),
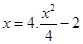

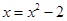

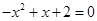

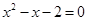

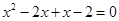

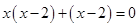

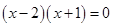

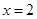 or
or 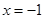
For 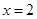 ,from eq. (i),
,from eq. (i),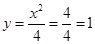
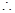 (2, 1)
(2, 1)
For 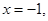 from eq. (i),
from eq. (i),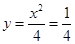
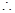
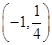
Therefore, the two points of intersection of parabola (i) and line (ii) are C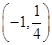 and D (2, 1).
and D (2, 1).
Step III. Area CMOEDN between parabola (i) and 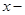 axis =
axis = 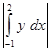 =
= 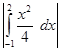
=  =
= 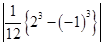 =
= 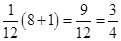 sq. units……..(iii)
sq. units……..(iii)
Step IV. Area of trapezium CMND between line (ii) and 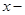 axis =
axis = 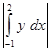 =
= 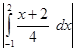
= 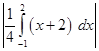 =
= 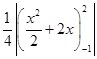 =
= 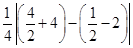 =
= 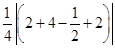
= 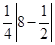 =
= 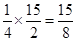 sq. units……….(iv)
sq. units……….(iv)
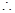 Required shaded area = Area given by eq. (iv) – Area given by eq. (iii)
Required shaded area = Area given by eq. (iv) – Area given by eq. (iii)
= 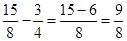 sq. units
sq. units
NCERT Solutions class 12 Maths Exercise 8.1
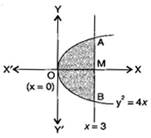
11. Find the area of the region bounded by the curve  and the line
and the line 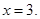
Ans. Equation of the (parabola) curve is
 ………(i)
………(i)

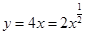 ………(ii)
………(ii)
Here required shaded area OAMB = 2 x Area OAM
=  =
= 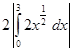 =
= 
= 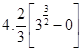 =
= 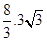 =
=  sq. units
sq. units
12. Choose the correct answer:
Area lying in the first quadrant and bounded by the circle 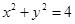 and the lines
and the lines 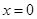 and
and 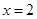 is
is
(A) 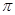
(B) 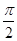
(C) 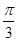
(D) 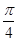
Ans. Equation of the circle is 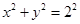 ……….(i)
……….(i)

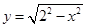 ……….(ii)
……….(ii)

Required area = 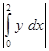 =
= 
= 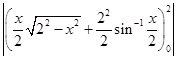
= 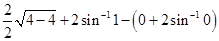
=  =
= 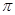 sq. units
sq. units
Therefore, option (A) is correct.
13. Choose the correct answer:
Area of the region bounded by the curve 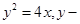 axis and the line
axis and the line 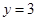 is:
is:
(A) 2
(B) 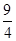
(C) 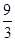
(D) 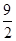
Ans. Equation of the curve (parabola) is 

Required area = Area OAM = 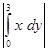 =
= 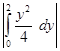
= 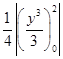 =
= 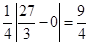 sq. units
sq. units
Therefore, option (B) is correct.
NCERT Solutions class 12 Maths Exercise 8.1
NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes text book solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 20 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide
CBSE App for Class 12
To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now