NCERT Solutions class 12 Maths Exercise 10.3 Class 12 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
Download NCERT solutions for Vector Algebra as PDF.

NCERT Solutions class 12 Maths Vector Algebra
1. Find the angle between two vectors 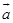 and
and 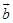 with magnitude
with magnitude 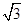 and 2 respectively having
and 2 respectively having 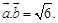
Ans. Given: 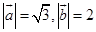 and
and 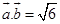
Let 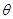 be the angle between the vector
be the angle between the vector 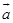 and
and 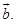
We know that 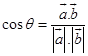

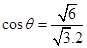
= 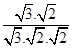 =
= 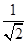

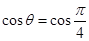

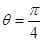
2. Find the angle between the vectors 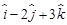 and
and 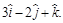
Ans. Given: Let  and
and 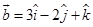

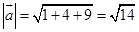 and
and 
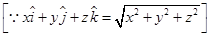
Also 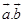
= Product of coefficients of 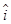 + Product of coefficients of
+ Product of coefficients of  + Product of coefficients
+ Product of coefficients 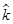
= 
Let 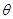 be the angle between the vector
be the angle between the vector 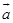 and
and 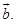
We know that 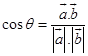

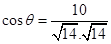
=  =
= 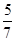

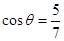

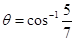
3. Find the projection of the vector 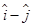 on the vector
on the vector 
Ans. Let 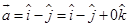 and
and 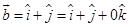
Projection of vector 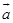 and
and 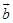 =
= 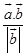
= 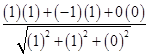
= 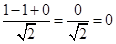
If projection of vector 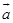 and
and 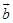 is zero, then vector
is zero, then vector 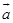 is perpendicular to
is perpendicular to 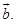
4. Find the projection of the vector 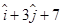 on the vector
on the vector 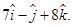
Ans. Let 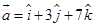 and
and 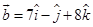
Projection of vector 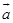 and
and 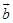 =
= 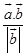
= 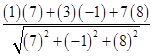
= 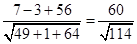
5. Show that each of the given three vectors is a unit vector:
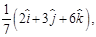
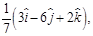

Also show that they are mutually perpendicular to each other.
Ans. Let 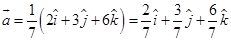 ……….(i)
……….(i)
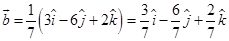 ……….(ii)
……….(ii)
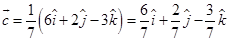 ……….(iii)
……….(iii)


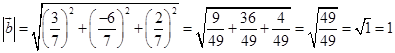
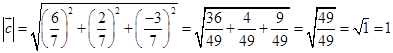
 Each of the three given vectors
Each of the three given vectors 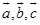 is a unit vector.
is a unit vector.
From eq. (i) and (ii),
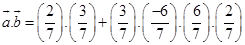
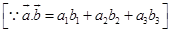
= 

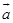 and
and 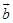 are perpendicular to each other.
are perpendicular to each other.
From eq. (ii) and eq. (iii),
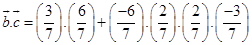
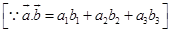
= 

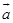 and
and 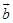 are perpendicular to each other.
are perpendicular to each other.
From eq. (i) and (iii),

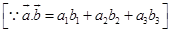
= 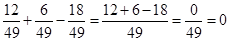

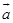 and
and 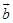 are perpendicular to each other.
are perpendicular to each other.
Hence, 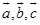 are mutually perpendicular vectors.
are mutually perpendicular vectors.
6. Find 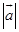 and
and 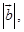 if
if 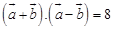 and
and 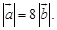
Ans. Given: 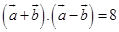 and
and 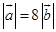 ……….(i)
……….(i)

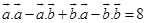



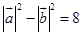 ……….(ii)
……….(ii)
Putting 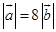 in eq. (ii),
in eq. (ii),
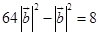



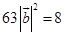

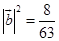

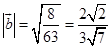
Putting 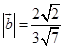 in eq (i),
in eq (i),
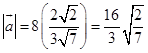
7. Evaluate the product 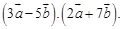
Ans. Given: 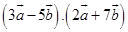 =
= 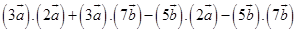
= 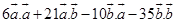
= 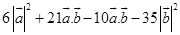
= 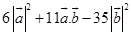
NCERT Solutions class 12 Maths Exercise 10.3
8. Find the magnitude of two vectors 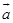 and
and 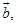 having the same magnitude such that the angle between them is
having the same magnitude such that the angle between them is  and their scalar product is
and their scalar product is 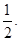
Ans. Given: 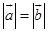 , angle
, angle 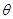 (say) between
(say) between 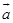 and
and 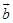 is
is  and their scalar (i.e., dot) product =
and their scalar (i.e., dot) product = 

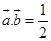

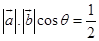
Putting 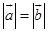 and
and 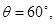 we have
we have 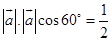

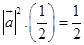

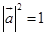

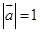

 =
= 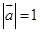

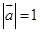 and
and 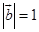
NCERT Solutions class 12 Maths Exercise 10.3
9. Find 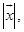 if for a unit vector
if for a unit vector 

Ans. Given: 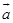 is a unit vector
is a unit vector 
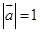 ……….(i)
……….(i)
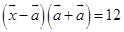

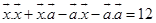

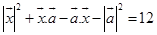




Putting 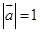 from eq. (i),
from eq. (i), 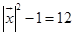

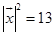

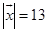
NCERT Solutions class 12 Maths Exercise 10.3
10. If 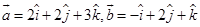 and
and  are such that
are such that 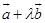 is perpendicular to
is perpendicular to 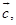 then find the value of
then find the value of 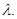
Ans. Given: 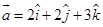 ,
, 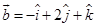 and
and 
Now 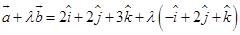 =
=
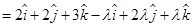

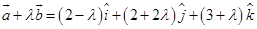
Again,  =
= 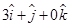
Since, 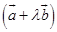 is perpendicular to
is perpendicular to 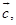 therefore,
therefore, 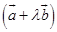 .
.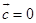

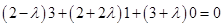

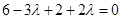

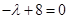

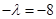

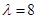
NCERT Solutions class 12 Maths Exercise 10.3
11. Show that 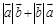 is perpendicular to
is perpendicular to 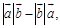 for any two non-zero vectors
for any two non-zero vectors 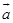 and
and 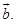
Ans. Let 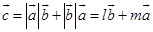 , where
, where 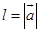 and
and 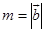
Let 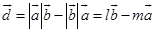
Now 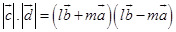
= 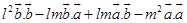
= 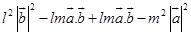
= 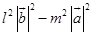
Putting, 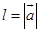 and
and 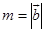 ,
,
=  = 0
= 0

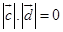
Therefore, vectors 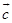 and
and 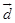 are perpendicular ot each other.
are perpendicular ot each other.
NCERT Solutions class 12 Maths Exercise 10.3
12. If 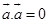 and
and  , then what can be concluded about the vector
, then what can be concluded about the vector 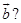
Ans. Given: 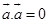

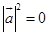

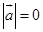
Again 

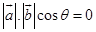

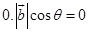
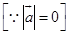
 0 = 0 for all (any vector
0 = 0 for all (any vector 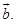 )
)
Therefore, 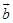 can be any vector.
can be any vector.
NCERT Solutions class 12 Maths Exercise 10.3
13. If 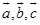 are unit vectors such that
are unit vectors such that  find the value of
find the value of 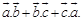
Ans. Since, 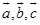 are unit vectors.
are unit vectors.
Therefore, 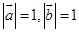 and
and 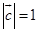 ……….(i)
……….(i)
Also given 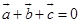

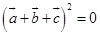

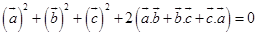
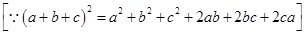

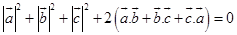
Putting the values from eq. (i), we get

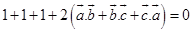

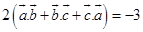

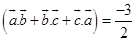
NCERT Solutions class 12 Maths Exercise 10.3
14. If either vector 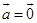 or
or 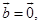 then
then  . But the converse need not be true. Justify your answer with an example.
. But the converse need not be true. Justify your answer with an example.
Ans. Case I: Vector 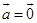 . Therefore by definition of zero vector,
. Therefore by definition of zero vector, 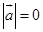 ……….(i)
……….(i)

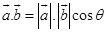
= 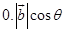 [From eq. (i)]
[From eq. (i)]


Case II: Vector 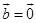 . Therefore by definition of zero vector,
. Therefore by definition of zero vector, 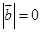 ……….(ii)
……….(ii)

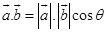
= 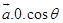 [From eq. (ii)]
[From eq. (ii)]


But the converse is not true.
Justification: Let 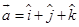
Therefore, 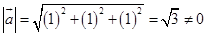
Therefore, 
Again let 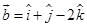

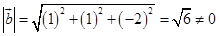
Therefore, 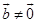
But 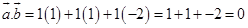
Hence, here  , but
, but  and
and 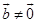 .
.
NCERT Solutions class 12 Maths Exercise 10.3
15. If the vertices A, B, C of a triangle ABC are 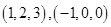 and (0, 1, 2) respectively, then find
and (0, 1, 2) respectively, then find 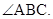
Ans. Vertices A, B, C of a triangle are A (1, 2,3 ), B and C (0, 1, 2) respectively.
and C (0, 1, 2) respectively.
 Position vector of point A =
Position vector of point A = 
Position vector of point B = 
Position vector of point C = 
Now  = Position vector of point A – Position vector of point B
= Position vector of point A – Position vector of point B
= 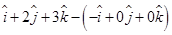
=  ……….(i)
……….(i)
And 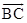 = Position vector of point C – Position vector of point B
= Position vector of point C – Position vector of point B
= 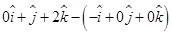
= 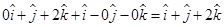 ……….(ii)
……….(ii)
Let 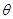 be the angle between the vectors
be the angle between the vectors  and
and 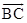 .
.

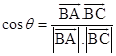
= 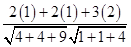 [Using eq. (i) and (ii)]
[Using eq. (i) and (ii)]

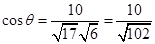

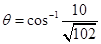
NCERT Solutions class 12 Maths Exercise 10.3
16. Show that the points A (1, 2, 7), B (2, 6, 3) and C are collinear.
are collinear.
Ans. Vertices A, B, C of a triangle are A (1, 2, 7), B (2, 6, 3) and C respectively.
respectively.
 Position vector of point A =
Position vector of point A = 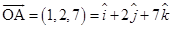
Position vector of point B = 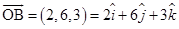
Position vector of point C = 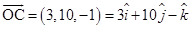
Now 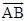 = Position vector of point B – Position vector of point A
= Position vector of point B – Position vector of point A
= 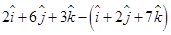
= 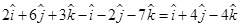 ……….(i)
……….(i)
And 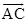 = Position vector of point C – Position vector of point A
= Position vector of point C – Position vector of point A
= 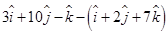
= 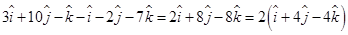 ……….(ii)
……….(ii)

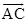 = 2.
= 2.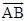 [Using eq. (i)]
[Using eq. (i)]
 Vectors
Vectors 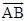 and
and 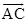 are collinear and parallel.
are collinear and parallel. 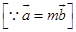
Thus, points A, B and C are collinear.
And also vectors 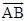 and
and 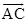 have a common point A and hence can’t be parallel.
have a common point A and hence can’t be parallel.
NCERT Solutions class 12 Maths Exercise 10.3
17. Show that the vectors 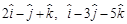 and
and 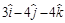 form the vertices of a right angled triangle.
form the vertices of a right angled triangle.
Ans. Let the given position vectors be A, B, C.
 Position vector of point A is
Position vector of point A is  Position vector of point B is
Position vector of point B is 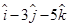 and Position vector of point C is
and Position vector of point C is 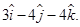

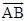 = Position vector of B – Position vector of A
= Position vector of B – Position vector of A
= 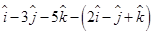
= 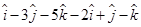 =
= 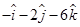 ……….(i)
……….(i)
 = Position vector of C – Position vector of B
= Position vector of C – Position vector of B
= 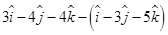
= 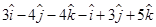 =
= 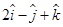 ……….(ii)
……….(ii)
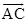 = Position vector of C – Position vector of A
= Position vector of C – Position vector of A
= 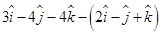
= 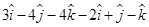 =
= 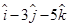 ……….(iii)
……….(iii)
Adding eq. (i) and (ii),
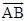 +
+ 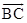 =
= 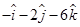 +
+ 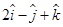 =
= 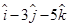 =
= 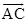 [Using eq. (iii)]
[Using eq. (iii)]
Therefore, by Triangle law of addition of vectors, points A, B, C are the vertices of a triangle ABC.
Now from eq. (i) and (ii),
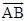 .
.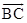 =
=  =
= 
Again from eq. (ii) and (iii),
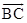 .
.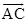 =
= 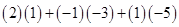 = 2 + 3 – 5 = 0
= 2 + 3 – 5 = 0

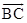 is perpendicular to
is perpendicular to 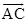 .
.
 Angle C is
Angle C is  Therefore
Therefore 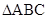 is a right angled at C.
is a right angled at C.
Thus, A, B, C are the vertices of a right angled triangle.
NCERT Solutions class 12 Maths Exercise 10.3
18. If 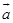 is a non-zero vector of magnitude
is a non-zero vector of magnitude 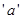 and
and 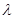 is a non-zero scalar, then
is a non-zero scalar, then 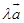 is a unit vector if:
is a unit vector if:
(A) 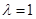
(B) 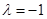
(C) 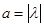
(D) 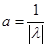
Ans. Given: 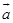 is a non-zero vector of magnitude
is a non-zero vector of magnitude 

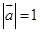
Also given 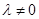 and
and 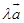 is a unit vector.
is a unit vector.



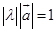

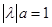

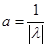
Therefore, option (D) is correct.
NCERT Solutions class 12 Maths Exercise 10.3
NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes text book solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 13 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide
CBSE App for Students
To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now