NCERT Solutions class 12 Maths Exercise 1.1 solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT textbooks. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
Download NCERT solutions for Relations and Functions as PDF.

NCERT Solutions class 12 Maths Relations and Functions
1. Determine whether each of the following relations are reflexive, symmetric and transitive:
(i) Relation R in the set A = {1, 2, 3, ……….. 13, 14} defined as R = 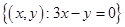 .
.
(ii) Relation R in the set N of natural numbers defined as R =.
(iii) Relation R in the set A = {1, 2, 3, 4, 5, 6} as R = 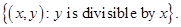
(iv) Relation R in the set Z of all integers defined as R = 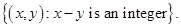
(v) Relation R in the set A of human beings in a town at a particular time given by:
(a) R = 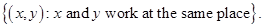
(b) R = 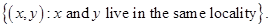
(c) R = 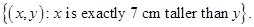
(d) R = 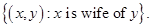
(e) R = 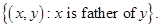
Ans. (i) R = 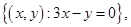 in A = {1, 2, 3, 4, 5, 6, ……13, 14}
in A = {1, 2, 3, 4, 5, 6, ……13, 14}
Clearly R = {(1, 3), (2, 6), (3, 9), (4, 12)}
Since,  R,
R,  R is not reflexive.
R is not reflexive.
Again  R but
R but 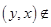 R
R  R is not symmetric.
R is not symmetric.
Also (1, 3) R and (3, 9)
R and (3, 9)  R but (1, 9)
R but (1, 9) 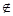 R,
R,  R is not transitive.
R is not transitive.
Therefore, R is neither reflexive, nor symmetric and nor transitive.
(ii) R = 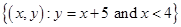 in set N of natural numbers.
in set N of natural numbers.
Clearly R = {(1, 6), (2, 7), (3, 8)}
Now  R,
R,  R is not reflexive.
R is not reflexive.
Again  R but
R but 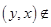 R
R  R is not symmetric.
R is not symmetric.
Also (1, 6) R and (2, 7)
R and (2, 7)  R but (1, 7)
R but (1, 7) 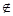 R,
R,  R is not transitive.
R is not transitive.
Therefore, R is neither reflexive, nor symmetric and nor transitive.
(iii) R = 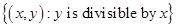 in A = {1, 2, 3, 4, 5, 6}
in A = {1, 2, 3, 4, 5, 6}
Clearly R = (1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (2, 2), (2, 4), (2, 6), (3, 3), (3, 6), (4, 4), (5, 5), (6, 6)
Now 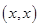 i.e., (1, 1), (2, 2) and (3, 3)
i.e., (1, 1), (2, 2) and (3, 3)  R
R  R is reflexive.
R is reflexive.
Again  i.e., (1, 2)
i.e., (1, 2) R but
R but 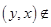 R
R  R is not symmetric.
R is not symmetric.
Also (1, 4) R and (4, 4)
R and (4, 4)  R and (1, 4)
R and (1, 4)  R,
R,  R is transitive.
R is transitive.
Therefore, R is reflexive and transitive but not symmetric.
(iv) R = 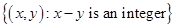 in set Z of all integers.
in set Z of all integers.
Now 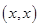 i.e., (1, 1) = 1 – 1 = 0
i.e., (1, 1) = 1 – 1 = 0  Z
Z  R is reflexive.
R is reflexive.
Again 
 R and
R and 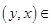 R, i.e.,
R, i.e., 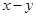 and
and 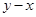 are an integer
are an integer R is symmetric.
R is symmetric.
Also 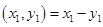
 Z and
Z and 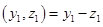
 Z and
Z and
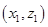
 R,
R,  R is transitive.
R is transitive.
Therefore, R is reflexive, symmetric and transitive.
(v) Relation R in the set A of human being in a town at a particular time.
(a) R = 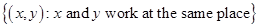
Since  R, because
R, because  and
and  work at the same place.
work at the same place.  R is reflexive.
R is reflexive.
Now, if  R and
R and  R, since
R, since  and
and 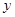 work at the same place and
work at the same place and 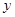 and
and  work at the same place.
work at the same place.  R is symmetric.
R is symmetric.
Now, if  R and
R and 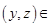 R
R 
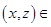 R.
R.  R is transitive
R is transitive
Therefore, R is reflexive, symmetric and transitive.
(b) R = 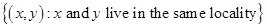
Since  R, because
R, because  and
and  live in the same locality.
live in the same locality.  R is reflexive.
R is reflexive.
Also  R
R 
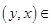 R because
R because  and
and 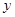 live in same locality and
live in same locality and 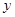 and
and  also live in same locality.
also live in same locality.  R is symmetric.
R is symmetric.
Again  R and
R and 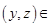 R
R 
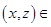 R
R  R is transitive.
R is transitive.
Therefore, R is reflexive, symmetric and transitive.
(c) R = 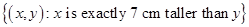
 is not exactly 7 cm taller than
is not exactly 7 cm taller than  , so
, so  R
R  R is not reflexive.
R is not reflexive.
Also  is exactly 7 cm taller than
is exactly 7 cm taller than 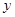 but
but 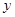 is not 7 cm taller than
is not 7 cm taller than  , so
, so  R but
R but 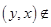 R
R  R is not symmetric.
R is not symmetric.
Now  is exactly 7 cm taller than
is exactly 7 cm taller than 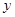 and
and 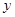 is exactly 7 cm taller than
is exactly 7 cm taller than 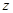 then it does not imply that
then it does not imply that  is exactly 7 cm taller than
is exactly 7 cm taller than 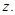
 R is not transitive.
R is not transitive.
Therefore, R is neither reflexive, nor symmetric and nor transitive.
(d) R = 
 is not wife of
is not wife of  , so
, so  R
R  R is not reflexive.
R is not reflexive.
Also  is wife of
is wife of 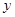 but
but 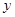 is not wife of
is not wife of  , so
, so  R but
R but 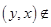 R
R  R is not symmetric.
R is not symmetric.
Also  R and
R and 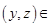 R then
R then 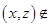 R
R  R is not transitive.
R is not transitive.
Therefore, R is neither reflexive, nor symmetric and nor transitive.
(e) R = 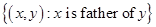
 is not father of
is not father of  , so
, so  R
R  R is not reflexive.
R is not reflexive.
Also  is father of
is father of 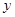 but
but 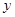 is not father of
is not father of  ,
,
so  R but
R but 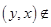 R
R  R is not symmetric.
R is not symmetric.
Also  R and
R and 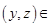 R then
R then 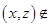 R
R  R is not transitive.
R is not transitive.
Therefore, R is neither reflexive, nor symmetric and nor transitive.
2. Show that the relation R in the set R of real numbers defined as R = 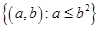 is neither reflexive nor symmetric nor transitive.
is neither reflexive nor symmetric nor transitive.
Ans. R = 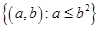 , Relation R is defined as the set of real numbers.
, Relation R is defined as the set of real numbers.
(i) Whether 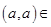 R, then
R, then 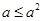 which is false.
which is false.  R is not reflexive.
R is not reflexive.
(ii) Whether 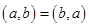 , then
, then 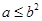 and
and 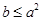 , it is false.
, it is false.  R is not symmetric.
R is not symmetric.
(iii) Now 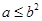 ,
, 

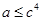 , which is false.
, which is false.  R is not transitive
R is not transitive
Therefore, R is neither reflexive, nor symmetric and nor transitive.
3. Check whether the relation R defined in the set {1, 2, 3, 4, 5, 6} as R = 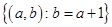 is reflexive, symmetric or transitive.
is reflexive, symmetric or transitive.
Ans. R = 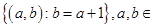 R
R
Now R = {(1, 2), (2, 3), (3, 4), (4, 5), (5, 6)} and 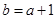
(i) When 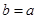

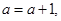 which is false, so
which is false, so 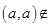 R,
R,  R is not reflexive.
R is not reflexive.
(ii) Whether 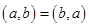 , then
, then 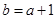 and
and  , false
, false  R is not symmetric.
R is not symmetric.
(iii) Now if 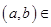 R,
R, 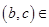 R
R 
 R
R
(iv) Then 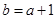 and
and 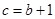

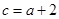 which is false.
which is false.  R is not transitive.
R is not transitive.
Therefore, R is neither reflexive, nor symmetric and nor transitive.
4. Show that the relation R in R defined as R = 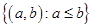 , is reflexive and transitive but not symmetric.
, is reflexive and transitive but not symmetric.
Ans. (i) 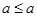 which is true, so
which is true, so 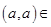 R,
R,  R is reflexive.
R is reflexive.
(ii) 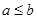 but
but 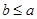
 R is not symmetric.
R is not symmetric.
(iii) 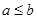 and
and 

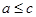 which is true.
which is true.  R is transitive.
R is transitive.
Therefore, R is reflexive and transitive but not symmetric.
NCERT Solutions class 12 Maths Exercise 1.1
5. Check whether the relation R in R defined by R = 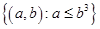 is reflexive, symmetric or transitive.
is reflexive, symmetric or transitive.
Ans. (i) For 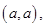
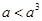 which is false.
which is false.  R is not reflexive.
R is not reflexive.
(ii) For 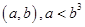 and
and 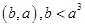 which is false.
which is false.  R is not symmetric.
R is not symmetric.
(iii) For 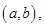
 and
and  ,
, 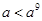 which is false.
which is false.  R is not transitive.
R is not transitive.
Therefore, R is neither reflexive, nor symmetric and nor transitive.
NCERT Solutions class 12 Maths Exercise 1.1
6. Show that the relation in the set A = {1, 2, 3} given by R = {(1, 2), (2, 1)} is symmetric but neither reflexive nor transitive.
Ans. R = {(1, 2), (2, 1)}, so for 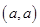 , (1, 1)
, (1, 1) 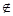 R.
R.  R is not reflexive.
R is not reflexive.
Also if 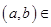 then
then 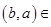 R
R R is symmetric.
R is symmetric.
Now 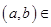 R and
R and 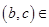 then does not imply
then does not imply 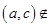 R
R  R is not transitive..
R is not transitive..
Therefore, R is symmetric but neither reflexive nor transitive.
NCERT Solutions class 12 Maths Exercise 1.1
7. Show that the relation R in the set A of all the books in a library of a college, given by R = 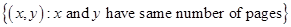 is an equivalence relation.
is an equivalence relation.
Ans. Books  and
and  have same number of pages
have same number of pages 
 R
R  R is reflexive.
R is reflexive.
If  R
R 
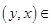 R, so
R, so 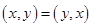
 R is symmetric.
R is symmetric.
Now if  R,
R, 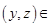 R
R 
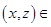 R
R  R is transitive.
R is transitive.
Therefore, R is an equivalence relation.
NCERT Solutions class 12 Maths Exercise 1.1
8. Show that the relation R in the set A = {1, 2, 3, 4, 5} given by R = 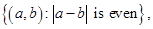 is an equivalence relation. Show that all the elements of {1, 3, 5} are related to each other and all the elements of {2, 4} are related to each other. But no element of {1, 3, 5} is related to any element of {2, 4}.
is an equivalence relation. Show that all the elements of {1, 3, 5} are related to each other and all the elements of {2, 4} are related to each other. But no element of {1, 3, 5} is related to any element of {2, 4}.
Ans. A = {1, 2, 3, 4, 5} and R = 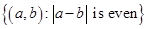 , then R = {(1, 3), (1, 5), (3, 5), (2, 4)}
, then R = {(1, 3), (1, 5), (3, 5), (2, 4)}
(a) For 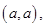
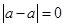 which is even.
which is even.  R is reflexive.
R is reflexive.
If 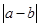 is even, then
is even, then 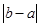 is also even.
is also even.  R is symmetric.
R is symmetric.
Now, if 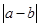 and
and 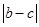 is even then
is even then 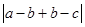

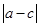 is also even.
is also even.  R is transitive.
R is transitive.
Therefore, R is an equivalence relation.
(b) Elements of {1, 3, 5} are related to each other.
Since 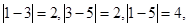 all are even numbers
all are even numbers
 Elements of {1, 3, 5} are related to each other.
Elements of {1, 3, 5} are related to each other.
Similarly elements of (2, 4) are related to each other.
Since 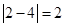 an even number, then no element of the set {1, 3, 5} is related to any element of (2, 4).
an even number, then no element of the set {1, 3, 5} is related to any element of (2, 4).
Hence no element of {1, 3, 5} is related to any element of {2, 4}.
NCERT Solutions class 12 Maths Exercise 1.1
9. Show that each of the relation R in the set A =  given by:
given by:
(i) R = 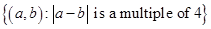
(ii) R =  is an equivalence relation. Find the set of all elements related to 1 in each case.
is an equivalence relation. Find the set of all elements related to 1 in each case.
Ans. (a) (i) A = 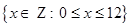
 A = {0, 1, 2, 3, ……….., 12}
A = {0, 1, 2, 3, ……….., 12}
Now R = 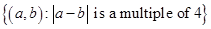
 R = {(4, 0), (0, 4), (5, 1), (1, 5), (6, 2), (2, 6), ….. (12, 9), (9, 12), …. (8, 0), (0, 8), ….. (8, 4), (4, 8), …… (12, 12)}
R = {(4, 0), (0, 4), (5, 1), (1, 5), (6, 2), (2, 6), ….. (12, 9), (9, 12), …. (8, 0), (0, 8), ….. (8, 4), (4, 8), …… (12, 12)}
Here,  is a multiple of 4.
is a multiple of 4.

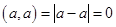 is a multiple of 4.
is a multiple of 4.  R is reflexive.
R is reflexive.
Also we observe that 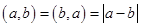
 R is symmetric.
R is symmetric.
And 

 is the multiple of 4.
is the multiple of 4.  R is transitive.
R is transitive.
Hence R is an equivalence relation.
(ii) R =  and A = {0, 1, 2, 3, ……….., 12}
and A = {0, 1, 2, 3, ……….., 12}
 R = {(0, 0), (1, 1), (2, 2), …….. (12, 12)}
R = {(0, 0), (1, 1), (2, 2), …….. (12, 12)}
For 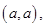
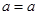
 R is reflexive.
R is reflexive.
As 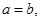 then
then 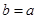
 R is symmetric.
R is symmetric.
Also 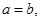
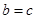 then
then 
 R is transitive.
R is transitive.
Hence R is an equivalence relation.
(b) Now set of all elements related to 1 in each case.
(i) Required set = {1, 5, 9} (ii) Required set = {1}
NCERT Solutions class 12 Maths Exercise 1.1
10. Give an example of a relation, which is:
(i) Symmetric but neither reflexive nor transitive.
(ii) Transitive but neither reflexive nor symmetric.
(iii) Reflexive and symmetric but not transitive.
(iv) Reflexive and transitive but not symmetric.
(v) Symmetric and transitive but not reflexive.
Ans. (i) The relation “is perpendicular to” 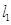 is not perpendicular to
is not perpendicular to 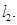
If  then
then 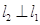 , however if
, however if  and
and  then
then 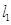 is not perpendicular to
is not perpendicular to 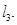
So it is clear that R “is perpendicular to” is a symmetric but neither reflexive nor transitive.
(ii) Relation R = 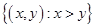
We know that 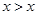 is false. Also
is false. Also 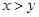 but
but 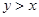 is false and if
is false and if 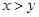 ,
, 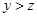 this implies
this implies 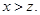
Therefore, R is transitive, but neither reflexive nor symmetric.
(iii) “is friend of” R = 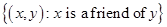
It is clear that  is friend of
is friend of 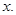
 R is reflexive.
R is reflexive.
Also  is friend of
is friend of 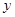 and
and 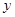 is friend of
is friend of 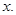
 R is symmetric.
R is symmetric.
Also if  is friend of
is friend of 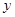 and
and 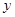 is friend of
is friend of 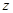 then
then
 cannot be friend of
cannot be friend of 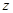
 R is not transitive.
R is not transitive.
Therefore, R is reflexive and symmetric but not transitive.
(iv) “is greater or equal to” R = 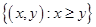
It is clear that 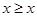
 R is reflexive.
R is reflexive.
And 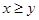 does not imply
does not imply 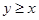
 R is not symmetric.
R is not symmetric.
But 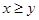 ,
, 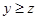

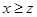
 R is transitive.
R is transitive.
Therefore, R is reflexive and transitive but not symmetric.
(v) “is brother of” R = 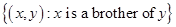
It is clear that  is not the brother of
is not the brother of 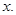
 R is not reflexive.
R is not reflexive.
Also  is brother of
is brother of 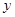 and
and 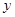 is brother of
is brother of 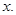
 R is symmetric.
R is symmetric.
Also if  is brother of
is brother of 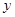 and
and 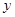 is brother of
is brother of 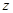 then
then
 can be brother of
can be brother of 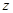
 R is transitive.
R is transitive.
Therefore, R is symmetric and transitive but not reflexive.
NCERT Solutions class 12 Maths Exercise 1.1
11. Show that the relation R in the set A of points in a plane given by R = {(P. Q) : distance of the point P from the origin is same as the distance of the point Q from the origin}, is an equivalence relation. Further, show that the set of all points related to a point P  (0, 0) is the circle passing through P with origin as centre.
(0, 0) is the circle passing through P with origin as centre.
Ans. Part I: R = {(P, Q): distance of the point P from the origin is the same as the distance of the point Q from the origin}
Let P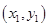 and Q
and Q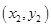 and O (0, 0).
and O (0, 0).
 OP = OQ
OP = OQ 
 =
= 

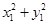 =
= 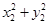
Now, For (P, P), OP = OP  R is reflexive.
R is reflexive.
Also OP = OQ and OQ = OP  (P, Q) = (Q, P)
(P, Q) = (Q, P) R
R  R is symmetric.
R is symmetric.
Also OP = OQ and OQ = OR  OP = OQ
OP = OQ  R is transitive.
R is transitive.
Therefore, R is an equivalent relation.
Part II: As 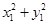 =
= 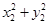 =
= 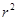 (let)
(let) 
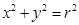 which represents a circle with centre (0, 0) and radius
which represents a circle with centre (0, 0) and radius 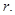
NCERT Solutions class 12 Maths Exercise 1.1
12. Show that the relation R defined in the set A of all triangles as R = {(T1, T2) : T1 is similar to T2}, is equivalence relation. Consider three right angle triangles T1 with sides 3, 4, 5, T2 with sides 5, 12, 13 and T3 with sides 6, 8, 10. Which triangles among T1, T2 and T3 are related?
Ans. Part I: R = {(T1, T2): T1 is similar to T2} and T1, T2 are triangle.
We know that each triangle similar to itself and thus (T1, T2) R
R  R is reflexive.
R is reflexive.
Also two triangles are similar, then T1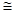 T2
T2  T1
T1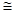 T2
T2  R is symmetric.
R is symmetric.
Again, if then T1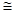 T2 and then T2
T2 and then T2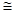 T3
T3  then T1
then T1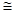 T3
T3  R is transitive.
R is transitive.
Therefore, R is an equivalent relation.
Part II: It is given that T1, T2 and T3 are right angled triangles.
 T1 with sides 3, 4, 5 T2 with sides 5, 12, 13 and
T1 with sides 3, 4, 5 T2 with sides 5, 12, 13 and
T3 with sides 6, 8, 10
Since, two triangles are similar if corresponding sides are proportional.
Therefore, 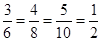
Therefore, T1 and T3 are related.
NCERT Solutions class 12 Maths Exercise 1.1
13. Show that the relation R defined in the set A of all polygons as R = {(P1, P2) : P1 and P2 have same number of sides}, is an equivalence relation. What is the set of all elements in A related to the right angle triangle T with sides 3, 4, and 5?
Ans. Part I: R = {(P1, P2): P1 and P2 have same number of sides}
(i) Consider the element (P1, P2), it shows P1 and P2 have same number of sides. Therefore, R is reflexive.
(ii) If (P1, P2) R then also (P2, P1)
R then also (P2, P1) R
R
 (P1, P2) = (P2, P1) as P1 and P2 have same number of sides, therefore, R is symmetric.
(P1, P2) = (P2, P1) as P1 and P2 have same number of sides, therefore, R is symmetric.
(iii) If (P1, P2) R and (P2, P3)
R and (P2, P3) R then also (P1, P3)
R then also (P1, P3) R as P1, P2 and P3 have same number of sides, therefore, R is transitive.
R as P1, P2 and P3 have same number of sides, therefore, R is transitive.
Therefore, R is an equivalent relation.
Part II: we know that if 3, 4, 5 are the sides of a triangle, then the triangle is right angled triangle. Therefore, the set A is the set of right angled triangle.
NCERT Solutions class 12 Maths Exercise 1.1
14. Let L be the set of all lines in XY plane and R be the relation in L defined as R = {(L1, L2) : L1 is parallel to L2}. Show that R is an equivalence relation. Find the set of all lines related to the line 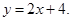
Ans. Part I: R = {(L1, L2): L1 is parallel to L2}
(i) It is clear that L1  L1 i.e., (L1, L1)
L1 i.e., (L1, L1) R
R  R is reflexive.
R is reflexive.
(ii) If L1  L2 and L2
L2 and L2  L1 then (L1, L2)
L1 then (L1, L2) R
R  R is symmetric.
R is symmetric.
(iii) If L1  L2 and L2
L2 and L2  L3
L3  L1
L1  L3
L3  R is transitive.
R is transitive.
Therefore, R is an equivalent relation.
Part II: All the lines related to the line 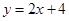 and
and 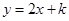 where
where 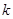 is a real number.
is a real number.
NCERT Solutions class 12 Maths Exercise 1.1
15. Let R be the relation in the set {1, 2, 3, 4} given by R = {(1, 2), (2, 2), (1, 1), (4, 4), (1, 3), (3, 3), (3, 2)}. Choose the correct answer:
(A) R is reflexive and symmetric but not transitive.
(B) R is reflexive and transitive but not symmetric.
(C) R is symmetric and transitive but not reflexive.
(D) R is an equivalence relation.
Ans. Let R be the relation in the set {1, 2, 3, 4} is given by
R = {(1, 2), (2, 2), (1, 1), (4, 4), (1, 3), (3, 3), (3, 2)}
(a) (1, 1), (2, 2), (3, 3), (4, 4)  R
R  R is reflexive.
R is reflexive.
(b) (1, 2)  R but (2, 1)
R but (2, 1) 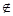 R
R  R is not symmetric.
R is not symmetric.
(c) If (1, 3)  R and (3, 2)
R and (3, 2)  R then (1, 2)
R then (1, 2)  R
R  R is transitive.
R is transitive.
Therefore, option (B) is correct.
NCERT Solutions class 12 Maths Exercise 1.1
16. Let R be the relation in the set N given by R =  Choose the correct answer:
Choose the correct answer:
(A) (2, 4)  R
R
(B) (3, 8)  R
R
(C) (6, 8)  R
R
(D) (8, 7)  R
R
Ans. Given: 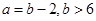
(A) 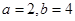 , Here
, Here 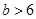 is not true, therefore, this option is incorrect.
is not true, therefore, this option is incorrect.
(B) 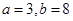 and
and 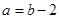

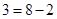
 3 = 6, which is false.
3 = 6, which is false.
Therefore, this option is incorrect.
(C) 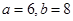 and
and 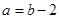

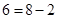
 6 = 6, which is true.
6 = 6, which is true.
Therefore, option (C) is correct.
(D)  and
and 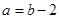

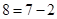
 8 = 5, which is false.
8 = 5, which is false.
NCERT Solutions class 12 Maths Exercise 1.1
NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes textbook solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 13 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide
CBSE App for Students
To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now