Class Mark = ${ Upper\ class\ Limit + Lower\ Class\ Limit\over 2}$

Class Mark = ${ 110+90\over 2}$

Class Mark = ${ 200\over 2}$

Class Mark = 100

27x³ + y³ + z³ - 9xyz.
(3x)³ + (y)³ + (z)³ - 3(3x)(y)(z)
= (3x + y + z){(3x)² + (y)² + (z)² - (3x)(y) - (y)(z) - (z)(3x)}
(Using Identity a³+ b³+ c³– 3abc= (a + b + c)(a²+ b²+ c²– ab – bc – ca))
= (3x + y + z)(9x² + y² + z² - 3xy - yz - 3zx).

In order to get the result we have to find the HCF of 2002 and 2618. which is given by
2002 = 2 $\times$ 7 $\times$ 11 $\times$ 13 and
2618 = 2 $\times$ 7 $\times$ 11 $\times$ 17
Hence  HCF = 2 $\times$ 7 $\times$ 11 = 154

According to the question, given equation is $2x + 3y + 15 = 0.$
So, putting x = 2k - 3 and y = k + 2 in equation, we get
$\Rightarrow$ $2(2k - 3) + 3(k + 2) +15 = 0$
$\Rightarrow$ $4k - 6+ 3k + 6 + 15 = 0$
$\Rightarrow$ $7k + 15 = 0$
$\Rightarrow 7 k=-15 \\ \Rightarrow \ k=-\frac{15}{7}$

We need to prove that every line segment has one and only one mid-point. Let us consider the given below line segment AB and assume that C and D are the mid-points of the line segment AB

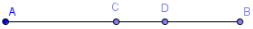
If C is the mid-point of line segment AB, then
AC = CB.
An axiom of the Euclid says that “If equals are added to equals, the wholes are equal.”
AC + AC = CB + AC....(i)
From the figure, we can conclude that CB + AC will coincide with AB.
An axiom of the Euclid says that “Things which coincide with one another are equal to one another.” AC + AC = AB....(ii)
An axiom of the Euclid says that “Things which are equal to the same thing are equal to one another.”
Let us compare equations (i) and (ii), to get
AC + AC = AB, or 2AC = AB.(iii)
If D is the mid-point of line segment AB, then
AD = DB.
An axiom of the Euclid says that “If equals are added to equals, the wholes are equal.”
AD + AD = DB + AD....(iv)
From the figure, we can conclude that DB + AD will coincide with AB.
An axiom of the Euclid says that “Things which coincide with one another are equal to one another.”
AD + AD = AB....(v)
An axiom of the Euclid says that “Things which are equal to the same thing are equal to one another.”
Let us compare equations (iv) and (v), to get
AD + AD = AB, or
2AD = AB....(vi)
An axiom of the Euclid says that “Things which are equal to the same thing are equal to one another.” Let us compare equations (iii) and (vi), to get
2AC = 2AD.
An axiom of the Euclid says that “Things which are halves of the same things are equal to one another.” AC = AD.
Therefore, we can conclude that the assumption that we made previously is false and a line segment has one and only one mid-point.

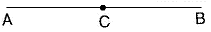
Given, AC = BC
AC + AC = BC + AC . . . . [AC are added to both the side]
2AC = AB . . . . [BC + AC coincides with AB]
$\therefore$ AC = $\frac{1}{2}$AB

$95 \times 96$
$95 \times 96{\text{ can also be written as}}\left( {100 - 5} \right)\left( {100 - 4} \right)$ We can observe that we can apply the identity $\left( {x + a} \right)\left( {x + b} \right) = {x^2} + \left( {a + b} \right)x + ab$

Here a = -5 and b = -4
$\left( {100 - 5} \right)\left( {100 - 4} \right) = {\left( {100} \right)^2} + \left[ {\left( { - 5} \right) + \left( { - 4} \right)} \right]\left( {100} \right) + \left( { - 5} \right) \times \left( { - 4} \right)$
 $= 10000 - 900 + 20$
= 9120 Therefore, we conclude that the value of the product $95 \times 96$ is 9120

You can check NCERT Solutions here : https://mycbseguide.com/ncert-solutions.html

Given: A ray OA.
Required: To construct an angle of 135⁰ at O.
Steps of construction :

1. Produce AO to A' to form OA'.
2. Taking O as centre and some radius, draw an arc of a circle, which intersects OA at a point B and OA' at a point B'.
3. Taking B as centre and with the same radius as before, draw an arc intersecting the previously drawn arc at a point C.
4. Taking C as centre and with the same radius as before, draw an arc intersecting the arc drawn in step 1, say at D.
   
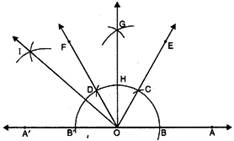
5. Draw the ray OE passing through C.
   Then $\angle$EOA = 60^o.
6. Draw the ray OF passing through D.
   Then $\angle$FOE = 60^o.
7. Taking C and D as centres and with the radius more than $1 \over2$CD, draw arcs to intersect each other, say at G.
8. Draw the ray OG intersecting the arc drawn in step 1 at H. This ray OG is the bisector of the angle FOE, i.e. $\angle$FOG = $\angle$EOG = $1 \over2$$\angle$FOE = $1 \over2$(60^o) = 30^o.
   Thus $\angle$GOA = $\angle$GOE + $\angle$EOA = 30^o + 60^o = 90^o
   $\angle$B'OH = 90^o
9. Taking B' and H as centres and with the radius more than $1 \over2$B'H, draw arcs to intersect each other, say at I.
10. Draw the ray OI. This ray OI is the bisector of the angle B'OG i.e. $\angle$B'OI = $\angle$GOI = $1 \over2$$\angle$B'OG = $1 \over2$(90^o) = 45^o
    $\angle$IOA = $\angle$IOG + $\angle$GOA
    = 45^o + 90^o = 135^o
    On measuring the $\angle$IOA by protractor, find that $\angle$IOA = 135^o.