CBSE > Class 09 > Mathematics | CBSE Board | Homework Help

Ask questions which are clear, concise and easy to understand.

Ask Question

Proof that sum of the angles of the triangle is 180

Posted by Faeem Saifi 5 years, 9 months ago

CBSE > Class 09 > Mathematics

3 answers

Harshit Garg 5 years, 9 months ago

Number of sides and subtract 2 and multipl by 180

0Thank You

Riddhi Kamde 5 years, 9 months ago

Consider a=x, b=x, c=x By using angle sum property a+b+c=180 i. e x+x+x=180 3x=180 X=180/3 X=60 hence, all angles of equilateral triangle are equal Therefore, a =60„ b=60 „c=60

0Thank You

Raja Shri 5 years, 9 months ago

60+60+60=180

0Thank You

ANSWER

Please give some rd questions

Posted by Kulsoom Rizvi 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

theorem proof

Posted by Owais Pathan 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Classify terminating decimalor non terminating. 1.6666

Posted by Shashi Rana 5 years, 9 months ago

CBSE > Class 09 > Mathematics

1 answers

Anurag Singh 5 years, 9 months ago

Non terminating

0Thank You

ANSWER

Two angles whose measures are a and b are such that 2a-3b=60° then 5b=?,if they form a linear pair

Posted by Naman Tomar 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

How to find √2 ?

Posted by Vanshi Ka 5 years, 9 months ago

CBSE > Class 09 > Mathematics

2 answers

Rajneesh Payal 5 years, 9 months ago

1.8

0Thank You

Raj Subba 5 years, 9 months ago

1.8

0Thank You

ANSWER

Kuch nhii

Posted by Rajat Khandelwal 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

25xcube +10xsquare+5x-10x-2

Posted by Pinankee Patel 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Factorise a^3-b^3-c^3

Posted by Swapna Tripathydash 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

2a5 -54a2

Posted by Simran Rajput 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

If a^3+b^3 +c^3=200 and ab+bc+ca=28 find the value of a+b+c

Posted by Shruthi Shirgaonkar 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Can you explain what is line segment,,, prependicular line Square Yesterday my exam Please explain it

Posted by Rakshit Goyal 5 years, 9 months ago

CBSE > Class 09 > Mathematics

3 answers

Rajneesh Payal 5 years, 9 months ago

Start point to ending point

1Thank You

Saabiq Kader 5 years, 9 months ago

Line segment means starting to ending point

1Thank You

Rishav Jha 5 years, 9 months ago

Line segment is a line which have both end points .. Perpendicular line when two line intersect each other and form an angle of 90 Square it is a polygon whose every side are equal and its diagnal are perpendicularly bisecting each other .. and interior angle is of 90

1Thank You

ANSWER

5+4

Posted by Sunny Kumar 5 years, 9 months ago

CBSE > Class 09 > Mathematics

6 answers

Rajneesh Payal 5 years, 9 months ago

0Thank You

Safu Mandal 5 years, 9 months ago

5+4=9

0Thank You

Vanshi Ka 5 years, 9 months ago

5+4=9

0Thank You

Akshat Shukla 5 years, 9 months ago

5+4=9

0Thank You

Riya Khari 5 years, 9 months ago

0Thank You

Ayush Raj 5 years, 9 months ago

0Thank You

ANSWER

The diagonals of a parrallelogram bisect each other

Posted by Pankaj Patel 5 years, 9 months ago

CBSE > Class 09 > Mathematics

3 answers

Rajneesh Payal 5 years, 9 months ago

Yes at 90 degree

0Thank You

Muskan Yadav 5 years, 9 months ago

Write one irrational number between 2 and '5

0Thank You

Akshat Shukla 5 years, 9 months ago

Yes

2Thank You

ANSWER

2.4ka question 5

Posted by Sonam Sonam 5 years, 9 months ago

CBSE > Class 09 > Mathematics

1 answers

Sonam Sonam 5 years, 9 months ago

2.4

4Thank You

ANSWER

What is à polynomial OR Defination of polynomial

Posted by Kanishka Dodwal 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

What is long division method

Posted by Akku Verma 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

In a triangle prove that the angle between the internal bisector of one base angle and the external bisector of other is equal to the half of the Vertical angle

Posted by Ankit Raj 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Set 1

Posted by Rohit Mahto 5 years, 9 months ago

CBSE > Class 09 > Mathematics

1 answers

Shreyas Kulal 5 years, 9 months ago

What is set 1 , and donot ask questions like this

1Thank You

ANSWER

Finde the value of k 7x+8y=k

Posted by Rashmi Gupta 5 years, 9 months ago

CBSE > Class 09 > Mathematics

4 answers

Kulsoom Rizvi 5 years, 9 months ago

What nonsense

0Thank You

Akshat Shukla 5 years, 9 months ago

Question is wrong... give the value or co-ordinate

0Thank You

Satyam Gulati 5 years, 9 months ago

7x+8y= k 15xy=k

0Thank You

Rohit Mahto 5 years, 9 months ago

0Thank You

ANSWER

Without actually calculating, use identities to find the value of (0.2)cube-(0.3) cube+(0.1)cube

Posted by Sourabh Shukla 5 years, 9 months ago

CBSE > Class 09 > Mathematics

2 answers

Sourabh Shukla 5 years, 9 months ago

Which identities using

1Thank You

Shreyas Kulal 5 years, 9 months ago

0.008-0.009+0.001=0

0Thank You

ANSWER

2ka cube

Posted by Technical Guruji 5 years, 9 months ago

CBSE > Class 09 > Mathematics

8 answers

Rajneesh Payal 5 years, 9 months ago

0Thank You

Vanshi Ka 5 years, 9 months ago

2³=2×2×2=8

0Thank You

Utlarsh Kumar 5 years, 9 months ago

2³ = 2×2×2= 8

0Thank You

Akshat Shukla 5 years, 9 months ago

0Thank You

Riya Khari 5 years, 9 months ago

0Thank You

Kanishka Dodwal 5 years, 9 months ago

0Thank You

Eva Kandoth 5 years, 9 months ago

2*2*2=8

0Thank You

Prachi Sharma 5 years, 9 months ago

2Thank You

ANSWER

Degree of 0 polynomial

Posted by Karan Singh 5 years, 9 months ago

CBSE > Class 09 > Mathematics

7 answers

Rajneesh Payal 5 years, 9 months ago

0Thank You

Shreyas Kulal 5 years, 9 months ago

Not defined

0Thank You

Akshat Shukla 5 years, 9 months ago

Not defined

0Thank You

Apoorv Kushwaha 5 years, 9 months ago

0Thank You

Satyam Gulati 5 years, 9 months ago

Not defined

0Thank You

Babu Jaya 5 years, 9 months ago

0Thank You

Harshit Garg 5 years, 9 months ago

0Thank You

ANSWER

24x^2-41x+12

Posted by Shivam Sharma 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

the base of an isosceles triangle is 4cm and its area is 16cm^2. the side of the triangle is k cm.find the value of k

Posted by Dhriti Sanghvi 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

Hii

Posted by Hassan Zemo 5 years, 9 months ago

CBSE > Class 09 > Mathematics

1 answers

Akshat Shukla 5 years, 9 months ago

Hello

0Thank You

ANSWER

2_5

Posted by Neha Ahuja 5 years, 9 months ago

CBSE > Class 09 > Mathematics

0 answers

ANSWER

If x = (0) and Y = (K) is a solution of the equation 5 x - 3y = 3 find the value of k

Posted by Meenu Gautam 5 years, 9 months ago

CBSE > Class 09 > Mathematics

3 answers

Vanshi Ka 5 years, 9 months ago

5(0)-3(k)=3 -3(k)=3 K=3÷(-3) k=-1

1Thank You

Pinankee Patel 5 years, 9 months ago

5×0-3×k=3 =0-3k=3 =-3k=3 k=-1

1Thank You

Sujal Agrawal 5 years, 9 months ago

-1=K

1Thank You

ANSWER

If x is equal to zero and Y is equal to K is a solution of the equation 5 x minus 3 Y is equal to 3 find the value of k .

Posted by Meenu Gautam 5 years, 9 months ago

CBSE > Class 09 > Mathematics

1 answers

Sujal Agrawal 5 years, 9 months ago

-1

1Thank You

ANSWER

If the angle bisectors of one angle bisects the other side. Prove that it is a isosceles triangle.

Posted by Kushal Shah 5 years, 9 months ago

CBSE > Class 09 > Mathematics

1 answers

Sia ? 5 years, 9 months ago

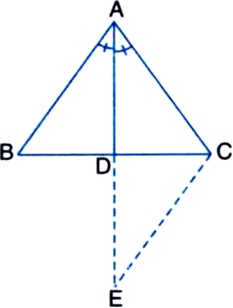
Given: In a $\triangle$ ABC, consider AD be the bisector of $\angle$ A which meets BC in D such that BD = DC.
To Prove: $\triangle$ ABC is an isosceles triangle i.e. AB = AC.
Construction: Produce AD to E such that AD=DE and then join CE.
Proof: In $\Delta ABD \;and \;\Delta ECD,$ we have

BD = CD [Given]

AD = ED [By construction]

and $\angle$ ADB = $\angle$ EDC [Vertically opposite angles]

Therefore, $\Delta ABD=\Delta ECD$ [SAS congruence criterion]

So, AB = EC [Corresponding parts of congruent triangles are congruent] ---- (i)
and $\angle$ BAD = $\angle$ CED [Corresponding parts of congruent triangles are congruent] ---- (ii)
Also, $\angle$ BAD = $\angle$ CAD [Given] ---- (iii)
Therefore, From (ii) and (iii) we get
$\angle$ CAD = $\angle$ CED
So, AC = EC [Sides opposite to angles are equal]....... (iv)
From (i) and (iv), we get
AB = AC
$\therefore$ $\triangle$ ABC is an isosceles triangle.

0Thank You

ANSWER

Page 343 of 1011

myCBSEguide

myCBSEguide

Test Generator

CUET Mock Tests

Download myCBSEguide App

All Courses

Other Websites

CBSE Courses

Resources

Corporate

NCERT Solutions

CBSE Sample Papers