
myCBSEguide App
Download the app to get CBSE Sample Papers 2023-24, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowNCERT Solutions class 12 Maths Miscellaneous Class 12 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
Download NCERT solutions for Applications of Derivatives as PDF.

NCERT Solutions for Class 12 Maths Application of Derivatives
1. Using differentials, find the approximate value of each of the following:
(a) 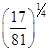
(b) 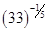
Ans. (a) 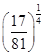
Let 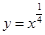 ……….(i)
……….(i)

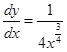

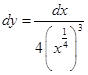
=  ……….(ii)
……….(ii)
Changing  to
to  and
and 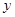 to
to 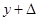 in eq. (i), we have
in eq. (i), we have
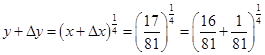 ……….(iii)
……….(iii)
Here 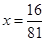 and
and 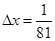

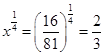
From eq. (iii),
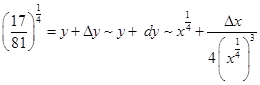


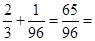 0.677
0.677
(b) 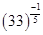
Let 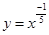 ……….(i)
……….(i)

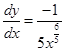

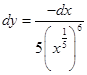
=  ……….(ii)
……….(ii)
Changing  to
to  and
and 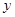 to
to 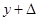 in eq. (i), we have
in eq. (i), we have
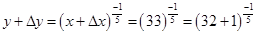 …….(iii)
…….(iii)
Here 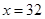 and
and 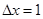

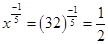
From eq. (iii),
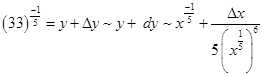


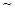
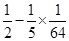
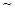
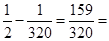 0.497
0.497
2. Show that the function given by  has maximum value at
has maximum value at 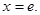
Ans. Here 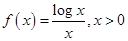 ……….(i)
……….(i)

 ……..(ii)
……..(ii)
And 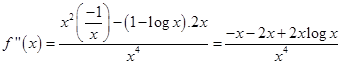
= 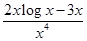

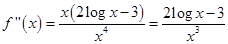 ……….(iii)
……….(iii)
Now 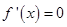

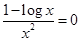

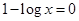

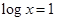

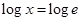

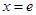
 From eq. (iii),
From eq. (iii),
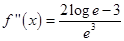 =
= 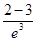 =
= 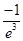 < 0
< 0

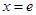 is a point of local maxima and maximum value of
is a point of local maxima and maximum value of 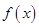 is at
is at 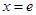 .
.
NCERT Solutions class 12 Maths Miscellaneous
3. The two equal sides of an isosceles triangle with fixed base 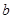 are decreasing at the rate of 3 cm per second. How fast is the area decreasing when the two equal sides are equal to the base?
are decreasing at the rate of 3 cm per second. How fast is the area decreasing when the two equal sides are equal to the base?
Ans. Let BC = 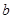 be the fixed base and AB = AC =
be the fixed base and AB = AC =  be
be
the two equal sides of given isosceles triangle.
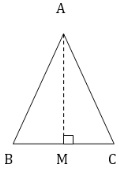
Since 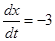 cm/s ……….(i)
cm/s ……….(i)
Area of 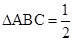 x BC x AM
x BC x AM
= 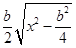
= 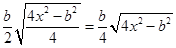

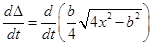
= 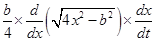 [By chain rule]
[By chain rule]

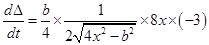
= 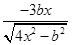 cm2/s
cm2/s
Now when 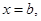
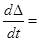
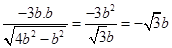 cm2/s
cm2/s
Therefore, the area is decreasing at the rate of 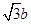 cm2/s.
cm2/s.
NCERT Solutions class 12 Maths Miscellaneous
4. Find the equation of the normal to the curve 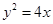 at the point (1, 2).
at the point (1, 2).
Ans. Equation of the curve is 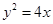 ……….(i)
……….(i)



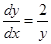
 Slope of the tangent to the curve at the point (1, 2) to curve (i) is
Slope of the tangent to the curve at the point (1, 2) to curve (i) is 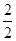 =1
=1
 Slope of the normal to the curve at (1, 2) is
Slope of the normal to the curve at (1, 2) is 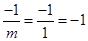
 Equation of the normal to the curve (i) at (1, 2) is
Equation of the normal to the curve (i) at (1, 2) is 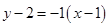

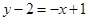

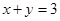
NCERT Solutions class 12 Maths Miscellaneous
5. Show that the normal at any point  to the curve
to the curve 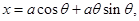
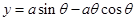 is at a constant distance from the origin.
is at a constant distance from the origin.
Ans. The parametric equations of the curve are
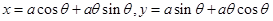

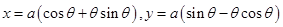

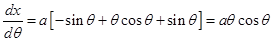
And 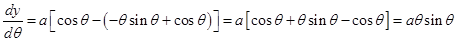
 Slope of tangent at point
Slope of tangent at point 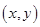
= 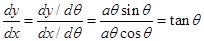
 Slope of normal at any point
Slope of normal at any point 
= 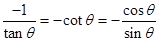
And Equation of normal at any point 
i.e., at 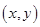 =
= 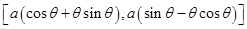 is
is 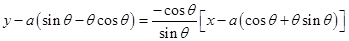

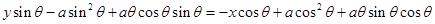



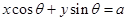

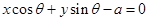
 Distance of normal from origin (0, 0)
Distance of normal from origin (0, 0)
= 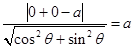 which is a constant.
which is a constant.
NCERT Solutions class 12 Maths Miscellaneous
6. Find the intervals in which the function 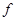 given by
given by 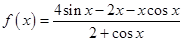 is (i) increasing (ii) decreasing.
is (i) increasing (ii) decreasing.
Ans. Given: 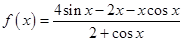
= 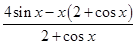
= 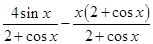
= 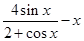

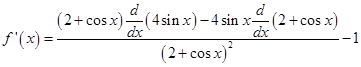

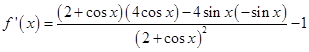
= 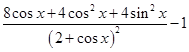

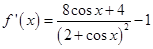
= 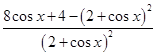
= 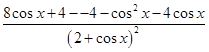

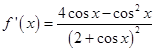
= 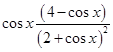 ……….(i)
……….(i)
Now 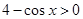 for all real
for all real  as
as 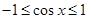 . Also
. Also 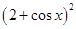 > 0
> 0
(i) 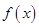 is increasing if
is increasing if 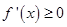 , i.e., from eq. (i),
, i.e., from eq. (i), 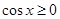

 lies in I and IV quadrants, i.e.,
lies in I and IV quadrants, i.e., 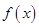 is increasing for
is increasing for 
and 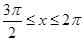
and (ii) 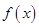 is decreasing if
is decreasing if 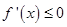 , i.e., from eq. (i),
, i.e., from eq. (i), 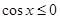

 lies in II and III quadrants, i.e.,
lies in II and III quadrants, i.e., 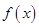 is decreasing for
is decreasing for 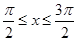
7. Find the intervals in which the function 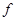 given by
given by 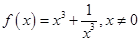 is (i) increasing (ii) decreasing.
is (i) increasing (ii) decreasing.
Ans. (i) Given: 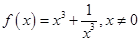



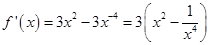
= 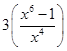 =
= 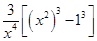

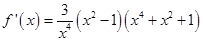
= 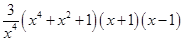 ……….(i)
……….(i)
Now 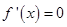

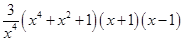 = 0
= 0

 = 0
= 0
Here,  is positive for all real
is positive for all real 
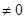

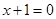 or
or 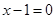 [Turning points]
[Turning points]
Therefore, 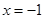 or
or 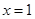 divide the real line into three sub intervals
divide the real line into three sub intervals  and
and 
For 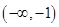 , from eq. (i) at
, from eq. (i) at 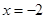 (say),
(say),

Therefore, 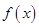 is increasing at
is increasing at 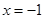
For 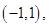 from eq. (i) at
from eq. (i) at 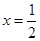 (say)
(say)
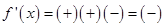
Therefore, 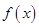 is decreasing at
is decreasing at 
For 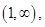 from eq. (i) at
from eq. (i) at 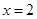 (say),
(say),
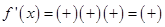
Therefore, 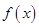 is increasing at
is increasing at 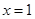
Therefore, 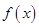 is (i) an increasing function for
is (i) an increasing function for 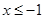 and for
and for 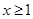 and (ii) decreasing function for
and (ii) decreasing function for 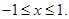
NCERT Solutions class 12 Maths Miscellaneous
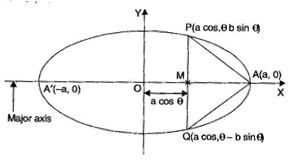
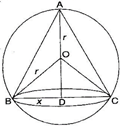
8. Find the maximum area of an isosceles triangle inscribed in the ellipse 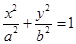 with its vertex at one end of the major axis.
with its vertex at one end of the major axis.
Ans. Equation of the ellipse is 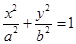 ……….(i)
……….(i)
Comparing eq. (i) with  we have
we have 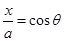 and
and 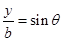

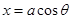 and
and 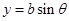
 Any point on ellipse is P
Any point on ellipse is P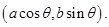
Draw PM perpendicular to 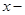 axis and produce it to meet the ellipse in the point Q.
axis and produce it to meet the ellipse in the point Q.
 OM =
OM = 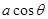 and PM =
and PM = 
We know that the ellipse (i) is symmetrical about 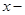 axis, therefore, PM = QM and hence triangle APQ is isosceles.
axis, therefore, PM = QM and hence triangle APQ is isosceles.
Area of 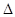 APQ
APQ 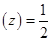 x Base x Height
x Base x Height
= 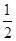 PQ.AM =
PQ.AM = 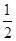 . 2PM.AM = PM (OA – OM)
. 2PM.AM = PM (OA – OM)

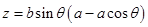
= 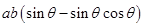

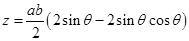
= 

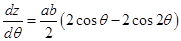
= 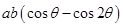

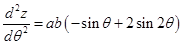
Now 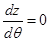

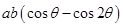 = 0
= 0

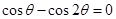

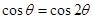

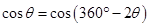

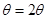 or
or 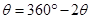
i.e., 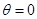 or
or 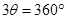


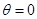 is impossible
is impossible


At  ,
, 
= 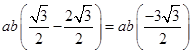 [Negative]
[Negative]

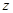 is maximum at
is maximum at 
 From eq. (i), Maximum area
From eq. (i), Maximum area
= 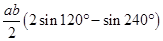
= 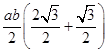
= 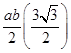 =
= 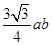
NCERT Solutions class 12 Maths Miscellaneous
9. A tank with rectangular base and rectangular sides, open at the top is to be constructed so that its depth is 2 m and volume is 8 m3. If building of tank costs ` 70 per sq. meter for the base and ` 45 per square meter for sides. What is the cost of least expensive tank?
Ans. Given: Depth of tank = 2 m
Let  m be the length and
m be the length and 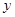 m be the breadth of the base of the tank.
m be the breadth of the base of the tank.
Volume of tank = 8 cubic meters

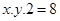

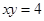

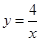
Cost of building the base of the tank at the rate of ` 70 per sq. meter is 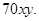
And cost of building the four walls of the tank at the rate of ` 45 per sq. meter is
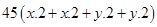
= `
Let 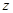 be the total cost of building the tank.
be the total cost of building the tank.
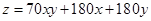

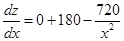 and
and 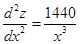
Now 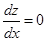

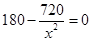

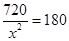



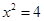

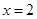 [length cannot be negative]
[length cannot be negative]
At 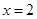
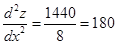 [Positive]
[Positive]

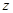 is minimum at
is minimum at 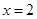 .
.
 Minimum cost =
Minimum cost = 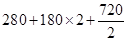
= 280 + 360 + 360 = ` 1000
NCERT Solutions class 12 Maths Miscellaneous
10. The sum of the perimeter of a circle and square is 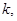 where
where 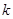 is some constant. Prove that the sum of their areas is least when the side of square is double the radius of the circle.
is some constant. Prove that the sum of their areas is least when the side of square is double the radius of the circle.
Ans. Let  be the radius of the circle and
be the radius of the circle and 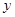 be the side of square.
be the side of square.
According to question, Perimeter of circle + Perimeter of square = 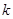

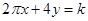

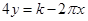

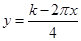 ……….(i)
……….(i)
Let 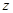 be the sum of areas of circle and square.
be the sum of areas of circle and square.



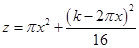 [From eq. (i)]
[From eq. (i)]

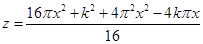
= 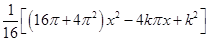

 and
and 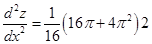
Now 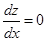

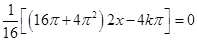

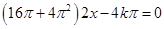




At 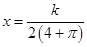
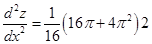 [Positive]
[Positive]

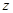 is minimum when
is minimum when 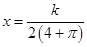
 From eq. (i),
From eq. (i),
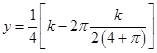
= 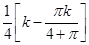
= 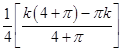

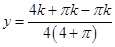
= 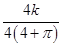
= 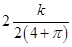 =
= 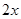
Therefore, sum of areas is minimum when side of the square is double the radius of the circle.
NCERT Solutions class 12 Maths Miscellaneous
11. A window is in the form of a rectangle surmounted by a semicircular opening. The total perimeter of the window is 10 m. Find the dimensions of the window to admit maximum light through the whole opening.
Ans. Let  m be the radius of the semi-circular opening of the window. Then one side of rectangle part of window is
m be the radius of the semi-circular opening of the window. Then one side of rectangle part of window is 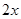 and
and 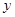 m be the other side of rectangle.
m be the other side of rectangle.
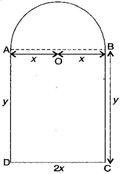
 Perimeter of window
Perimeter of window
= Semi-circular arc AB + Length (AD + DC + BC)

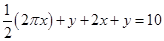

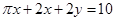

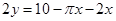

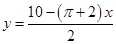 ……….(i)
……….(i)
Area of window 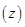
= Area of semi-circle + Area of rectangle
= 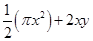

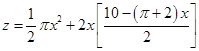
= 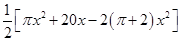

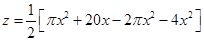
= 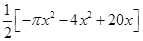

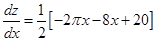 and
and 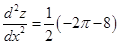 =
= 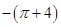
Now 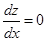

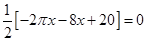

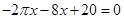



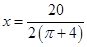

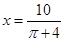
At 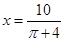
 [Negative]
[Negative]

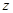 is maximum at
is maximum at 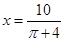
 From eq. (i),
From eq. (i), 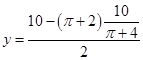
= 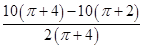


= 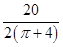 =
= 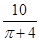 m
m
Therefore, Length of rectangle =  m and Width of rectangle =
m and Width of rectangle = 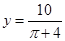 m
m
And Radius of semi-circle = 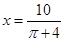 m
m
NCERT Solutions class 12 Maths Miscellaneous
12. A point on the hypotenuse of a triangle is at distances 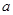 and
and 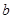 from the sides of the triangle. Show that the maximum length of the hypotenuse is
from the sides of the triangle. Show that the maximum length of the hypotenuse is 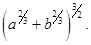
Ans. Let P be a point on the hypotenuse AC of a right triangle ABC such that PL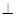 AB =
AB = 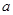 and PM
and PM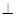 BC =
BC = 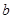 and let
and let  BAC =
BAC =  MPC =
MPC =  , then in right angled
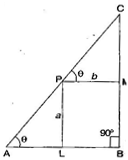
, then in right angled 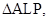

 AP = PL
AP = PL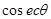 =
= 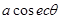
And in right angled 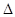 PMC,
PMC, 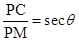
 PM = PM
PM = PM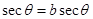
Let AC = 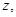 then
then
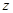 = AP + PC =
= AP + PC = 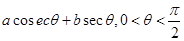 ……….(i)
……….(i)

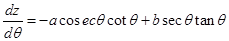
Now 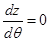

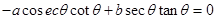

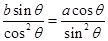



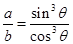

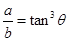

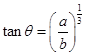 ……….(ii)
……….(ii)
And 



 [
[ and
and  is +ve as
is +ve as 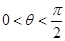 )
)

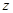 is minimum when
is minimum when 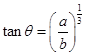

 =
= 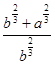

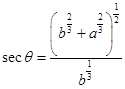
Also 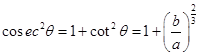
= 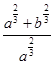

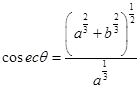
Putting these values in eq. (i),
Minimum length of hypotenuse = 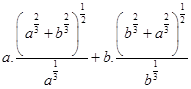
=  =
= 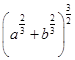
NCERT Solutions class 12 Maths Miscellaneous
13. Find the points at which the function 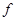 given by
given by 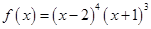 has:
has:
(i) local maxima
(ii) local minima
(iii) point of inflexion.
Ans. Given: 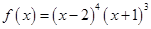 ……….(i)
……….(i)

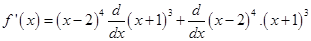
= 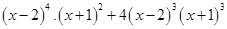
= 
= 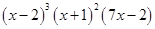
Now 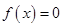

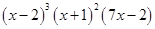 = 0
= 0

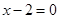 or
or 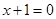 or
or 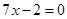

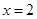 or
or 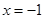 or
or 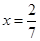
Now, for values of  close to
close to 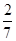 and to the left of
and to the left of  . Also for values of
. Also for values of  close to
close to 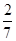 and to the right of
and to the right of 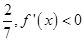 .
.
Therefore, 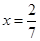 is the point of local maxima.
is the point of local maxima.
Now, for values of  close to 2 and to the left of
close to 2 and to the left of 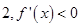 . Also for values of
. Also for values of  close to 2 and to the right of
close to 2 and to the right of 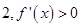 .
.
Therefore, 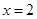 is the point of local minima.
is the point of local minima.
Now as the values of  varies through
varies through  does not change its sign. Therefore,
does not change its sign. Therefore, 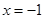 is the point of inflexion.
is the point of inflexion.
NCERT Solutions class 12 Maths Miscellaneous
14. Find the absolute maximum and minimum values of the function 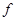 given by
given by 
Ans. Given: 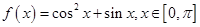 ……….(i)
……….(i)

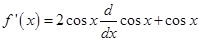
= 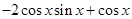 =
= 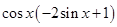
Now 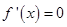

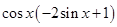 = 0
= 0

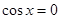 or
or 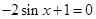

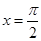 or
or 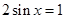

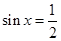

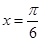 [Turning points]
[Turning points]
Now 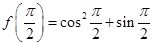
= 0 + 1 = 1
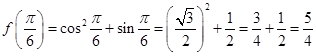
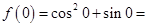 1 + 0 = 1
1 + 0 = 1
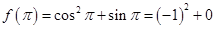 = 1
= 1
Therefore, absolute maximum is 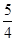 and absolute minimum is 1.
and absolute minimum is 1.
NCERT Solutions class 12 Maths Miscellaneous
15. Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius 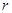 is
is 
Ans. Let  be the radius of base of cone and
be the radius of base of cone and 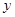 be the height of the cone inscribed in a sphere of radius
be the height of the cone inscribed in a sphere of radius 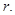
 OD = AD – AO =
OD = AD – AO = 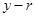
In right angled triangle OBD,
OD2 + BD2 = OB2



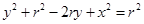

 ……….(i)
……….(i)
Volume of cone (V) = 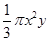 =
= 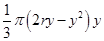 [From eq. (i)]
[From eq. (i)]
 V =
V = 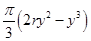

 and
and 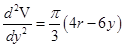
Now 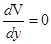

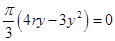



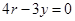

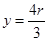
At 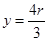

= 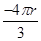 [Negative]
[Negative]
 Volume is maximum at
Volume is maximum at 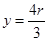
NCERT Solutions class 12 Maths Miscellaneous
16. Let 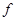 be a function defined on
be a function defined on  such that
such that 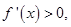 for all
for all 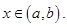 Then prove that
Then prove that 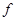 is an increasing function on
is an increasing function on 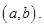
Ans. Let I be the interval 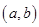
Given: 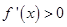 for all
for all  in an interval I. Let
in an interval I. Let 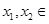 I with
I with 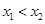
By Lagrange’s Mean Value Theorem, we have,
 where
where 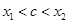

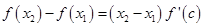 where
where 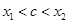
Now 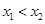

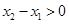 ……….(i)
……….(i)
Also, 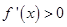 for all
for all  in an interval I
in an interval I

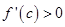
 From eq. (i),
From eq. (i), 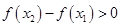

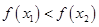
Thus, for every pair of points 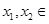 I with
I with 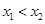

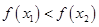
Therefore, 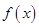 is strictly increasing in I.
is strictly increasing in I.
NCERT Solutions class 12 Maths Miscellaneous
17. Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is 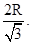 Also find the maximum volume.
Also find the maximum volume.
Ans. Let  be the radius and
be the radius and 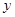 be the height of the cylinder inscribed in a sphere having centre O and radius R.
be the height of the cylinder inscribed in a sphere having centre O and radius R. 
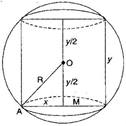
In right triangle OAM, AM2 + OM2 = OA2

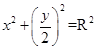

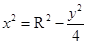 ……….(i)
……….(i)
Volume of cylinder (V) = 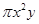 ……….(ii)
……….(ii)
 V =
V = 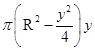
=  ……….(iii)
……….(iii)

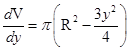 and
and 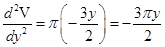
Now 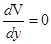

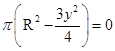

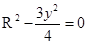

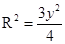

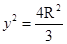

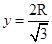
At 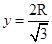
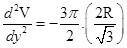
= 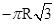 [Negative]
[Negative]
 V is maximum at
V is maximum at 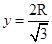
 From eq. (iii),
From eq. (iii),
Maximum value of cylinder = 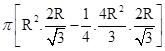
= 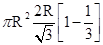
= 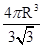
NCERT Solutions class 12 Maths Miscellaneous
18. Show that the height of the cylinder of greatest volume which can be inscribed in a right circular cone of height 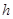 and having semi-vertical angle
and having semi-vertical angle 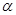 is one-third that of the cone and the greatest volume of the cylinder is
is one-third that of the cone and the greatest volume of the cylinder is 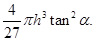
Ans. Let 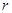 be the radius of the right circular cone of height
be the radius of the right circular cone of height  Let the radius of the inscribed cylinder be
Let the radius of the inscribed cylinder be  and height
and height 
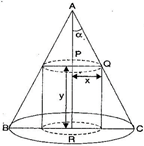
In similar triangles APQ and ARC, 

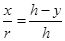



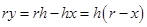

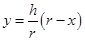
Volume of cylinder (V) = 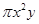 ……….(ii)
……….(ii)
 V =
V = 
= 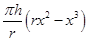 ……….(iii)
……….(iii)

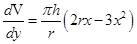 and
and 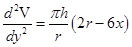
Now 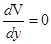

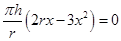

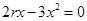



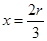
At 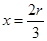
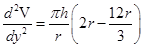
= 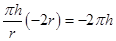 [Negative]
[Negative]
 V is maximum at
V is maximum at 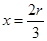
 From eq. (iii),
From eq. (iii),
Maximum value of cylinder = 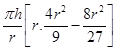
= 
= 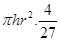
= 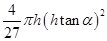
= 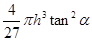
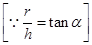
Choose the correct answer in the Exercises 19 to 24:
NCERT Solutions class 12 Maths Miscellaneous
19. A cylindrical tank of radius 10 m is being filled with wheat at the rate of 314 cubic meter per hour. Then the depth of wheat is increasing at the rate of:
(A) 1 m/h
(B) 0.1 m/h
(C) 1.1 m/h
(D) 0.5 m/h
Ans. Let 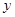 be the depth of the wheat in the cylindrical tank of radius 10 m at time
be the depth of the wheat in the cylindrical tank of radius 10 m at time 
 V = Volume of wheat in cylindrical tank at time
V = Volume of wheat in cylindrical tank at time  cu. m
cu. m
It is given that 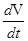 = 314 cu. m/hr
= 314 cu. m/hr

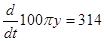

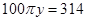

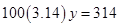

 1 m/h
1 m/h
Therefore, option (A) is correct.
NCERT Solutions class 12 Maths Miscellaneous
20. The slope of the tangent to the curve 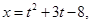
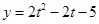 at the point
at the point 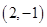 is:
is:
(A) 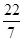
(B) 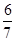
(C) 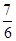
(D) 
Ans. Equation of the curves are 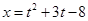 …..(i) and
…..(i) and 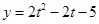 …..(ii)
…..(ii)

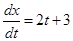 and
and 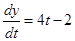
 Slope of the tangent to the given curve at point
Slope of the tangent to the given curve at point 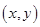 =
= 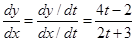 …..(iii)
…..(iii)
At the given point 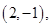
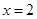 and
and 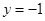
At 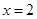 , from eq. (i),
, from eq. (i), 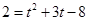

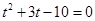

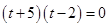

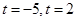
At 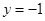 , from eq. (ii),
, from eq. (ii), 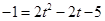

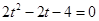

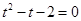



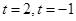
Here, common value of 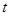 in the two sets of values is
in the two sets of values is 
 From eq. (iii),
From eq. (iii),
Slope of the tangent to the given curve at point 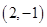 =
= 
Therefore, option (B) is correct.
NCERT Solutions class 12 Maths Miscellaneous
21. The line  is a tangent to the curve
is a tangent to the curve 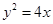 if the value of
if the value of 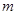 is:
is:
(A) 1
(B) 2
(C) 3
(D) 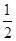
Ans. Equation of the curve is 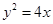 ……….(i)
……….(i)

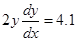

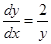
 Slope of the tangent to the given curve at point
Slope of the tangent to the given curve at point 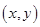 =
= 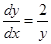

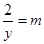

 ……….(ii)
……….(ii)
Now 

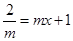

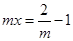

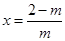 …..(iii)
…..(iii)
Putting the values of  and
and 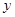 in eq. (i),
in eq. (i), 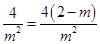

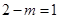

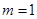
Therefore, option (A) is correct.
NCERT Solutions class 12 Maths Miscellaneous
22. The normal at the point (1, 1) on the curve 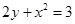 is:
is:
(A) 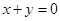
(B) 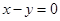
(C) 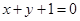
(D) 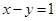
Ans. Equation of the given curve is 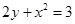 ……….(i)
……….(i)

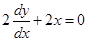

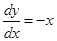
 Slope of the tangent to the given curve at point (1, 1) =
Slope of the tangent to the given curve at point (1, 1) = 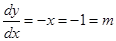 (say)
(say)
 Slope of the normal =
Slope of the normal = 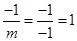
 Equation of the normal at (1, 1) is
Equation of the normal at (1, 1) is 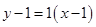

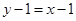

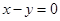
Therefore, option (B) is correct.
NCERT Solutions class 12 Maths Miscellaneous
23. The normal to the curve 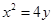 passing through (1, 2) is:
passing through (1, 2) is:
(A) 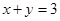
(B) 
(C) 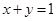
(D) 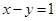
Ans. Equation of the curve is 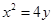 ………..(i)
………..(i)

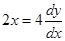

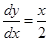
 Slope of the normal at
Slope of the normal at 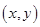 =
= 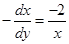 ……….(ii)
……….(ii)
Again slope of normal at given point (1, 2) = 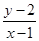 ……….(iii)
……….(iii)
From eq. (ii) and (iii), we have 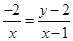

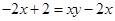

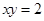

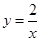
 From eq. (i),
From eq. (i), 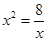

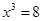

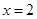

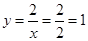
Now, at point (2, 1), slope of the normal from eq. (ii) = 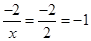
 Equation of the normal is
Equation of the normal is 

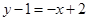

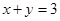
Therefore, option (A) is correct.
NCERT Solutions class 12 Maths Miscellaneous
24. The points on the curve 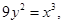 where the normal to the curve make equal intercepts with axes are:
where the normal to the curve make equal intercepts with axes are:
(A) 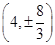
(B) 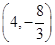
(C) 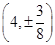
(D) 
Ans. Equation of the curve is 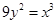 ……….(i)
……….(i)



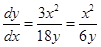
 Slope of the tangent to curve (i) at any point
Slope of the tangent to curve (i) at any point 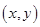 =
= 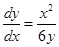
 Slope of the normal = negative reciprocal =
Slope of the normal = negative reciprocal = 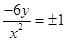
[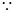 Slopes of lines making equal intercepts on the axes are
Slopes of lines making equal intercepts on the axes are 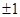 ]
]

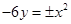
Taking positive sign, 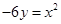

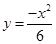 ……….(ii)
……….(ii)
From eq. (i) and (ii), we have 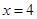 and
and 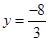
Taking positive sign, 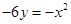

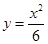 ……….(ii)
……….(ii)
From eq. (i) and (ii), we have 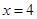 and
and 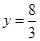
 Required points are
Required points are 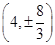
Therefore, option (A) is correct.
NCERT Solutions class 12 Maths Miscellaneous
NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes text book solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 20 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide
CBSE App for Class 12
To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
myCBSEguide
Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes
Install Now