
myCBSEguide App
Download the app to get CBSE Sample Papers 2023-24, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowNCERT Solutions class 12 Maths Exercise 6.5 Class 12 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
Download NCERT solutions for Applications of Derivatives as PDF.

NCERT Solutions for Class 12 Maths Application of Derivatives
1. Find the maximum and minimum values, if any, of the following functions given by:
(i) 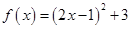
(ii) 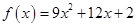
(iii) 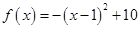
(iv) 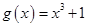
Ans. (i) Given: 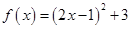
Since 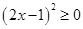 for all
for all  R
R
Adding 3 both sides, 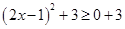

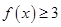
Therefore, the minimum value of 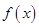 is 3 when
is 3 when 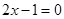 , i.e.,
, i.e., 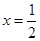
This function does not have a maximum value.
(ii) Given: 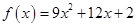

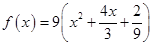

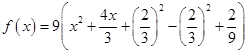
= 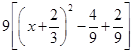

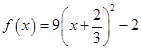 ……….(i)
……….(i)
Since  for all
for all 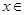 R
R
Subtracting 2 from both sides, 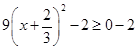


Therefore, minimum value of 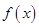 is
is  and is obtained when
and is obtained when 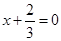 , i.e.,
, i.e., 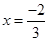
And this function does not have a maximum value.
(iii) Given: 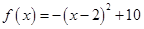 ……….(i)
……….(i)
Since  for all
for all 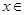 R
R
Multiplying both sides by 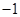 and adding 10 both sides,
and adding 10 both sides,


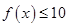 [Using eq. (i)]
[Using eq. (i)]
Therefore, maximum value of 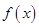 is 10 which is obtained when
is 10 which is obtained when 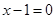 i.e.,
i.e., 
And therefore, minimum value of 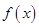 does not exist.
does not exist.
(iv) Given: 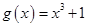
As 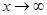

As 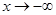

Therefore, maximum value and minimum value of 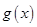 do not exist.
do not exist.
NCERT Solutions class 12 Maths Exercise 6.5
2. Find the maximum and minimum values, if any, of the following functions given by:
(i) 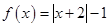
(ii) 
(iii) 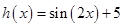
(iv) 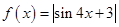
(v) 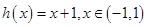
Ans. (i) Given: 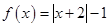 ……….(i)
……….(i)
Since 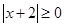 for all
for all 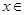 R
R
Subtracting 1 from both sides, 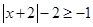

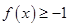
Therefore, minimum value of 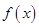 is
is 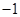 which is obtained when
which is obtained when 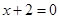 i.e.,
i.e., 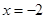
From eq. (i), maximum value of 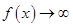 hence it does not exist.
hence it does not exist.
(ii) Given: 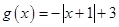
Since 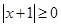 for all
for all 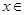 R
R
Multiplying by 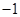 both sides and adding 3 both sides,
both sides and adding 3 both sides,
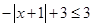


Therefore, maximum value of 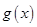 is
is 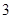 which is obtained when
which is obtained when 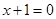 i.e.,
i.e., 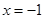
From eq. (i), minimum value of  hence it does not exist.
hence it does not exist.
(iii) Given: 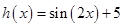 ……….(i)
……….(i)
Since 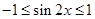 for all
for all 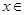 R
R
Adding 5 to all sides, 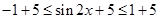

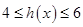
Therefore, minimum value of 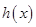 is 4 and maximum value is 6.
is 4 and maximum value is 6.
(iv) Given: 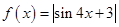
Since 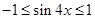 for all
for all 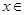 R
R
Adding 3 to all sides, 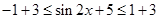

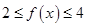
Therefore, minimum value of 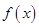 is 2 and maximum value is 4.
is 2 and maximum value is 4.
(v) Given: 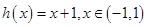 ……….(i)
……….(i)
Since 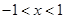
Adding 1 to both sides, 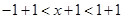

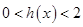
Therefore, neither minimum value not maximum value of 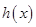 exists.
exists.
NCERT Solutions class 12 Maths Exercise 6.5
3. Find the local maxima and local minima, if any, of the following functions. Find also the local maximum and the local minimum values, as the case may be:
(i) 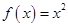
(ii) 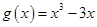
(iii) 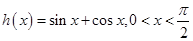
(iv) 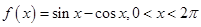
(v) 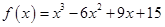
(vi) 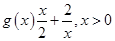
(vii) 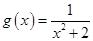
(viii) 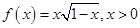
Ans. (i) Given: 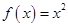

 and
and 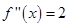
Now 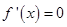

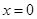 [Turning point]
[Turning point]
Again, when 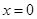 ,
, 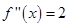 [Positive]
[Positive]
Therefore, 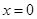 is a point of local minima and local minimum value =
is a point of local minima and local minimum value = 
(ii) Given: 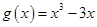

 and
and 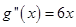
Now 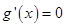

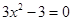

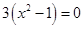

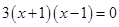

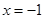 or
or  [Turning points]
[Turning points]
Again, when 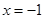 ,
,
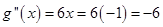 [Negative]
[Negative]

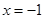 is a point of local maxima and local maximum value
is a point of local maxima and local maximum value 
And when 
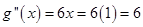 [Positive]
[Positive]

 is a point of local minima and local minimum value
is a point of local minima and local minimum value 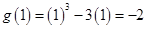
(iii) Given: 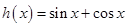
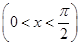 ……….(i)
……….(i)

 and
and 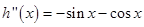
Now 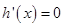



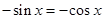

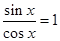

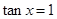 [Positive]
[Positive]

 can have values in both I and III quadrant.
can have values in both I and III quadrant.
But, 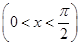 therefore,
therefore,  is only in I quadrant.
is only in I quadrant.

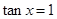 =
= 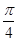 [Turning point]
[Turning point]
At 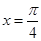
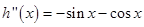

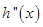 =
= 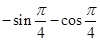
= 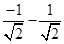 =
=  =
= 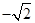 [Negative]
[Negative]

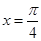 is a point of local maxima and local maximum value
is a point of local maxima and local maximum value
= 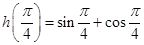
= 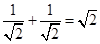
(iv) Given: 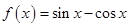
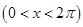 ……….(i)
……….(i)

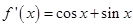 and
and 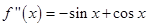
Now 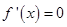

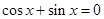

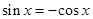

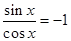

 [Negative]
[Negative]

 can have values in both II and IV quadrant.
can have values in both II and IV quadrant.

 =
= 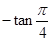
= 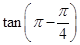 or
or 

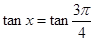 or
or 

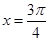 and
and 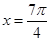 [Turning point]
[Turning point]
At 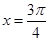
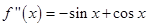 =
= 

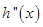 =
= 
= 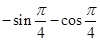
= 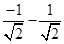
=  =
= 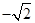 [Negative]
[Negative]

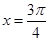 is a point of local maxima and local maximum value =
is a point of local maxima and local maximum value = 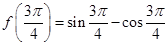
= 
= 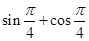 =
= 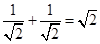
At 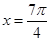
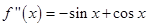
= 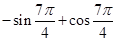

 =
= 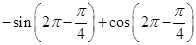
= 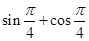
= 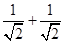
= 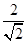 =
= 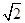 [Positive]
[Positive]

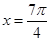 is a point of local maxima and local maximum value =
is a point of local maxima and local maximum value = 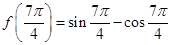
= 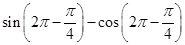
= 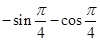 =
= 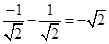
(v) Given: 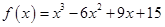

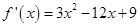 and
and 
Now 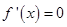

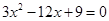





 or
or 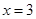 [Turning points]
[Turning points]
At 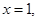
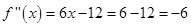 [Negative]
[Negative]

 is a point of local maxima and local maximum value is
is a point of local maxima and local maximum value is 
At 
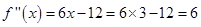 [Positive]
[Positive]

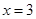 is a point of local minima and local minimum value is
is a point of local minima and local minimum value is 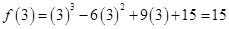
(vi) Given: 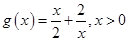

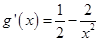
= 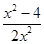
= 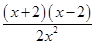 and
and 
Now 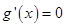

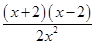 = 0
= 0

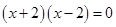

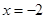 or
or 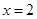
But  therefore
therefore 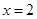 is only the turning point.
is only the turning point.

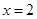 is a point of local minima and local minimum value is
is a point of local minima and local minimum value is 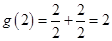
(vii) Given: 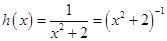

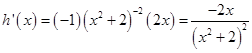 and
and
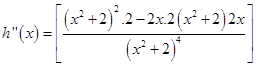
= 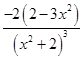
Now 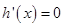

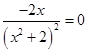

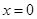 [Turning point]
[Turning point]
At 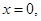
 [Negative]
[Negative]

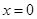 is a point of local maxima and local maximum value is
is a point of local maxima and local maximum value is 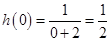
(viii) Given: 


= 
= 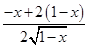 =
= 
And 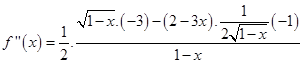
= 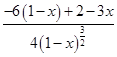
= 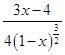
Now 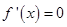

 = 0
= 0

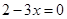

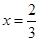

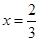 is a point of local maxima and local maximum value is
is a point of local maxima and local maximum value is 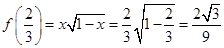
Again 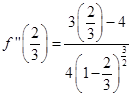
= 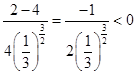

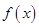 has local maximum value at
has local maximum value at 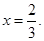
NCERT Solutions class 12 Maths Exercise 6.5
4. Prove that the following functions do not have maxima or minima:
(i) 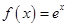
(ii) 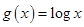
(iii) 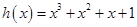
Ans. (i) Given: 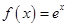

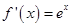
Now 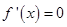

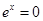
But this gives no real value of 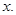 Therefore, there is no turning point.
Therefore, there is no turning point.

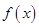 does not have maxima or minima.
does not have maxima or minima.
(ii) Given: 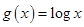

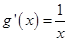
Now 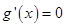

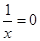
 1 = 0
1 = 0
But this is not possible. Therefore, there is no turning point.

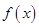 does not have maxima or minima.
does not have maxima or minima.
(iii) Given: 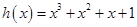

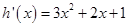
Now 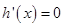

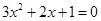

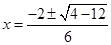
= 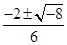
= 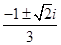
Here, values of  are imaginary.
are imaginary.

 does not have maxima or minima.
does not have maxima or minima.
5. Find the absolute maximum value and the absolute minimum value of the following functions in the given intervals:
(i) 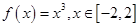
(ii) 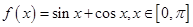
(iii) 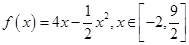
(iv) 
Ans. (i) Given: 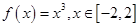

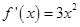
Now 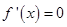

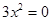

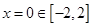
At 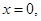
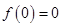
At 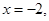
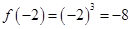
At 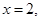
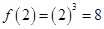
Therefore, absolute minimum value of 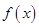 is
is  and absolute maximum value is 8.
and absolute maximum value is 8.
(ii) Given: 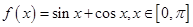


Now 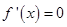



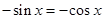

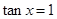 [Positive]
[Positive]

 is in I quadrant. [
is in I quadrant. [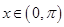 ]
]

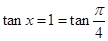

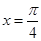

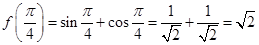
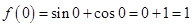
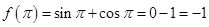
Therefore, absolute minimum value is 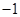 and absolute maximum value is 1.
and absolute maximum value is 1.
(iii) Given: 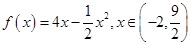

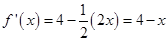
Now 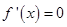

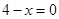

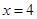
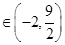
At 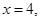

At 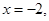
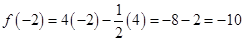
At 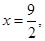
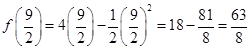
Therefore, absolute minimum value is 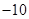 and absolute maximum value is 8.
and absolute maximum value is 8.
(iv) Given: 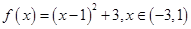


Now 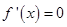

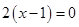

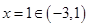
At 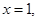
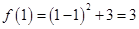
At 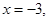
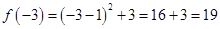
Therefore, absolute minimum value is 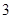 and absolute maximum value is 19.
and absolute maximum value is 19.
6. Find the maximum profit that a company can make, if the profit function is given by 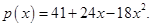
Ans. Given: Profit function 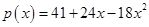

 and
and 
Now 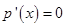

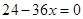

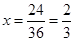
At 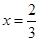 ,
,  [Negative]
[Negative]

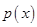 has a local maximum value at
has a local maximum value at 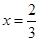 .
.
 At
At 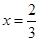 , Maximum profit
, Maximum profit
= 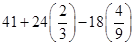
= 41 + 16 – 8 = 49
7. Find both the maximum value and minimum value of 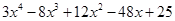 on the interval [0, 3].
on the interval [0, 3].
Ans. Let 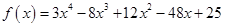 on [0, 3]
on [0, 3]

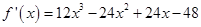
Now 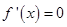

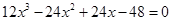

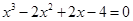

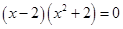

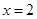 or
or 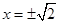
Since 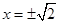 is imaginary, therefore it is rejected.
is imaginary, therefore it is rejected.

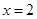 is turning point.
is turning point.
 At
At 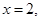
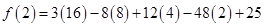 =
= 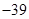
At 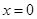
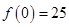
At 
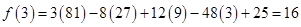
Therefore, absolute minimum value is 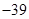 and absolute maximum value is 25.
and absolute maximum value is 25.
8. At what points on the interval 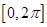 does the function
does the function 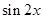 attain its maximum value?
attain its maximum value?
Ans. Let 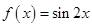

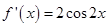
Now 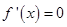



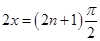

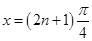
Putting 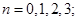
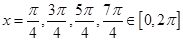
Now 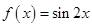

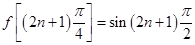
= 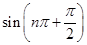
= 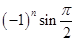 =
= 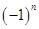
Putting 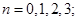
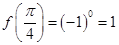
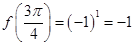
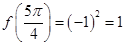
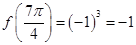
Also 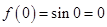 and
and 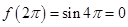
Since 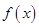 attains its maximum value 1 at
attains its maximum value 1 at 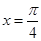 and
and 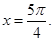
Therefore, the required points are  and
and 
9. What is the maximum value of the function 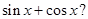
Ans. Let 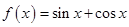


Now 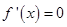



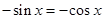
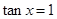 =
= 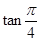

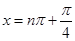 [Turning point]
[Turning point]

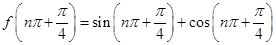
= 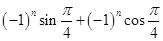
= 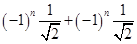
= 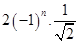
= 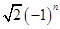
If 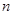 is even, then
is even, then 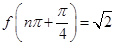
If 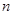 is odd, then
is odd, then 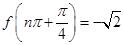
Therefore, maximum value of 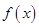 is
is 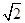 and minimum value of
and minimum value of 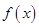 is
is 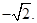
10. Find the maximum value of 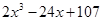 in the interval [1, 3]. Find the maximum value of the same function in
in the interval [1, 3]. Find the maximum value of the same function in 
Ans. Let 

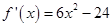
Now 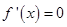

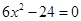



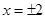

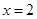 or
or 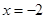 [Turning points]
[Turning points]
For Interval [1, 3], 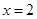 is turning point.
is turning point.
At 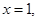

At 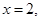
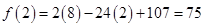
At 
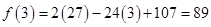
Therefore, maximum value of 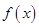 is 89.
is 89.
For Interval 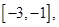
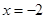 is turning point.
is turning point.
At 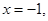

At 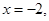
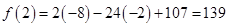
At 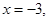
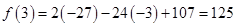
Therefore, maximum value of 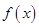 is 139.
is 139.
11. It is given that at 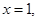 the function
the function 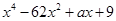 attains its maximum value, on the interval [0, 20]. Find the value of
attains its maximum value, on the interval [0, 20]. Find the value of 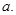
Ans. Let 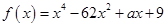

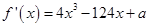
Since, 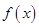 attains its maximum value at
attains its maximum value at  in the interval [0, 2], therefore
in the interval [0, 2], therefore 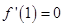

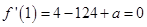

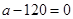


NCERT Solutions class 12 Maths Exercise 6.5
12. Find the maximum and minimum value of 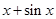 on
on 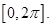
Ans. Let 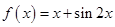

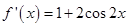
Now 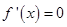






= 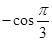 =
= 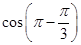
= 

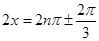 where
where 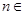 Z
Z 
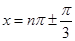
For 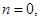
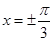 But
But 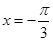
 , therefore
, therefore 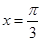
For 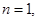
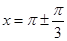 =
=  and
and 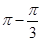
For 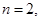
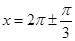
But 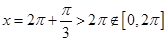 , therefore
, therefore 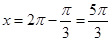
Therefore, it is clear that the only turning point of 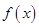 given by
given by 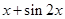 which belong to given closed interval
which belong to given closed interval 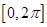 are,
are, 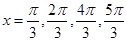
At 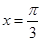
 nearly
nearly
At 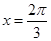
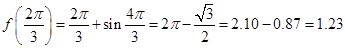 nearly
nearly
At 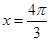
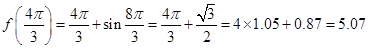 nearly
nearly
At 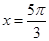
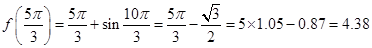 nearly
nearly
At 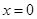
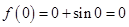
At 
 nearly
nearly
Therefore, Maximum value = 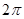 and minimum value = 0
and minimum value = 0
NCERT Solutions class 12 Maths Exercise 6.5
13. Find two numbers whose sum is 24 and whose product is as large as possible.
Ans. Let the two numbers be  and
and 
According to the question, 

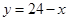 …….(i)
…….(i)
And let 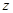 is the product of
is the product of  and
and 



 [From eq. (i)]
[From eq. (i)]

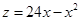

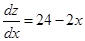 and
and 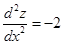
Now to find turning point, 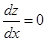

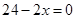

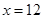
At 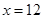 ,
, 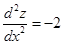 [Negative]
[Negative]

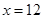 is a point of local maxima and
is a point of local maxima and 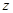 is maximum at
is maximum at 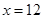 .
.
 From eq. (i),
From eq. (i), 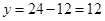
Therefore, the two required numbers are 12 and 12.
NCERT Solutions class 12 Maths Exercise 6.5
14. Find two positive integers  and
and 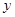 such that
such that 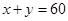 and
and 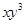 is maximum.
is maximum.
Ans. Given: 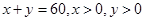 ……….(i)
……….(i)
Let P = 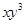 [To be maximized] ……….(ii)
[To be maximized] ……….(ii)
Putting from eq. (i),  in eq. (ii),
in eq. (ii),
P = 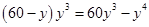

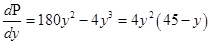 …..(iii)
…..(iii)
Now 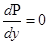

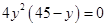

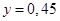
It is clear that  changes sign from positive to negative as
changes sign from positive to negative as 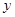 increases through 45.
increases through 45.
Therefore, P is maximum when 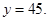
Hence, 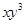 is maximum when
is maximum when 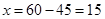 and
and 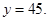
NCERT Solutions class 12 Maths Exercise 6.5
15. Find two positive integers  and
and 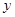 such that their sum is 35 and the product
such that their sum is 35 and the product 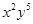 is a maximum.
is a maximum.
Ans. Given: 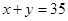

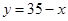 ……….(i)
……….(i)
Let 

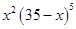 [From eq. (i)]
[From eq. (i)]

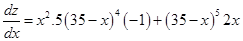

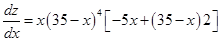

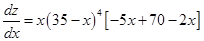

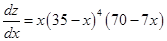

 ……….(ii)
……….(ii)
Now 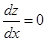



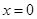 or
or 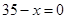 or
or 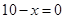

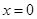 or
or  or
or 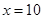
Now 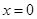 is rejected because according to question,
is rejected because according to question,  is a positive number.
is a positive number.
Also  is rejected because from eq. (i),
is rejected because from eq. (i), 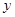 = 35 – 35 = 0, but
= 35 – 35 = 0, but 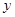 is positive.
is positive.
Therefore, 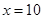 is only the turning point.
is only the turning point.

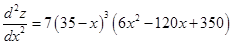
At 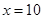 ,
, 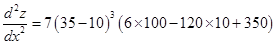
= 
 By second derivative test,
By second derivative test,  will be maximum at
will be maximum at 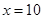 when
when 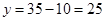 .
.
Therefore, the required numbers are 10 and 25.
NCERT Solutions class 12 Maths Exercise 6.5
16. Find two positive integers whose sum is 16 and sum of whose cubes is minimum.
Ans. Let the two positive numbers are  and
and 

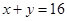

 ……….(i)
……….(i)
Let 

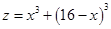 [From eq. (i)]
[From eq. (i)]

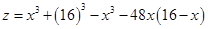
= 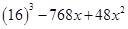

 and
and 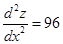
Now  = 0
= 0

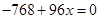

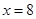
At 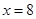
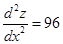 is positive.
is positive.

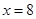 is a point of local minima and
is a point of local minima and 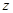 is minimum when
is minimum when 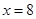 .
.

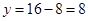
Therefore, the required numbers are 8 and 8.
NCERT Solutions class 12 Maths Exercise 6.5
17. A square piece of tin of side 18 cm is to be made into a box without top, by cutting a square from each corner and folding up the flaps to form the box. What should be the side of the square to be cut off so that the volume of the box is the maximum possible?
Ans. Given: Each side of square piece of tin is 18 cm.
Let  cm be the side of each of the four squares cut off from each corner.
cm be the side of each of the four squares cut off from each corner.
Then dimensions of the open box formed by folding the flaps after cutting off squares are 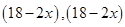 and
and  cm.
cm.
Let 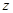 denotes the volume of the open box.
denotes the volume of the open box.



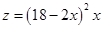

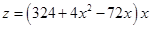
= 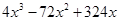

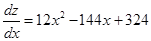 and
and 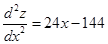
Now  = 0
= 0

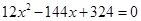
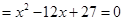

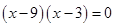

 or
or 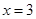
 is rejected because at
is rejected because at  length =
length =  which is impossible.
which is impossible.

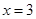 is the turning point.
is the turning point.
At 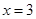 ,
,  [Negative]
[Negative]
· 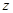 is minimum at
is minimum at 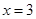 i.e., side of each square to be cut off from each corner for maximum volume is 3 cm.
i.e., side of each square to be cut off from each corner for maximum volume is 3 cm.
NCERT Solutions class 12 Maths Exercise 6.5
18. A rectangular sheet of tin 45 cm by 24 cm is to be made into a box without top, by cutting off square from each corner and folding up the flaps. What should be the side of the square to be cut off so that the volume of the box is maximum?
Ans. Given: Dimensions of rectangular sheet are 45 cm and 24 cm.
Let  cm be the side of each of the four squares cut off from each corner.
cm be the side of each of the four squares cut off from each corner.
Then dimensions of the open box formed by folding the flaps after cutting off squares are 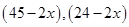 and
and  cm.
cm.
Let 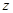 denotes the volume of the open box.
denotes the volume of the open box.



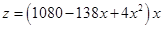
= 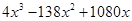

 and
and 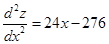
Now  = 0
= 0

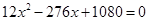

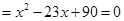



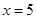 or
or 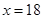
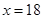 is rejected because at
is rejected because at 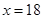 length =
length = 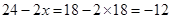 which is impossible.
which is impossible.

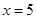 is the turning point.
is the turning point.
At 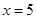 ,
, 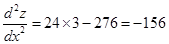 [Negative]
[Negative]
· 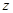 is minimum at
is minimum at 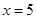 i.e., side of each square to be cut off from each corner for maximum volume is 5 cm.
i.e., side of each square to be cut off from each corner for maximum volume is 5 cm.
NCERT Solutions class 12 Maths Exercise 6.5
19. Show that of all the rectangles inscribed in a given fixed circle, the square has maximum area.
Ans. Let PQRS be the rectangle inscribed in a given circle with centre O and radius 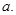
Let  and
and  be the length and breadth of the rectangle, i.e.,
be the length and breadth of the rectangle, i.e., 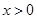 and
and 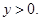
In right angled triangle PQR, using Pythagoras theorem,
PQ2 + QR2 = PR2

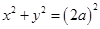

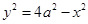

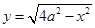 …..(i)
…..(i)
Let A be the area of the rectangle, then A =  =
= 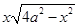

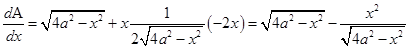
= 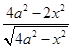
And 
= 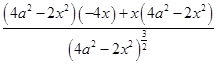


= 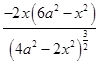
Now 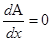

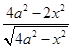 = 0
= 0

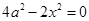

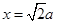
 At
At 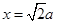 ,
, 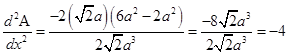 [Negative]
[Negative]
 At
At 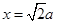 , area of rectangle is maximum.
, area of rectangle is maximum.
And from eq. (i), 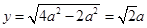 ,
,
i.e., 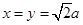
Therefore, the area of inscribed rectangle is maximum when it is square.
NCERT Solutions class 12 Maths Exercise 6.5
20. Show that the right circular cylinder of given surface and maximum volume is such that its height is equal to the diameter of the base.
Ans. Let  be the radius of the circular base and
be the radius of the circular base and 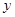 be the height of closed right circular cylinder.
be the height of closed right circular cylinder.
 Total surface area (S) =
Total surface area (S) = 

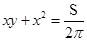 =
=  (say)
(say)

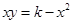

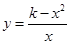 …..(i)
…..(i)
Volume of cylinder 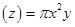
= 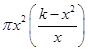 [From eq. (i)]
[From eq. (i)]

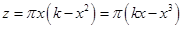

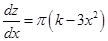 and
and 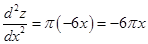
Now 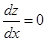

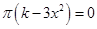

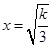
At 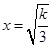
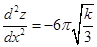 [Negative]
[Negative]

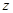 is maximum at
is maximum at 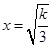 .
.
 From eq. (i),
From eq. (i), 
= 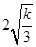 =
= 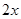
 Height = Diameter
Height = Diameter
Therefore, the volume of cylinder is maximum when its height is equal to the diameter of its base.
NCERT Solutions class 12 Maths Exercise 6.5
21. Of all the closed cylindrical cans (right circular), of a given volume of 100 cubic centimeters, find the dimensions of the can which has the minimum surface area.
Ans. Let  be the radius of the circular base and
be the radius of the circular base and 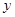 be the height of closed right circular cylinder.
be the height of closed right circular cylinder.
According to the question, Volume of the cylinder 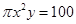

 …(i)
…(i)
 Total surface area (S) =
Total surface area (S) = 
= 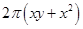
= 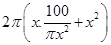 [From eq. (i)]
[From eq. (i)]
 S =
S = 
= 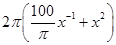

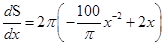 and
and 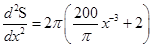
Now 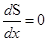

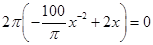



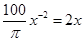

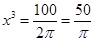

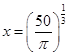
At 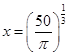
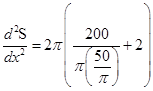
= 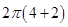 =
= 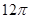 [Positive]
[Positive]
 S is minimum when radius
S is minimum when radius 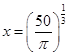 cm
cm
 From eq. (i)
From eq. (i)
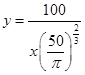
= 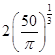 =
= 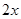
NCERT Solutions class 12 Maths Exercise 6.5
22. A wire of length 28 m is to be cut into two pieces. One of the pieces is to be made into a square and the other into a circle. What should be the length of the two pieces so that the combined area of the square and the circle is minimum?
Ans. Let  meters be the side of square and
meters be the side of square and 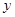 meters be the radius of the circle.
meters be the radius of the circle.
Length of the wire = Perimeter of square + Circumference of circle

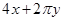 = 28
= 28



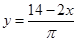 ……….(i)
……….(i)
Area of square = 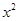 and Area of circle =
and Area of circle = 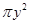
Combined area (A) = 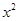 +
+ 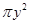 =
= 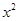 +
+ 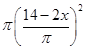
= 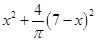

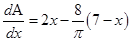 and
and 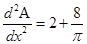
Now 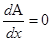

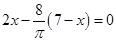

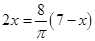

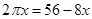

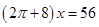

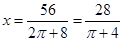
And 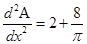 [Positive]
[Positive]
 A is minimum when
A is minimum when 
Therefore, the wire should be cut at a distance 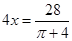 from one end.
from one end.
NCERT Solutions class 12 Maths Exercise 6.5
23. Prove that the volume of the largest cone that can be inscribed in a sphere of radius R is  of the volume of the sphere.
of the volume of the sphere.
Ans. Let O be the centre and R be the radius of the given sphere, BM =  and AM =
and AM = 
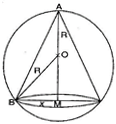
In right angled triangle OMB, using Pythagoras theorem,
OM2 + BM2 = OB2

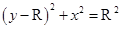

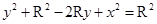

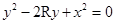

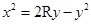 ……….(i)
……….(i)
Volume of a cone inscribed in the given sphere
 =
= 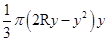

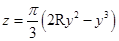 ……….(ii)
……….(ii)

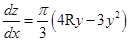 and
and 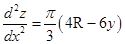
Now 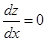



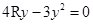

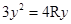

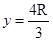
At 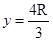
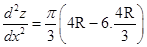
= 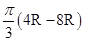
= 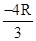 [Negative]
[Negative]

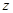 is maximum at
is maximum at 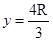
 From eq. (i)
From eq. (i) 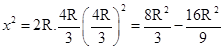
= 
 Maximum volume of the cone
Maximum volume of the cone
= 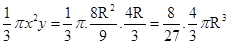
=  (Volume of the sphere)
(Volume of the sphere)
NCERT Solutions class 12 Maths Exercise 6.5
24. Show that the right circular cone of least curve surface and given volume has an altitude equal to 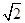 time the radius of the base.
time the radius of the base.
Ans. Let  be the radius and
be the radius and 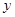 be the height of the cone.
be the height of the cone.
 Volume of the cone (V) =
Volume of the cone (V) = 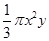

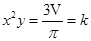 (say) ……….(i)
(say) ……….(i)
And Surface area of the cone (S) = 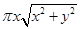

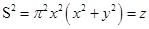 (say) ….(ii)
(say) ….(ii)

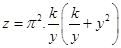
= 
= 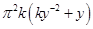

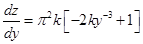 and
and 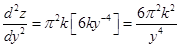
Now 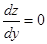

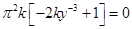

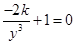

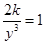

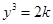

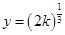 ……….(iii)
……….(iii)
At 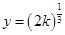
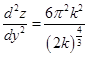 [Positive]
[Positive]

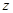 is minimum when
is minimum when 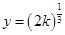
 From eq. (i),
From eq. (i), 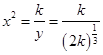
=  [From eq. (iii)]
[From eq. (iii)]

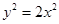


Therefore, Surface area is minimum when height = 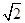 (radius of base)
(radius of base)
NCERT Solutions class 12 Maths Exercise 6.5
25. Show that the semi-vertical angle of the cone of the maximum value and of given slant height is 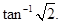
Ans. Let  be the radius,
be the radius, 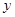 be the height,
be the height, 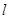 be the slant height of given cone and
be the slant height of given cone and  be the semi-vertical angle of cone.
be the semi-vertical angle of cone.

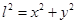

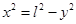 ……….(i)
……….(i)
 Volume of the cone (V) =
Volume of the cone (V) = 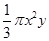 ……….(ii)
……….(ii)
 V =
V = 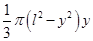
= 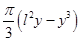

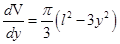 and
and 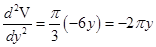
Now 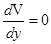



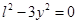

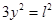

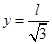
At 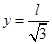
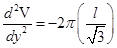
= 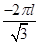 [Negative]
[Negative]
 V is maximum at
V is maximum at 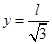
 From eq. (i),
From eq. (i), 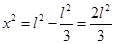

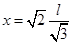
 Semi-vertical angle,
Semi-vertical angle, 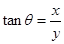
= 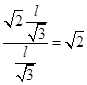

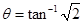
NCERT Solutions class 12 Maths Exercise 6.5
26. Show that the semi-vertical angle of the right circular cone of given surface area and maximum volume is 
Ans. Let  be the radius and
be the radius and 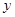 be the height of the cone and semi-vertical angle be
be the height of the cone and semi-vertical angle be 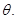
 Total Surface area of cone (S) =
Total Surface area of cone (S) = 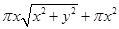

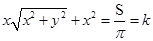 (say)
(say)

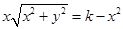

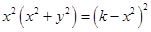

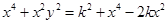



 ……….(i)
……….(i)
Volume of cone (V) = 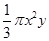
= 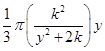
= 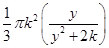

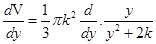
= 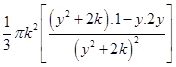 [Using quotient rule]
[Using quotient rule]

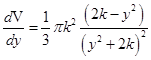 ……….(ii)
……….(ii)
Now 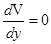

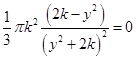

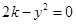

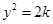

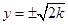

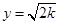 [height can’t be negative]
[height can’t be negative]

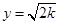 is the turning point.
is the turning point.
Since, 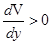 , therefore, Volume is maximum at
, therefore, Volume is maximum at 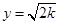
 From eq. (i),
From eq. (i), 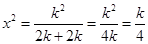

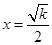
Now Semi-vertical angle of the cone 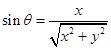 =
= 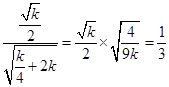

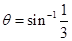
Choose the correct answer in the Exercises 27 to 29.
NCERT Solutions class 12 Maths Exercise 6.5
27. The point on the curve 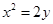 which is nearest to the point (0, 5) is:
which is nearest to the point (0, 5) is:
(A) 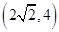
(B) 
(C) (0, 0)
(D) (2, 2)
Ans. Equation of the curve is 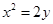 ……….(i)
……….(i)
Let P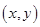 be any point on the curve (i), then according to question,
be any point on the curve (i), then according to question,
Distance between given point (0, 5) and P = 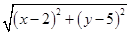
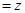 (say)
(say)

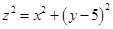
=  [From eq. (i)]
[From eq. (i)]

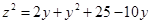

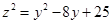 = Z (say)
= Z (say)

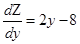 and
and 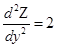
Now 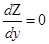

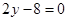


At 
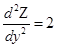 [Positive]
[Positive]
 Z is minimum and
Z is minimum and 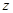 is minimum at
is minimum at 
 From eq. (i)
From eq. (i)
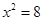



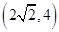 and
and 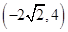 are two points on curve (i) which are nearest to (0, 5).
are two points on curve (i) which are nearest to (0, 5).
Therefore, option (A) is correct.
28. For all real values of 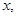 the minimum value of
the minimum value of 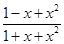 is:
is:
(A) 0
(B) 1
(C) 3
(D) 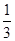
Ans. Given: 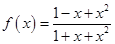 ……….(i)
……….(i)

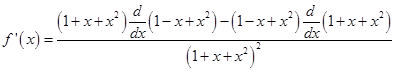

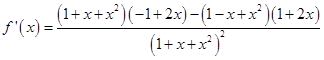



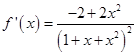 =
= 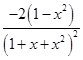
Now 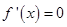

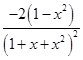 = 0
= 0

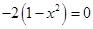

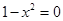

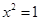

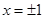

 and
and 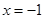 [Turning points]
[Turning points]
At 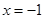 ,
,
from eq. (i),
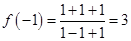
At  ,
,
from eq. (i),
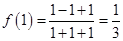 [Minimum value]
[Minimum value]
Therefore, option (D) is correct.
29. The maximum value of 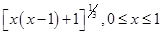 is:
is:
(A) 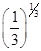
(B) 
(C) 1
(D) 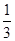
Ans. Let 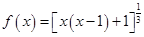
= 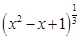 ,
, 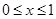 ……….(i)
……….(i)


= 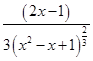
Now 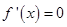

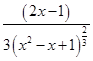 = 0
= 0

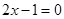

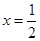 [Turning point] and it belongs to the given enclosed interval
[Turning point] and it belongs to the given enclosed interval 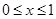 i.e., [0, 1].
i.e., [0, 1].
At 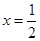 , from eq. (i),
, from eq. (i),
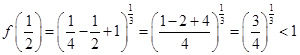
At 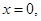 from eq. (i),
from eq. (i),
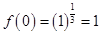
At  , from eq. (i),
, from eq. (i),
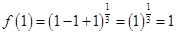
 Maximum value of
Maximum value of 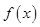 is 1.
is 1.
Therefore, option (C) is correct.
NCERT Solutions class 12 Maths Exercise 6.5
NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes text book solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 20 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide
CBSE App for Class 12
To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
myCBSEguide
Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes
Install Now
In solution 17th, it should be maximum not minimum.
god must be crazy
yea i agree
I am very impressed with the way the problems are explained here. Students will really understand the solutions very easily. Thanks
I am very impressed with the way the problems are explained here. Students will really understand the solutions very easily. Thanks