
myCBSEguide App
Download the app to get CBSE Sample Papers 2023-24, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowNCERT Solutions class 12 Maths Exercise 6.4 Class 12 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
Download NCERT solutions for Applications of Derivatives as PDF.

NCERT Solutions for Class 12 Maths Application of Derivatives
1. Using differentials, find the approximate value of each of the following up to 3 places of decimal:
(i) 
(ii) 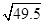
(iii) 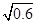
(iv) 
(v) 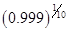
(vi) 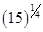
(vii) 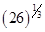
(viii) 
(ix) 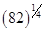
(x) 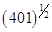
(xi) 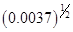
(xii) 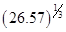
(xiii) 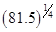
(xiv) 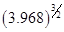
(xv) 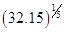
Ans. (i) 
Let 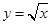 ……….(i)
……….(i)

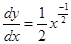 =
= 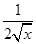

 ……….(ii)
……….(ii)
Now, from eq. (i), 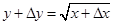
= 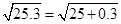
Here, 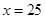 and
and 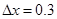 , then
, then 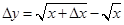
= 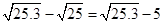

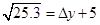
Since, 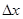 and
and 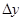 is approximately equal to
is approximately equal to 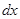 and
and 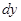 respectively.
respectively.
 From eq. (ii),
From eq. (ii),
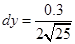 = 0.03
= 0.03
Therefore, approximately value of  is 5 + 0.03 = 5.03.
is 5 + 0.03 = 5.03.
(ii) 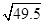
Let 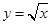 ……….(i)
……….(i)

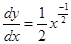 =
= 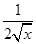

 ……….(ii)
……….(ii)
Now, from eq. (i), 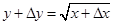
= 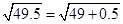
Here, 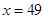 and
and 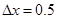 , then
, then
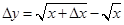
= 

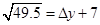
Since, 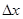 and
and 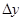 is approximately equal to
is approximately equal to 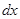 and
and 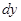 respectively.
respectively.
 From eq. (ii),
From eq. (ii), 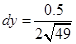
= 0.0357
Therefore, approximately value of 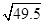 is 7 + 0.0357 = 7.0357.
is 7 + 0.0357 = 7.0357.
(iii) 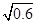
Let 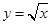 ……….(i)
……….(i)

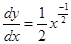 =
= 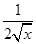

 ……….(ii)
……….(ii)
Now, from eq. (i), 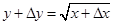
= 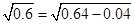
Here, 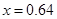 and
and 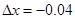 , then
, then
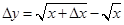
= 

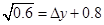
Since, 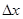 and
and 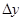 is approximately equal to
is approximately equal to 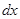 and
and 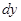 respectively.
respectively.
 From eq. (ii),
From eq. (ii),  =
= 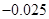
Therefore, approximately value of 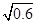 is 0.8 – 0.025 = 0.775.
is 0.8 – 0.025 = 0.775.
(iv) 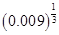
Let 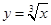 ……….(i)
……….(i)

 =
= 

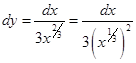 ……….(ii)
……….(ii)
Now, from eq. (i), 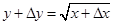
= 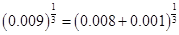
Here, 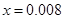 and
and  ,
,
then 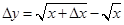
= 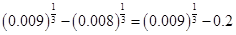

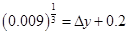
Since, 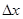 and
and 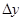 is approximately equal to
is approximately equal to 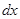 and
and 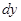 respectively.
respectively.
 From eq. (ii),
From eq. (ii), 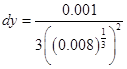
= 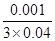 = 0.0083
= 0.0083
Therefore, approximately value of 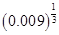 is 0.2 + 0.0083 = 0.2083.
is 0.2 + 0.0083 = 0.2083.
(v) 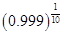
Let  ……….(i)
……….(i)

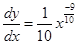 =
= 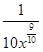

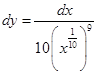 ……….(ii)
……….(ii)
Now, from eq. (i), 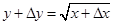
= 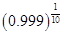 =
= 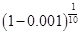 ……….(iii)
……….(iii)
Here 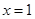 and
and 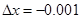
Then 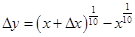
= 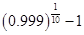

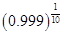 =
= 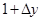
Since, 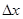 and
and 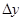 is approximately equal to
is approximately equal to 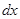 and
and 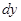 respectively.
respectively.
 From eq. (ii),
From eq. (ii),
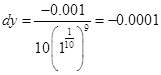
Therefore, approximate value of 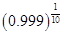 is 1 – 0.0001 = 0.9999.
is 1 – 0.0001 = 0.9999.
(vi) 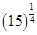
Let  ……….(i)
……….(i)

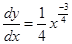 =
= 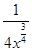

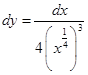 ……….(ii)
……….(ii)
Now, from eq. (i), 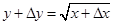
= 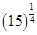 =
= 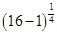 ……….(iii)
……….(iii)
Here 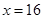 and
and 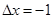
Then 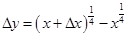
= 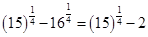

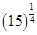 =
= 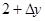
Since, 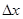 and
and 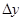 is approximately equal to
is approximately equal to 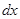 and
and 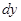 respectively.
respectively.
 From eq. (ii),
From eq. (ii), 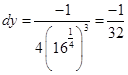
Therefore, approximate value of 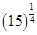 is
is 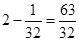 = 1.96875.
= 1.96875.
(vii) 
Let 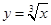 ……….(i)
……….(i)

 =
= 

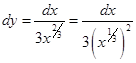 ……….(ii)
……….(ii)
Now, from eq. (i), 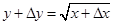
= 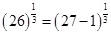
Here, 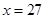 and
and 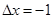 ,
,
then 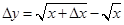 =
= 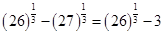

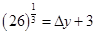
Since, 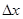 and
and 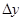 is approximately equal to
is approximately equal to 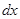 and
and 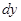 respectively.
respectively.
 From eq. (ii),
From eq. (ii), 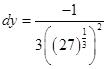 =
= 
Therefore, approximately value of  is
is 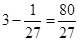 = 2.9629.
= 2.9629.
(viii) 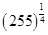
Let  ……….(i)
……….(i)

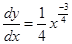 =
= 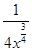

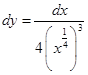 ……….(ii)
……….(ii)
Now, from eq. (i), 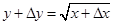
= 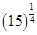 =
= 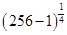 ……….(iii)
……….(iii)
Here 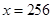 and
and 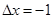
Then 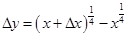
= 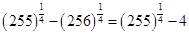

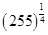 =
= 
Since, 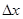 and
and 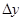 is approximately equal to
is approximately equal to 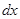 and
and 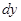 respectively.
respectively.
 From eq. (ii),
From eq. (ii), 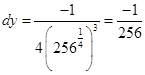
Therefore, approximate value of 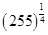 is
is 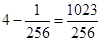 = 3.9961.
= 3.9961.
(ix) 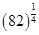
Let  ……….(i)
……….(i)

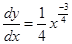
= 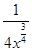

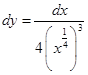 ……….(ii)
……….(ii)
Now, from eq. (i), 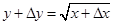 =
= 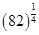 =
= 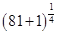 ……….(iii)
……….(iii)
Here 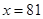 and
and 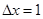
Then 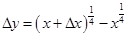
= 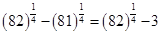

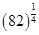 =
= 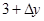
Since, 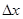 and
and 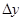 is approximately equal to
is approximately equal to 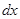 and
and 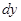 respectively.
respectively.
 From eq. (ii),
From eq. (ii), 
Therefore, approximate value of 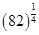 is
is 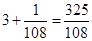 = 3.0092.
= 3.0092.
(x) 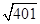
Let 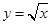 ……….(i)
……….(i)

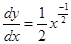 =
= 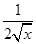

 ……….(ii)
……….(ii)
Now, from eq. (i), 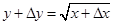
= 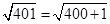
Here, 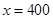 and
and  , then
, then 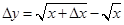
= 

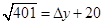
Since, 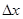 and
and 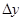 is approximately equal to
is approximately equal to 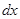 and
and 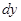 respectively.
respectively.
 From eq. (ii),
From eq. (ii),  =
= 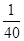
Therefore, approximately value of 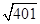 is
is 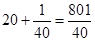 = 20.025.
= 20.025.
(xi) 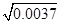
Let 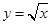 ……….(i)
……….(i)

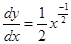 =
= 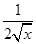

 ……….(ii)
……….(ii)
Now, from eq. (i), 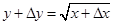
= 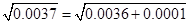
Here, 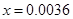 and
and 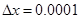 , then
, then
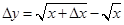
= 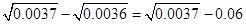

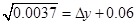
Since, 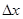 and
and 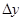 is approximately equal to
is approximately equal to 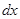 and
and 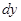 respectively.
respectively.
 From eq. (ii),
From eq. (ii), 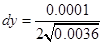
= 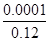
Therefore, approximately value of 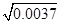 is 0.06 +
is 0.06 + 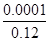 = 0.060833.
= 0.060833.
(xi) 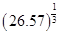
Let 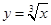 ……….(i)
……….(i)

 =
= 

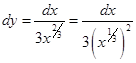 ……….(ii)
……….(ii)
Now, from eq. (i), 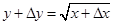
= 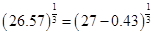
Here, 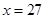 and
and  ,
,
then 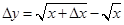
= 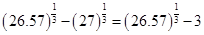

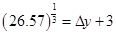
Since, 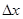 and
and 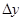 is approximately equal to
is approximately equal to 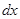 and
and 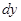 respectively.
respectively.
 From eq. (ii),
From eq. (ii), 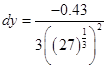
= 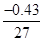 = 0.0159
= 0.0159
Therefore, approximately value of 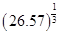 is 3 – 0.0159 = 2.9841.
is 3 – 0.0159 = 2.9841.
(xii) 
Let  ……….(i)
……….(i)

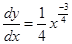 =
= 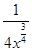

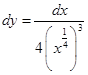 ……….(ii)
……….(ii)
Now, from eq. (i), 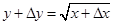
=  =
= 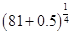 ……….(iii)
……….(iii)
Here 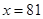 and
and 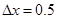
Then 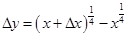
= 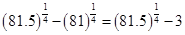

 =
= 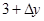
Since, 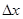 and
and 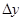 is approximately equal to
is approximately equal to 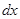 and
and 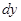 respectively.
respectively.
 From eq. (ii),
From eq. (ii), 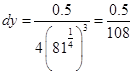
= 0.00462
Therefore, approximate value of 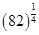 is 3 + 0.00462= 3.00462.
is 3 + 0.00462= 3.00462.
(xiv) 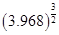
Let 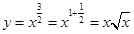 ……….(i)
……….(i)

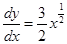

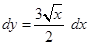 ……….(ii)
……….(ii)
Now, from eq. (i), 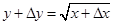
= 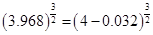
Here, 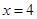 and
and  , then
, then 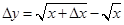
= 

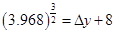
Since, 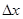 and
and 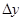 is approximately equal to
is approximately equal to 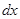 and
and 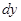 respectively.
respectively.
 From eq. (ii),
From eq. (ii),
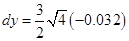 =
= 
Therefore, approximately value of 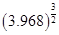 is 8 – 0.096 = 7.904.
is 8 – 0.096 = 7.904.
(xv) 
Let 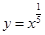 ……….(i)
……….(i)

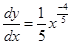 =
= 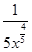

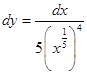 ……….(ii)
……….(ii)
Now, from eq. (i), 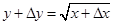
=  =
= 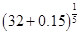 ……….(iii)
……….(iii)
Here 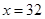 and
and 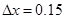
Then 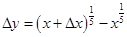
= 

 =
= 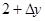
Since, 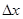 and
and 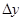 is approximately equal to
is approximately equal to 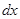 and
and 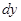 respectively.
respectively.
 From eq. (ii),
From eq. (ii),
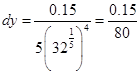 = 0.001875
= 0.001875
Therefore, approximate value of  is 2 + 0.001875= 2.001875.
is 2 + 0.001875= 2.001875.
2. Find the approximate value of 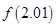 where
where 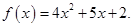
Ans. Let 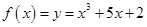 ……….(i)
……….(i)

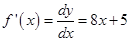


= 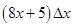 ……….(ii)
……….(ii)
Changing 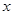 to
to 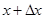 and
and 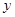 to
to 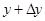 in eq. (i),
in eq. (i),
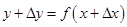
=  ……….(iii)
……….(iii)
Here, 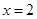 and
and 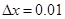
 From eq. (iii),
From eq. (iii), 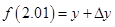
Since, 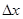 and
and 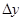 is approximately equal to
is approximately equal to 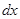 and
and 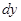 respectively.
respectively.
 From eq. (i) and (ii),
From eq. (i) and (ii), 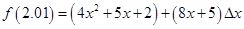

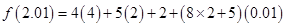 = 28.21
= 28.21
Therefore, approximate value of 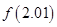 is 28.21.
is 28.21.
NCERT Solutions class 12 Maths Exercise 6.4
3. Find the approximate value of 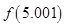 where
where 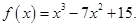
Ans. Let 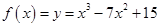 ……….(i)
……….(i)

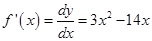

 ……….(ii)
……….(ii)
Changing 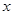 to
to 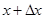 and
and 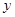 to
to 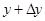 in eq. (i),
in eq. (i),
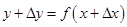
= 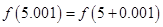 ……….(iii)
……….(iii)
Here,  and
and 
 From eq. (iii),
From eq. (iii), 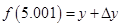
Since, 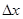 and
and 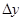 is approximately equal to
is approximately equal to 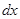 and
and 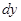 respectively.
respectively.
 From eq. (i) and (ii),
From eq. (i) and (ii),


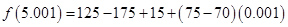
= 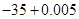 =
= 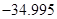
Therefore, approximate value of 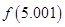 is
is 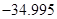 .
.
4. Find the approximate change in the volume of a cube of side 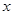 meters caused by increasing the side by 1%.
meters caused by increasing the side by 1%.
Ans. Since Volume (V) = 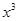 ……….(i)
……….(i)

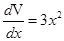 ……….(ii)
……….(ii)
It is given that increase in side = 1% = 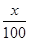

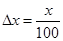 ……….(iii)
……….(iii)
Since approximate change in volume V of cube = 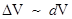 =
= 
= 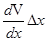
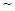
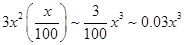 cubic meters
cubic meters
5. Find the approximate change in the surface area of a cube of side 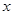 meters caused by decreasing the side by 1%.
meters caused by decreasing the side by 1%.
Ans. Since Surface area (S) = 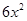

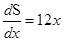
It is given that decrease in side = 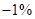 of
of 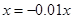

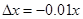
Since approximate change in surface area S of cube = 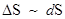 =
= 
= 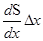
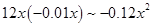 square meters (decreasing)
square meters (decreasing)
NCERT Solutions class 12 Maths Exercise 6.4
6. If the radius of a sphere is measured as 7 m with an error of 0.02 m, then find the approximate error in calculating its volume.
Ans. Let 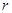 be the radius of the sphere and
be the radius of the sphere and 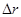 be the error in measuring the radius.
be the error in measuring the radius.
Then, according to the question, 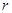 = 7 m and
= 7 m and 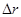 = 0.02 m
= 0.02 m
 Volume of sphere (V) =
Volume of sphere (V) = 

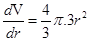
 Approximate error in calculating the volume = Approximate value of
Approximate error in calculating the volume = Approximate value of 
= 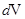 =
= 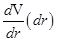
= 
= 
= 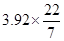 = 12.32 m3
= 12.32 m3
Therefore, the approximate error in calculating volume is 12.32 m3.
NCERT Solutions class 12 Maths Exercise 6.4
7. If the radius of a sphere is measured as 9 m with an error of 0.03 m, then find the approximate error in calculating its surface area.
Ans. Let 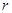 be the radius of the sphere.
be the radius of the sphere.
 Surface area of the sphere (S) =
Surface area of the sphere (S) = 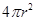



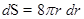

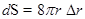
· 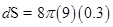
= 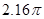 square meters
square meters
NCERT Solutions class 12 Maths Exercise 6.4
8. If 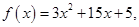 then the approximate value of
then the approximate value of 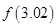 is:
is:
(A) 47.66
(B) 57.66
(C) 67.66
(D) 77.66
Ans. Let 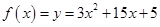 ……….(i)
……….(i)

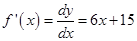

 ……….(ii)
……….(ii)
Changing 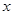 to
to 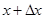 and
and 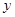 to
to 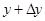 in eq. (i),
in eq. (i),
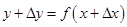
= 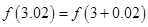 ……….(iii)
……….(iii)
Here, 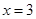 and
and 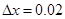
 From eq. (iii),
From eq. (iii), 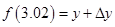
Since, 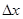 and
and 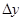 is approximately equal to
is approximately equal to 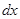 and
and 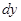 respectively.
respectively.
 From eq. (i) and (ii),
From eq. (i) and (ii),


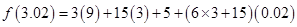
= 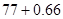 =
= 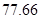
Therefore, option (D) is correct.
9. The approximate change in the volume of a cube of side 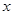 meters caused by increasing the side by 3% is:
meters caused by increasing the side by 3% is:
(A) 0.06 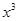 m3
m3
(B) 0.6 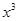 m3
m3
(C) 0.09 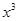 m3
m3
(D) 0.9 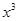 m3
m3
Ans. Since Volume (V) = 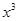 ……….(i)
……….(i)

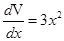 ……….(ii)
……….(ii)
It is given that increase in side = 3% = 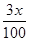

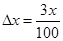 ……….(iii)
……….(iii)
Since approximate change in volume V of cube = 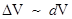 =
= 
= 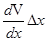
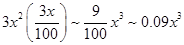 cubic meters
cubic meters
Therefore, option (C) is correct.
NCERT Solutions class 12 Maths Exercise 6.4
NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes text book solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 20 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide
CBSE App for Class 12
To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
myCBSEguide
Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes
Install Now
This app is very useful … Thku for that ??