NCERT Solutions for Class 10 Maths Exercise 4.3

NCERT Solutions for Class 10 Maths Exercise 4.3 Class 10 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 10 Maths chapter wise NCERT solution for Maths Book for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.

NCERT solutions for Maths Quadratic Equations Download as PDF

NCERT Solutions for Class 10 Maths Quadratic Equations

1. Find the roots of the following quadratic equations if they exist by the method of completing square.

(i)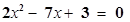

(ii)

(iii)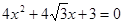

(iv)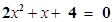

Ans. (i)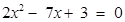

First we divide equation by 2 to make coefficient of x² equal to 1,

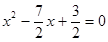
We divide middle term of the equation by 2x, we get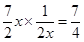

We add and subtract square of 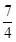from the equation ,

⇒

⇒

⇒

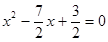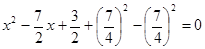
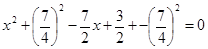
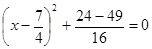
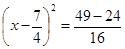
Taking Square root on both sides,

⇒

⇒

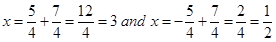
Therefore,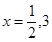

(ii)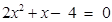

Dividing equation by 2,

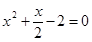
Following procedure of completing square,

⇒

⇒

⇒

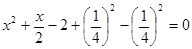
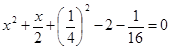
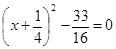
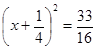
Taking square root on both sides,

⇒

⇒

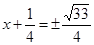
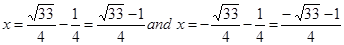
Therefore,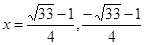

(iii)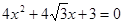

Dividing equation by 4,

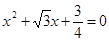
Following the procedure of completing square,

⇒

⇒

⇒

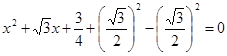
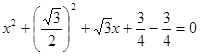
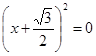
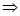
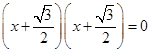
Taking square root on both sides,

⇒

⇒

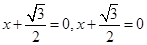
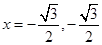
(iv)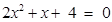

Dividing equation by 2,

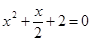
Following the procedure of completing square,

⇒

⇒

⇒

⇒

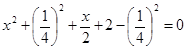
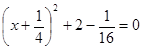
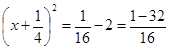
Taking square root on both sides

Right hand side does not exist because square root of negative number does not exist.

Therefore, there is no solution for quadratic equation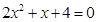

---

NCERT Solutions for Class 10 Maths Exercise 4.3

2. Find the roots of the following Quadratic Equations by applying quadratic formula.

(i)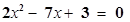

(ii)

(iii)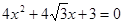

(iv)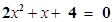

Ans. (i)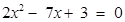

Comparing quadratic equation 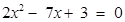with general form 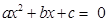, we get a = 2, b = -7 and c = 3

Putting these values in quadratic formula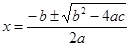

⇒

⇒

⇒

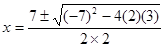
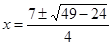
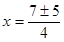
⇒ x = 3, ½

(ii)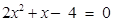

Comparing quadratic equation 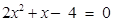with the general form 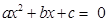, we get a = 2, b = 1 and c = −4

Putting these values in quadratic formula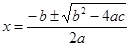

⇒

⇒

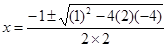
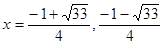
(iii)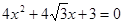

Comparing quadratic equation 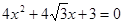with the general form 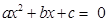, we get a = 4, b = 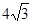and c = 3

Putting these values in quadratic formula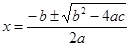

⇒

⇒

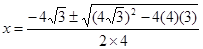
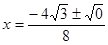
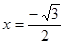
A quadratic equation has two roots. Here, both the roots are equal.

Therefore,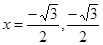

(iv)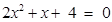

Comparing quadratic equation 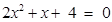with the general form 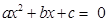, we get a = 2, b = 1 and c = 4

Putting these values in quadratic formula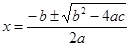

⇒

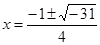
But, square root of negative number is not defined.

Therefore, Quadratic Equation 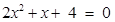has no solution.

---

NCERT Solutions for Class 10 Maths Exercise 4.3

3. Find the roots of the following equations:

(i)

(ii)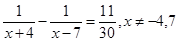

Ans. (i)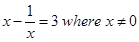

⇒

⇒

⇒

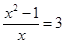
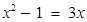
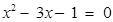
Comparing equation 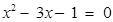with general form ,

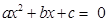We get a = 1, b = −3 and c = −1

Using quadratic formula 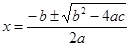to solve equation,

⇒

⇒

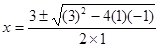
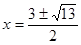
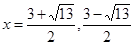
(ii)

⇒

⇒

⇒

⇒

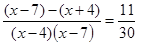
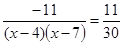
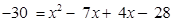
Comparing equation with general form ,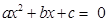

We get a = 1, b = −3 and c = 2

Using quadratic formula 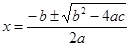to solve equation,

⇒

⇒

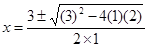
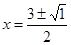
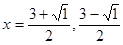
⇒ x = 2, 1

---

NCERT Solutions for Class 10 Maths Exercise 4.3

4. The sum of reciprocals of Rehman’s ages (in years) 3 years ago and 5 years from now is 13. Find his present age.

Ans. Let present age of Rehman = x years

Age of Rehman 3 years ago = (x − 3) years.

Age of Rehman after 5 years = (x + 5) years

According to the given condition:

⇒

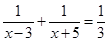
⇒ 3 (2x + 2) = (x − 3) (x + 5)

⇒ 6x + 6 =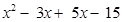

⇒

⇒

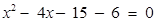
Comparing quadratic equation 0 with general form ,

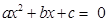We get a = 1, b = −4 and c = −21

Using quadratic formula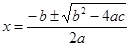

⇒

⇒

⇒

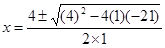
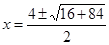
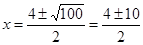
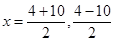
⇒ x = 7, −3

We discard x=−3. Since age cannot be in negative.

Therefore, present age of Rehman is 7 years.

---

NCERT Solutions for Class 10 Maths Exercise 4.3

5. In a class test, the sum of Shefali’s marks in Mathematics and English is 30. Had she got 2 marks more in Mathematics and 3 marks less in English, the product of their marks would have been 210. Find her marks in the two subjects.

Ans. Let Shefali’s marks in Mathematics = x

Let Shefali’s marks in English = 30 − x

If, she had got 2 marks more in Mathematics, her marks would be = x + 2

If, she had got 3 marks less in English, her marks in English would be = 30 – x − 3 = 27 − x

According to given condition:

(x + 2) (27 − x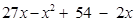) = 210

⇒ = 210

⇒

Comparing quadratic equation with general form ,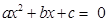

We get a = 1, b = −25 and c = 156

Applying Quadratic Formula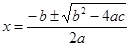

⇒

⇒

⇒

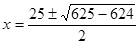
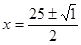
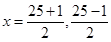
⇒ x = 13, 12

Therefore, Shefali’s marks in Mathematics = 13 or 12

Shefali’s marks in English = 30 – x = 30 – 13 = 17

Or Shefali’s marks in English = 30 – x = 30 – 12 = 18

Therefore, her marks in Mathematics and English are (13, 17) or (12, 18).

---

NCERT Solutions for Class 10 Maths Exercise 4.3

6. The diagonal of a rectangular field is 60 metres more than the shorter side. If, the longer side is 30 metres more than the shorter side, find the sides of the field.

Ans. Let shorter side of rectangle = xmetres

Let diagonal of rectangle = (x + 60) metres

Let longer side of rectangle = (x + 30) metres

According to pythagoras theorem,

=

⇒ =

⇒

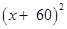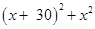
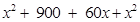
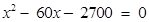
Comparing equation 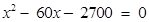with standard form ,

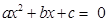We get a = 1, b = −60 and c = −2700

Applying quadratic formula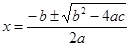

⇒

⇒

⇒

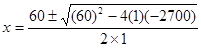
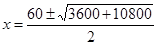
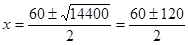
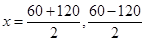
⇒ x = 90, –30

We ignore –30. Since length cannot be in negative.

Therefore, x = 90 which means length of shorter side = 90 metres

And length of longer side = x + 30 = 90 + 30 = 120 metres

Therefore, length of sides are 90 and 120 in metres.

---

NCERT Solutions for Class 10 Maths Exercise 4.3

7. The difference of squares of two numbers is 180. The square of the smaller number is 8 times the larger number. Find the two numbers.

Ans. Let smaller number = x and let larger number = y

According to condition:

… (1)

Also, we are given that square of smaller number is 8 times the larger number.

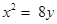⇒ … (2)

Putting equation (2) in (1), we get

⇒

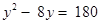
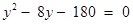
Comparing equation 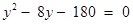with general form ,

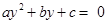We get a = 1, b = −8 and c = −180

Using quadratic formula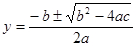

⇒

⇒

⇒

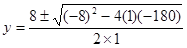
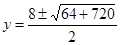
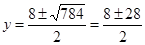
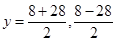
⇒ y = 18, −10

Using equation (2) to find smaller number:

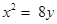
⇒ = 144

⇒ x = ±12

And, 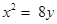= 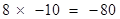{No real solution for x}

Therefore, two numbers are (12, 18) or (−12, 18)

---

NCERT Solutions for Class 10 Maths Exercise 4.3

8. A train travels 360 km at a uniform speed. If, the speed had been 5 km/hr more, it would have taken 1 hour less for the same journey. Find the speed of the train.

Ans. Let the speed of the train = x km/hr

If, speed had been 5 km/hr more, train would have taken 1 hour less.

So, according to this condition

⇒

⇒

⇒

⇒

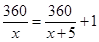
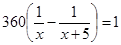
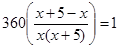
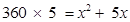
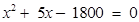
Comparing equation 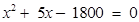with general equation ,

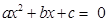We get a = 1, b = 5 and c = −1800

Applying quadratic formula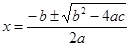

⇒

⇒

⇒

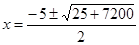
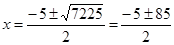
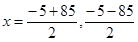
⇒ x = 40, −45

Since speed of train cannot be in negative. Therefore, we discard x = −45

Therefore, speed of train = 40 km/hr

---

NCERT Solutions for Class 10 Maths Exercise 4.3

9. Two water taps together can fill a tank in hours. The tap of larger diameter takes 10 hours less than the smaller one to fill the tank separately. Find the time in which each tap can separately fill the tank.

Ans. Let time taken by tap of smaller diameter to fill the tank = x hours

Let time taken by tap of larger diameter to fill the tank = (x – 10) hours

It means that tap of smaller diameter fills 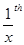part of tank in 1 hour.… (1)

And, tap of larger diameter fills 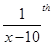part of tank in 1 hour. … (2)

When two taps are used together, they fill tank in 758 hours.

In 1 hour, they fill 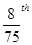part of tank… (3)

From (1), (2) and (3),

⇒

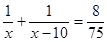
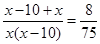
⇒ 75 (2x − 10) =

⇒ 150x – 750 =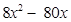

⇒

⇒

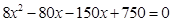
Comparing equation with general equation ,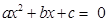

We get a = 4, b = −115 and c = 375

Applying quadratic formula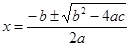

⇒

⇒

⇒

⇒

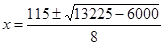
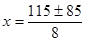
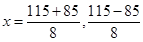
⇒ x = 25, 3.75

Time taken by larger tap = x – 10 = 3.75 – 10 = −6.25 hours

Time cannot be in negative. Therefore, we ignore this value.

Time taken by larger tap = x – 10 = 25 – 10 = 15 hours

Therefore, time taken by larger tap is 15 hours and time taken by smaller tap is 25 hours.

---

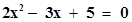
NCERT Solutions for Class 10 Maths Exercise 4.3

10. An express train takes 1 hour less than a passenger train to travel 132 km between Mysore and Bangalore (without taking into consideration the time they stop at intermediate stations). If, the average speed of the express train is 11 km/h more than that of the passenger train, find the average speed of two trains.

Ans. Let average speed of passenger train = x km/h

Let average speed of express train = (x + 11) km/h

Time taken by passenger train to cover 132 km = hours

Time taken by express train to cover 132 km = hours

According to the given condition,

⇒

⇒

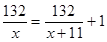
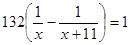
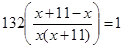
⇒ 132 (11) = x (x + 11)

⇒

⇒

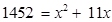
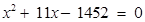
Comparing equation 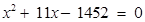with general quadratic equation 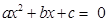, we get a = 1, b = 11 and c = −1452

Applying Quadratic Formula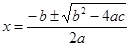

⇒

⇒

⇒

⇒

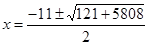
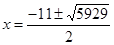
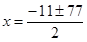
⇒ x = 33, −44

As speed cannot be in negative. Therefore, speed of passenger train = 33 km/h

And, speed of express train = x + 11 = 33 + 11 = 44 km/h

---

NCERT Solutions for Class 10 Maths Exercise 4.3

11. Sum of areas of two squares is 468 m². If, the difference of their perimeters is 24 metres, find the sides of the two squares.

Ans. Let perimeter of first square = x metres

Let perimeter of second square = (x + 24) metres

Length of side of first square = 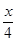metres {Perimeter of square = }

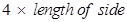Length of side of second square = 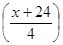metres

Area of first square = side × side =

Area of second square =

According to given condition:

⇒

⇒

⇒ =

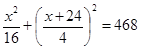
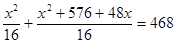
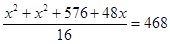
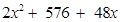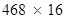
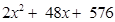⇒ = 7488

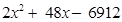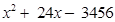⇒ = 0

⇒ = 0

Comparing equation 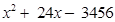=0 with standard form ,

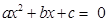We get a = 1, b =24 and c = −3456

Applying Quadratic Formula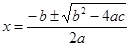

⇒

⇒

⇒

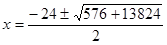
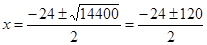
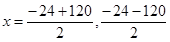
⇒ x = 48, −72

Perimeter of square cannot be in negative. Therefore, we discard x=−72.

Therefore, perimeter of first square = 48 metres

And, Perimeter of second square = x + 24 = 48 + 24 = 72 metres

⇒ Side of First square =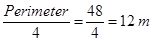

And, Side of second Square =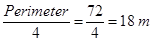

NCERT Solutions for Class 10 Maths Exercise 4.3

NCERT Solutions Class 10 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 10 Maths includes text book solutions from Mathematics Book. NCERT Solutions for CBSE Class 10 Maths have total 15 chapters. 10 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 10 solutions PDF and Maths ncert class 10 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.

CBSE app for Class 10

To download NCERT Solutions for Class 10 Maths, Computer Science, Home Science,Hindi ,English, Social Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.