NCERT Solutions class 12 Maths Exercise 6.1

Table of Contents

myCBSEguide App

Download the app to get CBSE Sample Papers 2024-25, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.

Install Now

NCERT Solutions class 12 Maths Exercise 6.1 Class 12 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.

Download NCERT solutions for Applications of Derivatives as PDF.

NCERT Solutions for Class 12 Maths Application of Derivatives

1. Find the rate of change of the area of a circle with respect to its radius 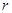when

(a) 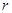= 3 cm

(b) 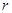= 4 cm

Ans. Let denote the area of the circle of variable radius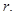

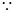Area of circle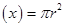

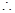Rate of change of area w.r.t.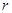

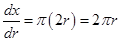
(a) When 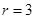cm, then

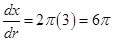sq. cm

(b) When 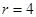cm, then

sq. cm

NCERT Solutions class 12 Maths Exercise 6.1

2. The volume of a cube is increasing at the rate of 8 cm³/sec. How fast is the surface area increasing when the length of an edge is 12 cm?

Ans. Let cm be the edge of the cube.

Given: Rate of increase of volume of cube = 8 cm³/sec

is positive = 8

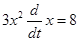
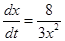……….(i)

Let 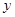be the surface area of the cube, i.e.,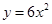

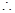Rate of change of surface area of the cube =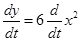

= =

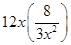
= cm²/sec

Putting = 12 cm (given),

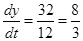cm²/sec

Since 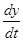is positive, therefore surface area is increasing at the rate of 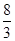cm²/sec.

NCERT Solutions class 12 Maths Exercise 6.1

3. The radius of the circle is increasing uniformly at the rate of 3 cm per second. Find the rate at which the area of the circle is increasing when the radius is 10 cm.

Ans. Let cm be the radius of the circle at time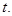

Rate of increase of radius of circle = 3 cm/sec

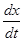is positive and = 3 cm/sec

Let 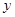be the area of the circle.

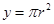
Rate of change of area of circle = =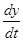

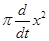
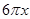
Putting = 10 cm (given),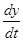

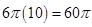= cm²/sec

Since 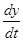is positive, therefore surface area is increasing at the rate of 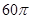cm²/sec.

NCERT Solutions class 12 Maths Exercise 6.1

4. An edge of a variable cube is increasing at the rate of 3 cm per second. How fast is the volume of the cube increasing when the edge if 10 cm long?

Ans. Let cm be the edge of variable cube at time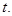

Rate of increase of edge = 3 cm/sec

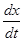is positive and = 3 cm/sec

Let 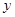be the volume of the cube.

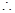Rate of change of volume of cube = =

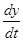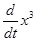
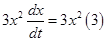
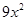= cm³/sec

Putting = 10 cm (given),

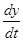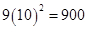= cm³/sec

Since 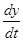is positive, therefore volume of cube is increasing at the rate of 900 cm³/sec.

NCERT Solutions class 12 Maths Exercise 6.1

5. A stone is dropped into a quite lake and waves move in circles at the rate of 5 cm/sec. At the instant when radius of the circular wave is 8 cm, how fast is the enclosed area increasing?

Ans. Let cm be the radius of the circular wave at time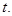

Rate of increase of radius of circular wave = 5 cm/sec

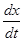is positive and = 5 cm/sec

Let 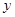be the enclosed area of the circular wave.

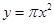
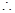Rate of change of area = =

= =

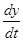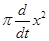
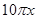
Putting = 8 cm (given),

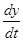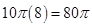= cm²/sec

Since 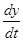is positive, therefore area of circular wave is increasing at the rate of cm²/sec.

NCERT Solutions class 12 Maths Exercise 6.1

6. The radius of a circle is increasing at the rate of 0.7 cm/s. What is the rate of its circumference?

Ans. Let cm be the radius of the circle at time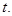

Rate of increase of radius of circle = 0.7 cm/sec

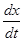is positive and = 0.7 cm/sec

Let 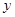be the circumference of the circle.

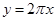
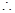Rate of change of circumference of circle =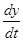

= =

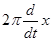= cm/sec

7. The length of a rectangle is decreasing at the rate of 5 cm/minute. When = 8 cm and 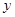= 6 cm, find the rates of change of (a) the perimeter and (b) the area of the rectangle.

Ans. Given: Rate of decrease of length of rectangle is 5 cm/minute.

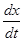is negative = –5 cm/minute

Also, Rate of increase of width 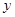of rectangle is 4 cm/minute

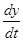is positive

= 4 cm/minute

(a) Let denotes the perimeter of rectangle.

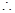
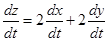
= is negative.

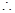Perimeter of the rectangle is decreasing at the rate of 2 cm/sec.

(b) Let denotes the area of rectangle.

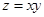
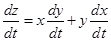
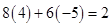= is positive.

Area of the rectangle is increasing at the rate of 2 cm²/sec.

8. A balloon, which always remains spherical on inflation, is being inflated by pumping in 900 cubic centimeters of gas per second. Find the rate at which the radius of the balloon increases when the radius is 15 cm.

Ans. Let cm be the radius of the spherical balloon at time

According to the question,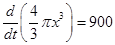

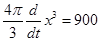
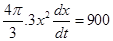
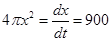
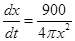
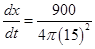
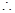Radius of balloon is increasing at the rate of 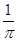cm sec.

9. A balloon, which always remains spherical has a variables radius. Find the rate at which its volume is increasing with the radius when the later is 10 cm.

Ans. Since, V =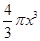

= =

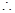
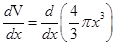
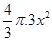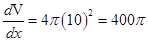
Therefore, the volume is increasing at the rate of 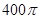cm³/sec.

10. A ladder 5 cm long is leaning against a wall. The bottom of the ladder is pulled along the ground, away from the wall, at the rate of 2 cm/s. How fast is its height on the wall decreasing when the foot of the ladder is 4 m away from the wall?

Ans. Let AB be the ladder and C is the junction of wall and ground, AB = 5 m B

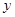Let CA = meters, CB = meters

According to the equation,

increases, 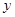decreases

and 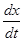= 2 cm/s

In 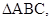AC² + BC² = AB² [Using Pythagoras theorem]

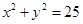……….(i)

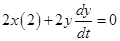
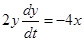
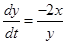……….(ii)

When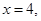

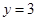[From eq. (i)]

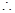From eq. (ii), 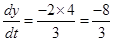cm/s

11. A particle moves along the curve 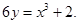Find the points on the curve at which the 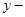coordinate is changing 8 times as fast as the 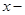coordinate.

Ans. Given: Equation of the curve ……….(i)

Let 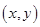be the required point on curve (i)

According to the question, 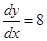……….(ii)

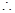From eq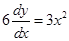. (i),

[From eq. (ii)]

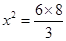
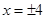
Taking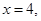

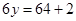
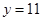
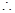Required point is (4, 11).

Taking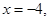

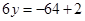
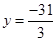
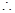Required point is .

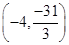12. The radius of an air bubble is increasing at the rate of 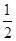cm/s. At what rate is the volume of the bubble increasing when the radius is 1 cm?

Ans. Let cm be the radius of the air bubble at time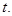

According to question, 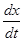is positive = 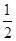cm/sec ……….(i)

Volume of air bubble

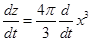
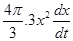
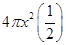
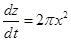
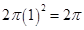
Therefore, required rate of increase of volume of air bubble is 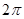cm³/sec.

13. A balloon which always remains spherical, has a variable diameter Find the rate of change of its volume with respect to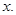

Ans. Given: Diameter of the ba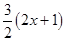lloon =

Radius of the balloon =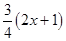

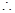Volume of the balloon =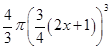

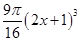= cu. units

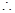Rate of change of volume w.r.t. =

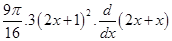
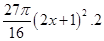
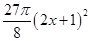
14. Sand is pouring from a pipe at the rate of 12 cm³/s. The falling sand forms a cone on the ground in such a way that the height of the cone is always one-sixth of the radius of the base. How fast is the height of the sand cone increasing when the height is 4cm?

Ans. Let the height and radius of the sand-cone formed at time 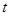second be 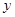cm and cm respectively.

According to question,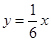

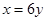
Volume of cone (V) =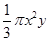

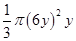
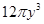
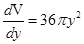
Now, since

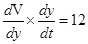
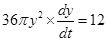
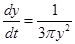
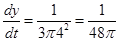cm/sec

15. The total cost C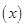 in rupees associated with the production of units of an item given by C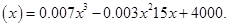 Find the marginal cost when 17 units are produced.

Ans. Marginal cost =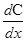

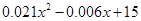
Now, when MC

= 6.069 – 0.102 + 15 = 20.967

Therefore, required Marginal cost is ` 20.97.

16. The total revenue in rupees received from the sale of units of a product is given by

R 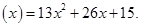Find the marginal revenue when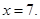

Ans. Marginal Revenue (MR) =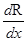

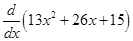
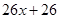
Now, when 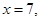MR = 26 x 7 + 26 = 208

Therefore, the required marginal revenue is ` 208.

Choose the correct answer in Exercises 17 and 18.

17. The rate of change of the area of a circle with respect to its radius 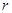at 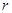=6 cm is:

(A)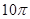

(B)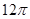

(C)

(D)

Ans. Area of circle (A) =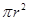

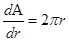
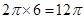
Therefore, option (B) is correct.

NCERT Solutions class 12 Maths Exercise 6.1

18. The total revenue in Rupees received from the sale of units of a product is given by

R The marginal revenue, when = 15 is:

(A) 116

(B) 96

(C) 90

(D) 126

Ans. Total re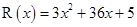venue

Marginal revenue =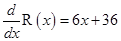

= 6 x 15 + 36 = 126

Therefore, option (D) is correct.

NCERT Solutions class 12 Maths Exercise 6.1

NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes text book solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 20 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide

NCERT Solutions for Mathematics Class 3rd to 12th

CBSE App for Class 12

To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator

Create question paper PDF and online tests with your own name & logo in minutes.

Create Now

myCBSEguide

Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes

Install Now

4 thoughts on “NCERT Solutions class 12 Maths Exercise 6.1”

Anonymous
July 24, 2018 at 9:24 am
It’s a very good site for all students of cbsc
Gaurav singh Tomar
July 24, 2018 at 9:25 am
It’s a very good site for all students of cbsc
Adorn Mariya Manson
December 10, 2018 at 10:13 pm
This is really a useful site for students. I could find the detailed answer of each & every question
Adorn Mariya Manson
December 10, 2018 at 10:15 pm
This is really a usefull site. Thank you.. ?

NCERT Solutions class 12 Maths Exercise 6.1

myCBSEguide App

NCERT Solutions for Class 12 Maths Application of Derivatives

NCERT Solutions class 12 Maths Exercise 6.1

2. The volume of a cube is increasing at the rate of 8 cm³/sec. How fast is the surface area increasing when the length of an edge is 12 cm?

NCERT Solutions class 12 Maths Exercise 6.1

3. The radius of the circle is increasing uniformly at the rate of 3 cm per second. Find the rate at which the area of the circle is increasing when the radius is 10 cm.

NCERT Solutions class 12 Maths Exercise 6.1

4. An edge of a variable cube is increasing at the rate of 3 cm per second. How fast is the volume of the cube increasing when the edge if 10 cm long?

NCERT Solutions class 12 Maths Exercise 6.1

5. A stone is dropped into a quite lake and waves move in circles at the rate of 5 cm/sec. At the instant when radius of the circular wave is 8 cm, how fast is the enclosed area increasing?

NCERT Solutions class 12 Maths Exercise 6.1

6. The radius of a circle is increasing at the rate of 0.7 cm/s. What is the rate of its circumference?

7. The length of a rectangle is decreasing at the rate of 5 cm/minute. When = 8 cm and = 6 cm, find the rates of change of (a) the perimeter and (b) the area of the rectangle.

8. A balloon, which always remains spherical on inflation, is being inflated by pumping in 900 cubic centimeters of gas per second. Find the rate at which the radius of the balloon increases when the radius is 15 cm.

9. A balloon, which always remains spherical has a variables radius. Find the rate at which its volume is increasing with the radius when the later is 10 cm.

10. A ladder 5 cm long is leaning against a wall. The bottom of the ladder is pulled along the ground, away from the wall, at the rate of 2 cm/s. How fast is its height on the wall decreasing when the foot of the ladder is 4 m away from the wall?

11. A particle moves along the curve Find the points on the curve at which the coordinate is changing 8 times as fast as the coordinate.

12. The radius of an air bubble is increasing at the rate of cm/s. At what rate is the volume of the bubble increasing when the radius is 1 cm?

13. A balloon which always remains spherical, has a variable diameter Find the rate of change of its volume with respect to

14. Sand is pouring from a pipe at the rate of 12 cm³/s. The falling sand forms a cone on the ground in such a way that the height of the cone is always one-sixth of the radius of the base. How fast is the height of the sand cone increasing when the height is 4cm?

15. The total cost C in rupees associated with the production of units of an item given by C Find the marginal cost when 17 units are produced.

16. The total revenue in rupees received from the sale of units of a product is given by

17. The rate of change of the area of a circle with respect to its radius at =6 cm is:

NCERT Solutions class 12 Maths Exercise 6.1

NCERT Solutions class 12 Maths Exercise 6.1

NCERT Solutions for Mathematics Class 3rd to 12th

CBSE App for Class 12

Test Generator

myCBSEguide

Related Posts

4 thoughts on “NCERT Solutions class 12 Maths Exercise 6.1”

Leave a Comment

myCBSEguide App

NCERT Solutions for Class 12 Maths Application of Derivatives

NCERT Solutions class 12 Maths Exercise 6.1

2. The volume of a cube is increasing at the rate of 8 cm3/sec. How fast is the surface area increasing when the length of an edge is 12 cm?

NCERT Solutions class 12 Maths Exercise 6.1

3. The radius of the circle is increasing uniformly at the rate of 3 cm per second. Find the rate at which the area of the circle is increasing when the radius is 10 cm.

NCERT Solutions class 12 Maths Exercise 6.1

4. An edge of a variable cube is increasing at the rate of 3 cm per second. How fast is the volume of the cube increasing when the edge if 10 cm long?

NCERT Solutions class 12 Maths Exercise 6.1

5. A stone is dropped into a quite lake and waves move in circles at the rate of 5 cm/sec. At the instant when radius of the circular wave is 8 cm, how fast is the enclosed area increasing?

NCERT Solutions class 12 Maths Exercise 6.1

6. The radius of a circle is increasing at the rate of 0.7 cm/s. What is the rate of its circumference?

7. The length of a rectangle is decreasing at the rate of 5 cm/minute. When = 8 cm and = 6 cm, find the rates of change of (a) the perimeter and (b) the area of the rectangle.

8. A balloon, which always remains spherical on inflation, is being inflated by pumping in 900 cubic centimeters of gas per second. Find the rate at which the radius of the balloon increases when the radius is 15 cm.

9. A balloon, which always remains spherical has a variables radius. Find the rate at which its volume is increasing with the radius when the later is 10 cm.

10. A ladder 5 cm long is leaning against a wall. The bottom of the ladder is pulled along the ground, away from the wall, at the rate of 2 cm/s. How fast is its height on the wall decreasing when the foot of the ladder is 4 m away from the wall?

11. A particle moves along the curve Find the points on the curve at which the coordinate is changing 8 times as fast as the coordinate.

12. The radius of an air bubble is increasing at the rate of cm/s. At what rate is the volume of the bubble increasing when the radius is 1 cm?

13. A balloon which always remains spherical, has a variable diameter Find the rate of change of its volume with respect to

14. Sand is pouring from a pipe at the rate of 12 cm3/s. The falling sand forms a cone on the ground in such a way that the height of the cone is always one-sixth of the radius of the base. How fast is the height of the sand cone increasing when the height is 4cm?

15. The total cost C in rupees associated with the production of units of an item given by C Find the marginal cost when 17 units are produced.

16. The total revenue in rupees received from the sale of units of a product is given by

17. The rate of change of the area of a circle with respect to its radius at =6 cm is:

NCERT Solutions class 12 Maths Exercise 6.1

NCERT Solutions class 12 Maths Exercise 6.1

NCERT Solutions for Mathematics Class 3rd to 12th

CBSE App for Class 12

Test Generator

myCBSEguide

Related Posts

4 thoughts on “NCERT Solutions class 12 Maths Exercise 6.1”

Leave a Comment

2. The volume of a cube is increasing at the rate of 8 cm³/sec. How fast is the surface area increasing when the length of an edge is 12 cm?

14. Sand is pouring from a pipe at the rate of 12 cm³/s. The falling sand forms a cone on the ground in such a way that the height of the cone is always one-sixth of the radius of the base. How fast is the height of the sand cone increasing when the height is 4cm?