NCERT Solutions class 12 Maths Exercise 9.4

NCERT Solutions class 12 Maths Exercise 9.4 Class 12 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.

Download NCERT solutions for Differential Equations  as PDF

NCERT Solutions class 12 Maths Differential Equations

For each of the differential equations in Questions 1 to 4, find the general solution:

1.  

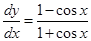
Ans. Given: Differential equation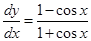

  

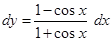
Integrating both sides,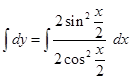

 

  

 

  

---

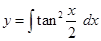
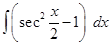
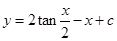
2.  

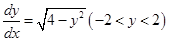
Ans. Given: Differential equation   

 

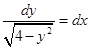
Integrating both sides,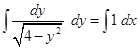

  

 

 

---

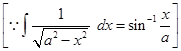
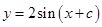
3.  

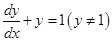
Ans. Given: Differential equation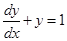   

      

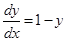
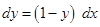
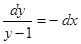
Integrating both sides,

 

  

 

 

 

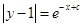
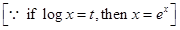
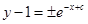
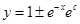
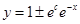
 , where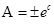

---

4.  

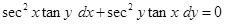
Ans. Given: Differential equation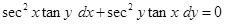

Dividing by  we have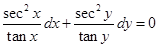

  

    

  

---

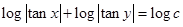
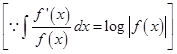
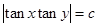
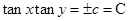
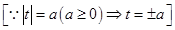
5.  

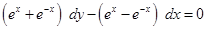
Ans. Given: Differential equation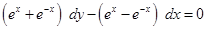

 

 

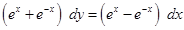
Integrating both sides,

   

---

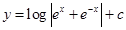
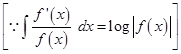
6.  

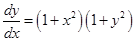
Ans. Given: Differential equation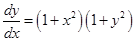

 

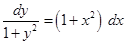   [Separating variables]

Integrating both sides,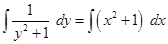

 

---

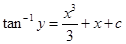
7.  

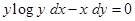
Ans. Given: Differential equation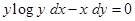   

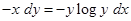
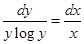  [Separating variables]

Integrating both sides,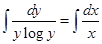

Putting 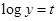 on L.H.S., we get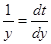  

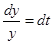
Now eq. (i) becomes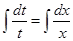

 

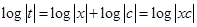
[If all the terms in the solution of a differential equation involve log, it is better to use 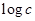 or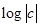 instead of  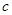in the solution.]

   

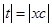
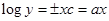  where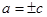

 

---

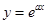
8.  

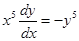
Ans. Given: Differential equation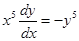

 

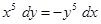
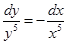   [Separating variables]

 

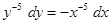
Integrating both sides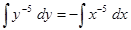

 

 

 

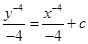
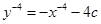
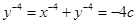
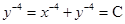 , where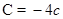

---

9.  

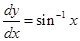
Ans. Given: Differential equation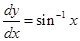

 

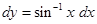
Integrating both sides,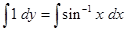

 

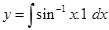
Applying product rule,           I          II

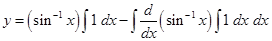
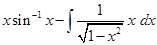=   ……….(i)

To evaluate

Putting, 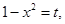 differentiate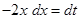

  =

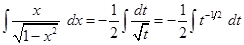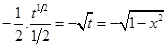
Putting this value in eq. (i), the required general solution is

---

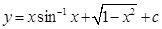
10.  

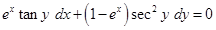
Ans. Given: Differential equation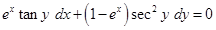

Dividing each term by 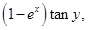 we get

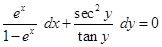  [Separating variables]

Integrating both sides,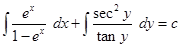

 

 

 

 

  

---

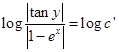
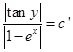
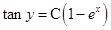
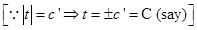
For each of the differential equations in Question 11 to 12, find a particular solution satisfying the given condition:

11. 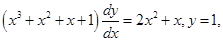 when  

Ans. Given: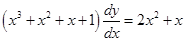

  

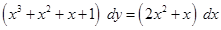
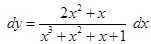 [Separating variables]

 

 

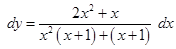
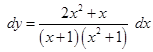
Integrating both sides,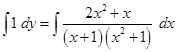

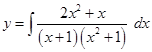   …(i)

Let 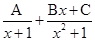  [Partial fraction] ……….(ii)

 

Comparing the coefficients of 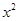 on both sides, A + B = 2 ……….(iii)

Comparing the coefficients of 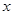 on both sides, B + C = 1 ……….(iv)

Comparing constants on both sides, A + C = 0 ……….(v)

From eq. (iii) – (iv), we haveA – C = 1 ……….(vi)

Adding eq. (v) and (vi), we have 2A = 1

 A =

From eq. (v), we have C = – A =

Putting the value of C in eq. (iv),  B –  = 1

 B = 1 +  =

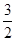
Putting the values of A, B, and C in eq. (ii), we have

=

=

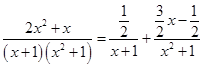
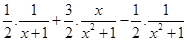
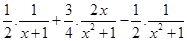
Putting this value in eq. (i),

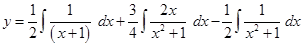
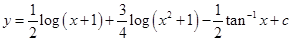 ……….(vii)

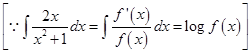
Now, when  , putting these values in eq. (vii),

  

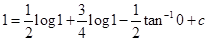
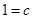
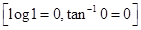
Putting value of 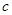 in eq. (vii), the required general solution is

 

 

 

---

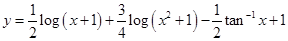
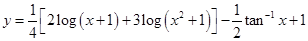
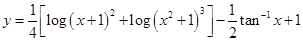
12. 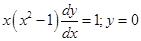 when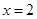  

For each of the differential equations in Question 13 to 14, find a particular solution satisfying the given condition.

Ans. Given: Differential equation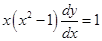

  

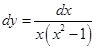
Integrating both sides,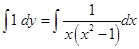

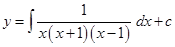   ……….(i)

Let 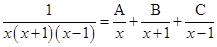 ……….(ii)

 

 

 

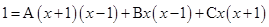
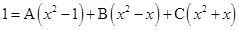
Comparing the coefficients of 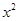 on both sides, A + B + C = 0 ……….(iii)

Comparing the coefficients of 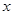 on both sides, 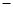B + C = 0

   C = B ……….(iv)

Comparing constants on both sides, 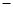A = 1

 A = 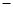1 ……….(v)

Putting A = 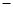1 and C = B in eq. (iii),

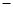1 + B + B = 0  2B = 1 B =

From eq. (iv),C = B =

Putting the values of A, B and C in eq. (ii), we get

 

 

 

 

 

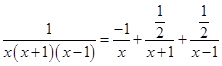
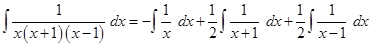
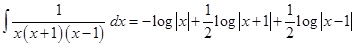
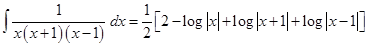
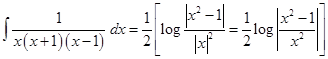
Putting this value in eq. (i),

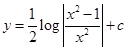 ……….(v)

Now, putting 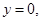 when 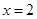 in eq. (v), we get

    

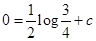
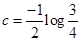
Putting the value of 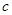 in eq. (v), the required general solution is

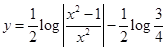
[NOTE: You can also do, to evaluate 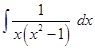 =  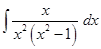=  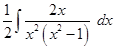Put  ]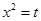

---

13. 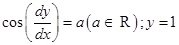 when  

Ans. Given: Differential equatio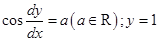n when

 

 

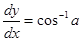
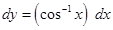
Integrating both sides,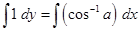

 

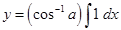
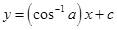  ……….(i)

Now putting 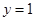 when  in eq. (i), we get

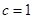
Putting 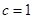 in eq. (i),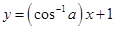

 

 

---

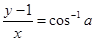
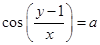
14.  when

Ans. Given: Differential equation   

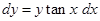
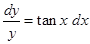  [Separating variables]

Integrating both sides,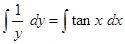

 

 

 

 

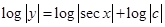
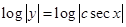
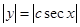
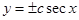
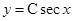   where 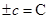 ……….(i)

Now putting 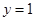 and  in eq. (i), we get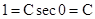

Putting C = 1 in eq. (i), we get the required general solution

 

---

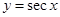
NCERT Solutions class 12 Maths Exercise 9.4

15. Find the equation of the curve passing through the point (0, 0) and whose differential equation is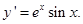  

Ans. Given: Differential equation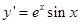

 

 

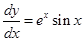
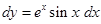
Integrating both sides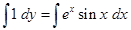

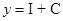   ………(i)

where I = 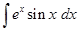  ……….(ii)

Applying product rule,

 

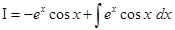
Again applying product rule,

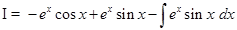
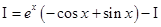  [By eq. (ii)]

 2I =

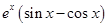
 I =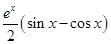

Putting this value of I in eq. (i), we get

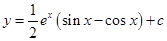  ……….(iii)

Now putting  and  in eq. (iii)

 

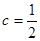
Putting the value of 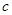 in eq. (iii), we get the required general solution

 

 

---

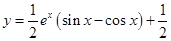
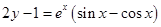
NCERT Solutions class 12 Maths Exercise 9.4

16. For the differential equation 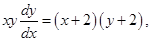 find the solution curve passing through the point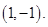  

Ans. Given: Differential equation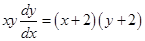

 

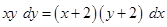
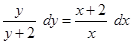  [Separating both sides]

Integrating both sides

 

 

 

 

 

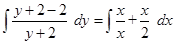
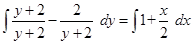
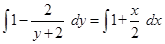
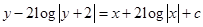
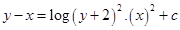  ……….(i)

Now putting 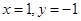 in eq. (i),

 

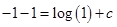
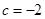
Putting this value of 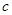 in eq. (i) to get the required solution curve

 

---

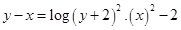
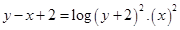
NCERT Solutions class 12 Maths Exercise 9.4

17. Find the equation of the curve passing through the point 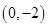 given that at any point  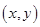on the curve the product of the slope of its tangent and 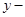coordinate of the point is equal to the 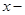coordinate of the point.

Ans. Let P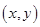 be any point on the required curve.

According to the question, Slope of the tangent to the curve at P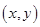 

    

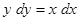
Integrating both sides,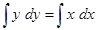

 

 

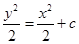
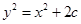
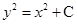  where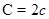

Now it is given that curve 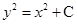 passes through the point .

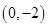Therefore, putting  and 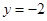 in this equation, we get C = 4

Putting the value of C in the equation ,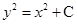

 

---

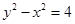
NCERT Solutions class 12 Maths Exercise 9.4

18. At any point 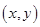 of a curve the slope of the tangent is twice the slope of the line segment joining the point of contact to the point  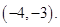Find the equation of the curve given that it passes through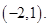  

Ans. According to the question, slope of the tangent at any point P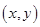 of the required curve

= 2. Slope of the line joining the point of contact P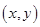 to the given point A

 

 

 

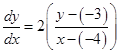
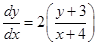
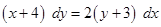
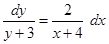   [Separating variables]

Integrating both side,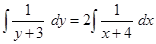

 

 

 

 

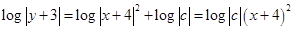
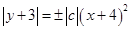
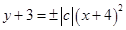
  where 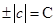  ……….(i)

Now it is given that curve (i) passes through the point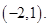

Therefore, putting  and 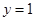 in eq. (i),

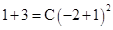
 4 = 4C C = 1

Putting C = 1 in eq. (i), we get the required solution,

 

---

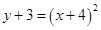
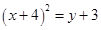
NCERT Solutions class 12 Maths Exercise 9.4

19. The volume of the spherical balloon being inflated changes at a constant rate. If initially its radius is 3 units and after 3 seconds it is 6 units. Find the radius of balloon after 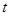 seconds.

Ans. Let 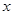 be the radius of the spherical balloon at time

Given: Rate of change of volume of spherical balloon is constant =  (say)

 

 

 

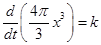
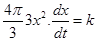
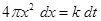   [Separating variables]

Integrating both sides,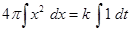

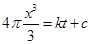   ……….(i)

Now it is given that initially radius is 3 units, when

Therefore, putting 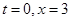 in eq. (i),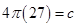

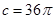   ……….(ii)

Again when  sec, then 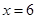 units

Therefore, putting  and 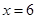 in eq. (i),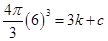

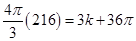  [From eq. (ii)]

 

    

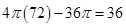
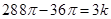
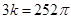
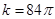   ……….(iii)

Putting the value of 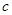 and  in eq. (i), we get

 

 

 

---

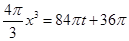
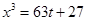
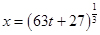
NCERT Solutions class 12 Maths Exercise 9.4

20. In a bank principal increases at the rate of 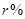 per year. Find the value of  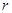is ` 100 double itself in 10 years.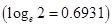  

Ans. Let P be the principal (amount) at the end of 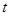 years.

According to the given condition, rate of increase of principal per year = 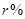 (of principal)

 

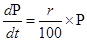
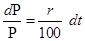  [Separating variables]

Integrating both sides,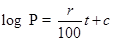  ……….(i)

 [Since P being principal > 0, hence  ]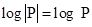

Now initial principal = ` 100 (given), i.e., when 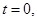 then P = 100

Therefore, putting 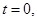 P = 100 in eq. (i),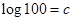

Putting 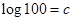 in eq. (i),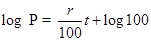

 

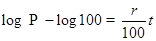
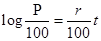   ……….(ii)

Now putting P = double of itself = 2 x 100 = ` 200, when 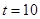 years (given)

 

 

 

---

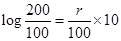
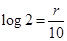
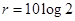
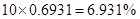
NCERT Solutions class 12 Maths Exercise 9.4

21. In a bank principal increases at the rate of 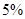 per year. An amount of ` 1000 is deposited with this bank, how much will it worth after 10 years?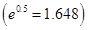  

Ans. Let P be the principal (amount) at the end of 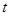 years.

According to the given condition, rate of increase of principal per year = 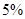 (of principal)

 

 

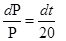  [Separating variables]

Integrating both sides,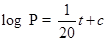  ……….(i)

 [Since P being principal > 0, hence  ]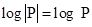

Now initial principal = ` 1000 (given), i.e., when 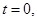 then P = 1000

Therefore, putting 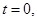 P = 1000 in eq. (i),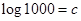

Putting 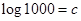 in eq. (i),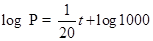

 

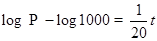
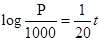   ……….(ii)

Now putting 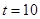 years (given)

  

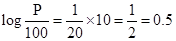
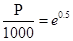
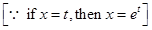
 P = 1000 x 1.648 = ` 1648

---

NCERT Solutions class 12 Maths Exercise 9.4

22. In a culture the bacteria count is 1,00,000. The number is increased by 10% in 2 hours. In how many hours will the count reach 2,00,000, if the rate of growth of bacteria is proportional to the number present.

Ans. Let 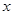 be the bacteria present in the culture at time  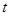hours.

According to the question,

Rate of growth of bacteria is proportional to the number present

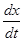  is proportional to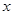

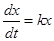  where 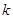 is the constant of proportionality

   

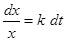
Integrating both sides,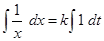

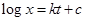  ……….(i)

Now it is given that initially the bacteria count is 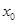 (say) = 1,00,000

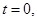 when  then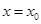

Putting these values in eq. (i)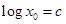

Putting 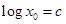  in eq. (i), we get

 

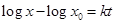
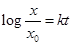  ……….(ii)

Now it is given also that the number of bacteria increased by 10% in 2 hours.

Therefore, increase in bacteria in 2 hours =  = 10,000

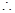
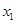   the amount of bacteria at  = 1,00,000 + 10,000 = 1,10,000 =  (say)

Putting 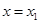 and  in eq. (ii),

 

 

 

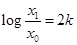
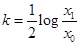
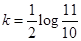
Putting this value of 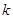 in eq. (ii), we get,

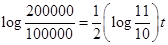  [when ]

 

 

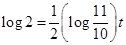
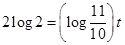
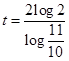  hours

---

NCERT Solutions class 12 Maths Exercise 9.4

23. The general solution of the differential equation 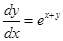 is:

(A)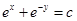  

(B)  

(C)  

(D)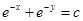

Ans. Given: Differential equation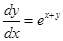  

  

 

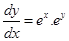
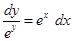   [Separating variables]

 

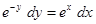
Integrating both sides,

 

 

  

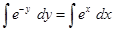
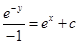
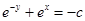
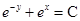   where  which is required solution

Therefore, option (A) is correct.

NCERT Solutions class 12 Maths Exercise 9.4

NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes text book solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 20 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide

CBSE App for Students

To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.