NCERT Solutions class 12 Maths Exercise 11.2 Class 12 Maths book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 12 Maths chapter wise NCERT solution for Maths part 1 and Maths part 2 for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
Download NCERT solutions for Three Dimensional Geometry as PDF.

NCERT Solutions class 12 Maths Three Dimensional Geometry
.Show that the three lines with direction cosines 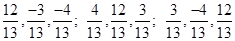 are mutually perpendicular.
are mutually perpendicular.
Ans. Given: Direction cosines of three lines are
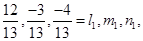

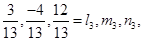
For first two lines,
= 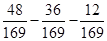 =
= 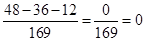
Therefore, the first two lines are perpendicular to each other.
For second and third lines,
= 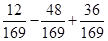 =
= 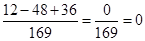
Therefore, second and third lines are also perpendicular to each other.
For First and third lines,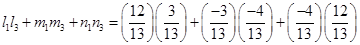
=  =
= 
Therefore, first and third lines are also perpendicular to each other.
Hence, given three lines are mutually perpendicular to each other.
2.Show that the line through the points 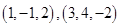 is perpendicular to the line through the points (0, 3, 2) and (3, 5, 6).
is perpendicular to the line through the points (0, 3, 2) and (3, 5, 6).
Ans. We know that direction ratios of the line joining the points A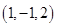 and B
and B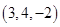 are
are 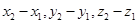

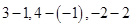


Again, direction ratios of the line joining the points C (0, 3, 2) and D (3, 5, 6) are 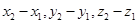

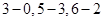

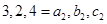 (say)
(say)
For lines AB and CD,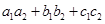 =
= 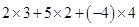 = 6 + 10 – 16 = 0
= 6 + 10 – 16 = 0
Therefore, line AB is perpendicular to line CD.
3.Show that the line through points (4, 7, 8), (2, 3, 4) is parallel to the line through the points 
Ans. We know that direction ratios of the line joining the points A (4, 7, 8) and B (2, 3, 4) are 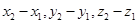



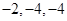 =
= 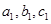 (say)
(say)
Again direction ratios of the line joining the points C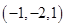 and D (1, 2, 5) are
and D (1, 2, 5) are 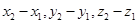



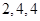 =
= 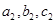 (say)
(say)
For the lines AB and CD,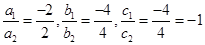
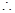
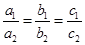
Therefore, line AB is parallel to line CD.
4.Find the equation of the line which passes through the point (1, 2, 3) and is parallel to the vector 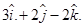
Ans. A point on the required line is A (1, 2, 3) = 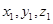
 Position vector of a point on the required line is
Position vector of a point on the required line is 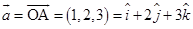
The required line is parallel to the vector 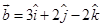
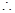 direction ratios of the required line are coefficient of
direction ratios of the required line are coefficient of 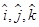 in
in 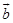 are
are

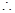 Vector equation of the required line is
Vector equation of the required line is
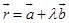

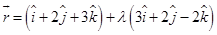
Where 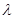 is a real number.
is a real number.
Cartesian equation of this equation is 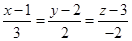
5.Find the equation of the line in vector and in Cartesian form that passes through the point with position vector 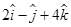 and is in the direction
and is in the direction 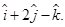
Ans. Position vector of a point on the required line is  =
= 
The required line is in the direction of the vector is 
 Direction ratios of required line are coefficients of
Direction ratios of required line are coefficients of 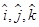 in
in 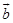 =
= 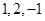
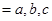
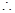 Equation of the required line in vector form is
Equation of the required line in vector form is 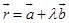

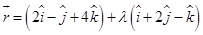
Where 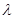 is a real number.
is a real number.
Cartesian equation of this equation is 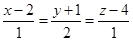
6.Find the Cartesian equation of the line which passes through the point 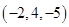 and parallel to the line given by
and parallel to the line given by 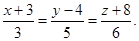
Ans. Given: A point on the line is 

Equation of the given line in Cartesian form is 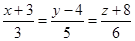
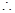 Direction ratios of the given line are its denominators 3, 5, 6
Direction ratios of the given line are its denominators 3, 5, 6 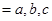
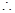 Equation of the required line is
Equation of the required line is 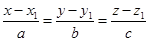

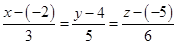 =
= 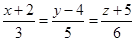
7.The Cartesian equation of a line is 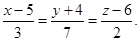 Write its vector form.
Write its vector form.
Ans. Given: The Cartesian equation of the line is 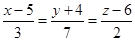 =
= 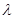 (say)
(say)

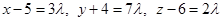

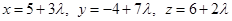
General equation for the required line is 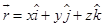
Putting the values of 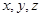 in this equation,
in this equation,
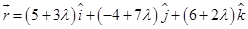 =
= 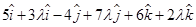



8.Find the vector and Cartesian equations of the line that passes through the origin and 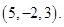
Ans. 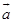 = Position vector of a point here O (say) on the line = (0, 0, 0) =
= Position vector of a point here O (say) on the line = (0, 0, 0) = 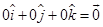
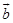 = A vector along the line
= A vector along the line
= 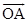 = Position vector of point A – Position vector of point O
= Position vector of point A – Position vector of point O
= 
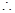 Vector equation of the line is
Vector equation of the line is 

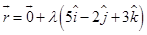

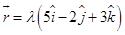
NowCartesian equation of the line
Direction ratios of line OA are 
And a point on the line is O (0, 0, 0) = 
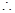 Cartesian equation of the line =
Cartesian equation of the line = 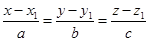
= 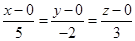 =
= 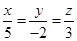
Remark:In the solution of the above question we can also take:
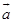 = Position vector of point A =
= Position vector of point A = 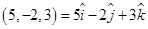 for vector form and point A as
for vector form and point A as  =
= 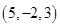 for Cartesian form.
for Cartesian form.
Then the equation of the line in vector form is 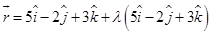
And equation of line in Cartesian form is 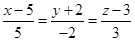
9.Find vector and Cartesian equations of the line that passes through the points 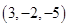 and
and 
Ans. Let 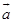 and
and 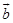 be the position vectors of the points A
be the position vectors of the points A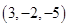 and B
and B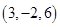 respectively.
respectively.
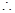
 and
and 
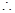 A vector along the line =
A vector along the line = 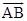 = Position vector of point B – Position vector of point A
= Position vector of point B – Position vector of point A

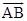 =
= 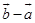 =
= 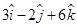
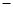
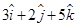 =
= 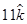
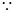 Vector equation of the line is
Vector equation of the line is 
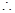
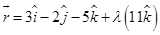
And another vector equation for the same line is 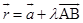 =
= 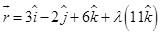
Cartesian equation
Direction ratios of line AB are 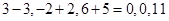
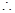 Equation of the line is
Equation of the line is 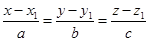 =
= 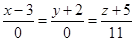
10.Find the angle between the following pairs of lines:
(i)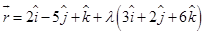 and
and 
(ii)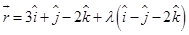 and
and 
Ans. (i)Equation of the first line is 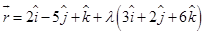
Comparing with  ,
,
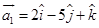 and
and 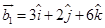
(vector 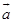 is the position vector of a point on line and
is the position vector of a point on line and 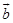 is a vector along the line)
is a vector along the line)
Again, equation of the second line is 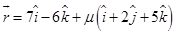
Comparing with  ,
,
 and
and 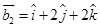
(vector 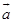 is the position vector of a point on line and
is the position vector of a point on line and 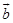 is a vector along the line)
is a vector along the line)
Let  be the angle between these two lines, then
be the angle between these two lines, then
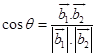 =
= 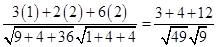 =
= 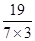
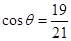

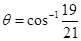
(ii)Comparing the first and second equations with  and
and  resp.
resp.
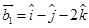 and
and 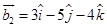
Let  be the angle between these two lines, then
be the angle between these two lines, then
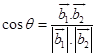 =
= 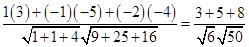 =
= 
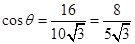

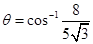
NCERT Solutions class 12 Maths Exercise 11.2
11.Find the angle between the following pair of lines:
(i)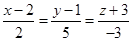 and
and 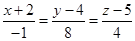
(ii) and
and 
Ans. (i)Given: Equation of first line is 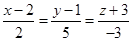
The direction ratios of this line i.e., a vector along the line is
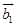 =
= 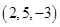 =
= 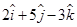
Nowequation of second line is 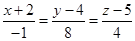
The direction ratios of this line i.e., a vector along the line is
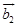 =
= 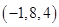 =
= 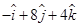
Let  be the angle between these two lines, then
be the angle between these two lines, then
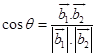 =
= 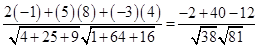 =
= 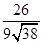

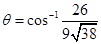
(ii)Given: Equation of first line is 
The direction ratios of this line i.e., a vector along the line is
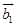 =
= 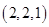 =
= 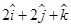
Nowequation of second line is 
The direction ratios of this line i.e., a vector along the line is
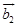 =
= 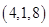 =
= 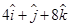
Let  be the angle between these two lines, then
be the angle between these two lines, then
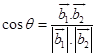 =
= 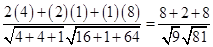 =
= 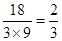

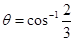
NCERT Solutions class 12 Maths Exercise 11.2
12.Find the values of 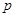 so that the lines
so that the lines  and
and 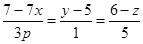 are at right angles.
are at right angles.
Ans. Given: Equation of one line 

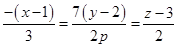

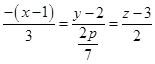
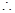 Direction ratios of this line are
Direction ratios of this line are 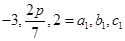
Again, equation of another line 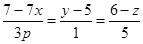

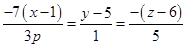

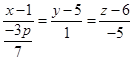
 Direction ratios of this line are
Direction ratios of this line are 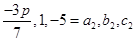
Since, these two lines are perpendicular.
Therefore,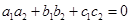

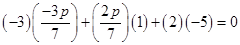

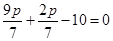

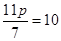

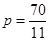
NCERT Solutions class 12 Maths Exercise 11.2
13.Show that the lines 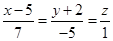 and
and 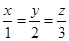 are perpendicular to each other.
are perpendicular to each other.
Ans. Equation of one line 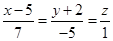
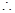 Direction ratios of this line are
Direction ratios of this line are  =
= 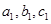


Again equation of another line 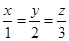
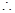 Direction ratios of this line are 1, 2, 3 =
Direction ratios of this line are 1, 2, 3 = 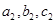

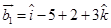
Now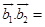
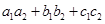 =
= 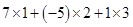 =
= 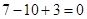
Hence, the given two lines are perpendicular to each other.
NCERT Solutions class 12 Maths Exercise 11.2
14.Find the shortest distance between the lines 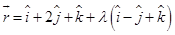 and
and 
Ans. Comparing the given equations with 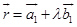 and
and  , we get
, we get
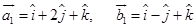 and
and 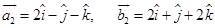
Since, the shortest distance between the two skew lines is given by
 ……….(i)
……….(i)
Here,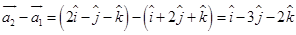
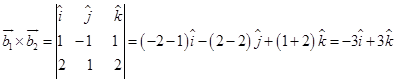
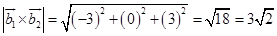

Putting these values in eq. (i),
Shortest distance 
NCERT Solutions class 12 Maths Exercise 11.2
15.Find the shortest distance between the lines 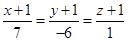 and
and  .
.
Ans. Equation of one line is 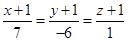
Comparing this equation with 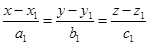 , we have
, we have
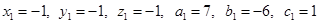
Again equation of another line is 
Comparing this equation with 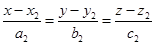 , we have
, we have
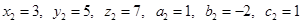
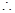
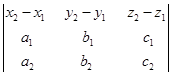 =
= 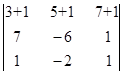
Expanding by first row = 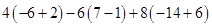 =
= 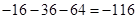
And
=  =
= 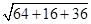 =
= 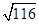
 Length of shortest distance =
Length of shortest distance = 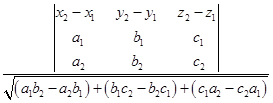
=  (numerically)
(numerically)
= 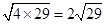
NCERT Solutions class 12 Maths Exercise 11.2
16.Find the shortest distance between the lines whose vector equations are
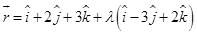 and
and 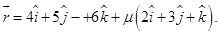
Ans. Equation of the first line is 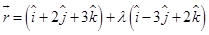
Comparing this equation with 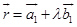 ,
,
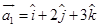 and
and 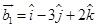
Again equation of second line 
Comparing this equation with 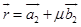 ,
,
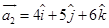 and
and 
Now shortest distance  =
= 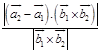 ……….(i)
……….(i)
Here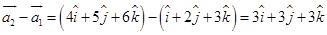
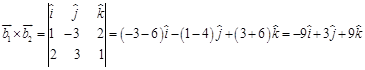
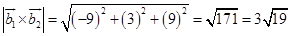
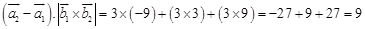
Putting these values in eq. (i),
Shortest distance 
NCERT Solutions class 12 Maths Exercise 11.2
17.Find the shortest distance between the lines whose vector equations are
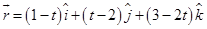 and
and 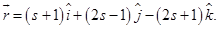
Ans. Equation of first line is 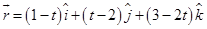
=  =
= 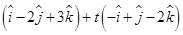
Comparing this equation with 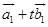 ,
,
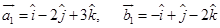
Equation of second line is 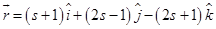
= 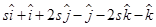 =
= 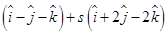
Comparing this equation with 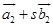 ,
,
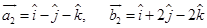
Now Shortest distance  =
=  ……….(i)
……….(i)
Here
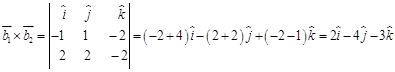
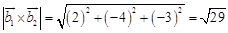
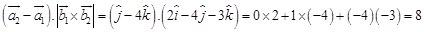
Putting these values in eq. (i),
Shortest distance 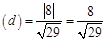
NCERT Solutions class 12 Maths Exercise 11.2
NCERT Solutions Class 12 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 12 Maths includes text book solutions from both part 1 and part 2. NCERT Solutions for CBSE Class 12 Maths have total 13 chapters. 12 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 12 solutions PDF and Maths ncert class 12 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide
CBSE App for Students
To download NCERT Solutions for class 12 Physics, Chemistry, Biology, History, Political Science, Economics, Geography, Computer Science, Home Science, Accountancy, Business Studies and Home Science; do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now