Miscellaneous Exercise
1. Evaluate: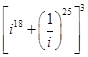 
Ans. Given: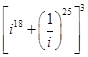
= 
= 
= 
= 
= 
= 
= 
= 
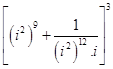
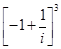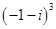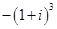
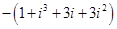
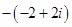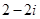
2. For any two complex numbers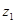 
and 
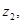prove that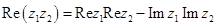 
Ans. Let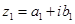 
and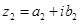 
Then 
and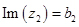 
Now, 
= 
= 
= 
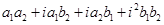
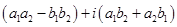
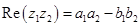
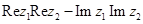
3. Reduce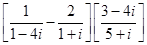 
to the standard form.
Ans. Here: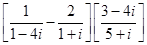
= 
= 
= 
= 
= 
= 
= 
= 
= 
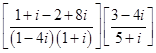
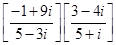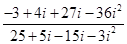
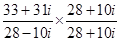
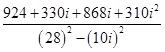
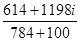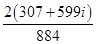
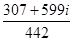
4. If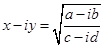 
prove that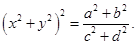 
Ans. Given: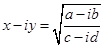
Squaring both sides, we get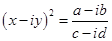
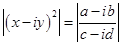
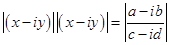
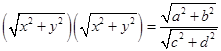
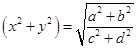
Squaring both sides, we get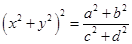 
5. Convert the following in the polar form:
(i) 
(ii)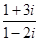 
Ans. (i) Here = 
= 
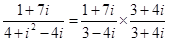
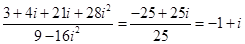
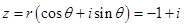
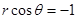and 
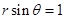
Squaring both sides and adding both the equations, we get
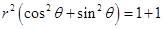
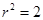
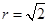
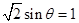
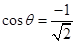and 
and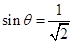 
[
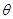lies in second quadrant]
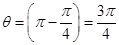
Therefore, Polar form of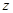 
is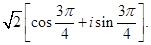 
(ii) Here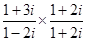 
= 
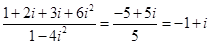
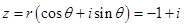
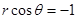and
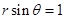
Squaring both sides and adding both the equations, we get
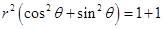
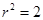
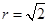
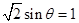
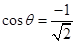and
and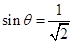
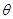[ lies in second quadrant]
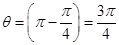
Therefore, Polar form of 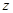is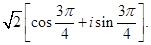
Solve each of the equations in exercises 6 to 9:
6. 
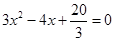
Ans. Given: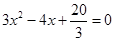
Comparing with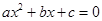 
, 
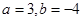and 
= 
= 
= 
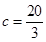
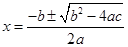
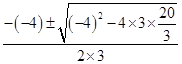
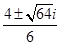
Therefore,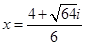 
and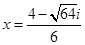 
7. 
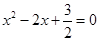
Ans. Given: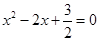
Comparing with ,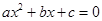 
and 
= 
= 
= 
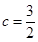
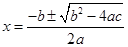
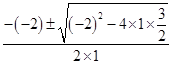
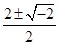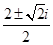
Therefore,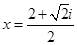 
and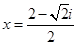 
8. 
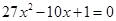
Ans. Given: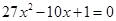
Comparing with ,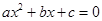 
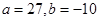and 
= 
= 
= 
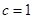
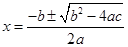
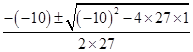
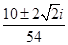
Therefore,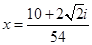 
and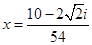 
9. 
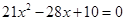
Ans. Given: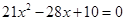
Comparing with ,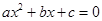 
and 
= 
= 
= 
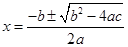
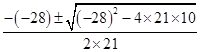
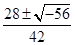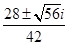
Therefore,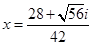 
and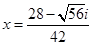 
10. If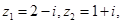 
find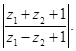 
Ans. Here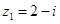 
and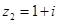 
= 
= 
= 
= 
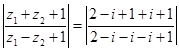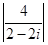
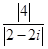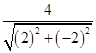
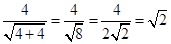
11. If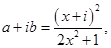 
prove that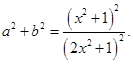 
Ans. Here 
= 
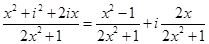
Comparing both sides, we have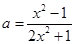 
and 
= 
= 
= 
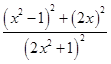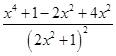
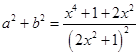
Proved.
12. Let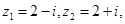 
find:
(i)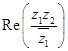 
(ii)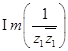 
Ans. Here 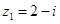and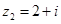 
(i)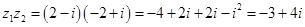 
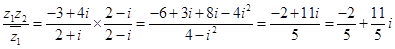
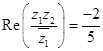
(ii)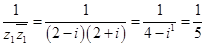 
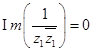
13. Find the modulus and argument of the complex number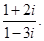 
Ans. Let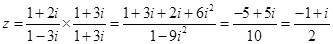 
= 
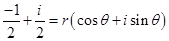
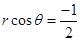and 
Squaring both sides and adding both the equations, we get
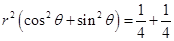
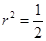
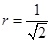
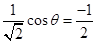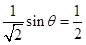
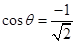and 
and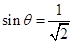
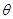[ lies in second quadrant]
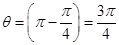
Therefore,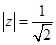 
and arg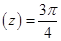
14. Find the real numbers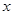 
and 
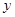if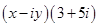 
is the conjugate of 
Ans. Here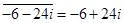 
Now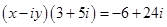 
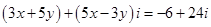
Comparing both sides, we have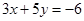 
and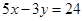 
Solving both equations, we have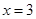 
and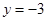 
15. Find the modulus of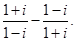 
Ans. Here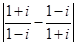 
= 
= 
= 
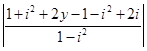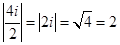
16. If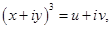 
then show that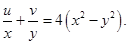 
Ans. Given: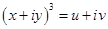 
Comparing both sides, we have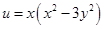 
and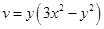 
Now,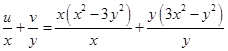 
= 
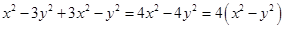
17. If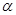 
and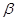 
are different complex numbers with 
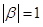then find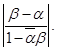 
Ans. Here 
= 
= 
= 
= 
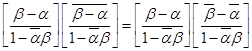
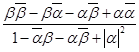
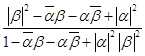
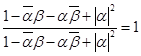
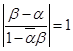
18. Find the number of non-zero integral solutions of the equation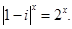 
Ans. Here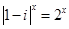 
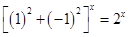
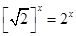
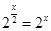
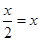
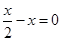
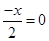
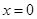
19. If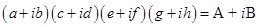 
then show that:
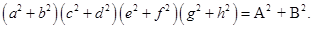
Ans. Given: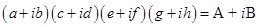
Taking modulus on both sides,
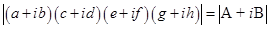
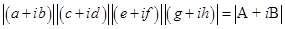
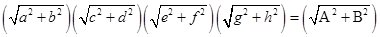
Squaring both sides, we get
20. If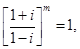 
then find the least positive integral value of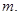 
Ans. Given: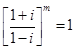 
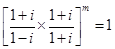
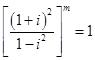
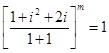
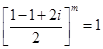
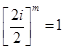
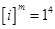
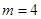
Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
Learn8 App
Practice unlimited questions for Entrance tests & government job exams at ₹99 only
Install Now